What Is 20 Percent Of 46
listenit
May 23, 2025 · 5 min read
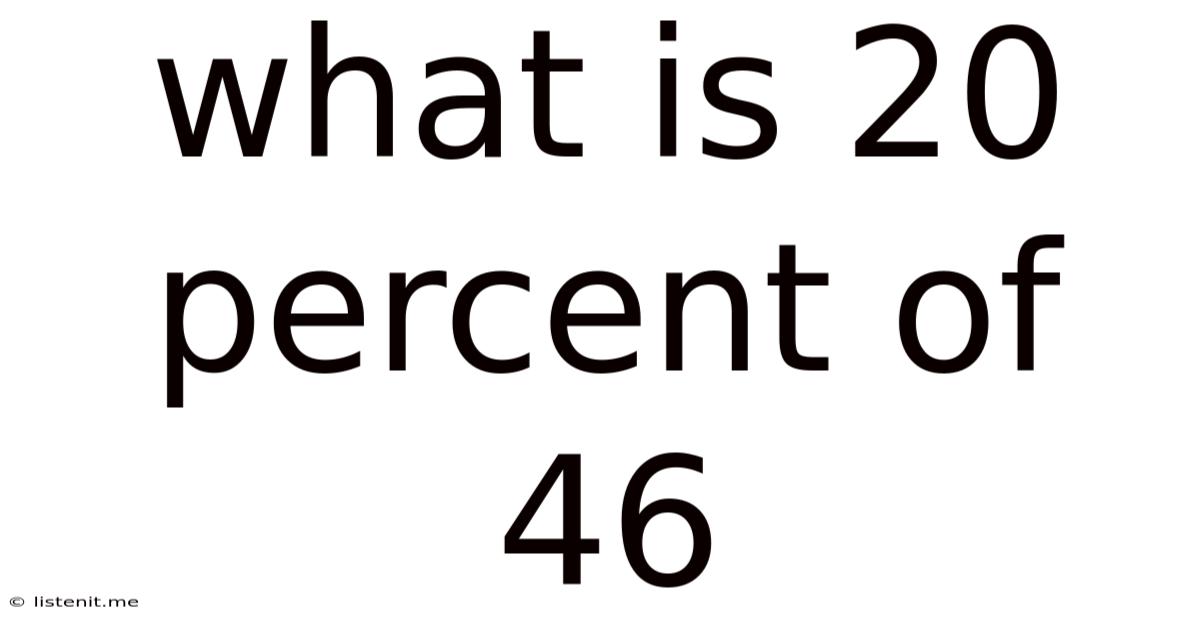
Table of Contents
What is 20 Percent of 46? A Deep Dive into Percentages and Their Applications
Finding 20 percent of 46 might seem like a simple arithmetic problem, easily solvable with a calculator. However, understanding the underlying concepts of percentages and their widespread applications is crucial for various aspects of life, from personal finance to business analysis. This article will delve into the calculation, explore the methodology behind percentage calculations, and illustrate the importance of percentages in real-world scenarios.
Calculating 20% of 46: The Basics
The most straightforward method to calculate 20% of 46 is to convert the percentage into a decimal and multiply it by the number. 20% can be written as 0.20 (or simply 0.2). Therefore:
0.20 x 46 = 9.2
Therefore, 20% of 46 is 9.2.
This simple calculation is fundamental to many more complex percentage-based problems. Let's explore different ways to arrive at the same answer and expand on the underlying mathematical principles.
Alternative Methods for Calculating Percentages
While the decimal method is the most efficient, understanding alternative approaches enhances comprehension and problem-solving skills.
Method 1: Fraction Method
Percentages are essentially fractions with a denominator of 100. 20% can be expressed as the fraction 20/100, which simplifies to 1/5. Therefore, finding 20% of 46 is equivalent to finding one-fifth of 46:
46 / 5 = 9.2
Method 2: Proportion Method
This method uses the concept of ratios and proportions. We can set up a proportion:
20/100 = x/46
where 'x' represents 20% of 46. Cross-multiplying and solving for 'x':
100x = 20 * 46 100x = 920 x = 9.2
Understanding the Concept of Percentages
A percentage is a way of expressing a number as a fraction of 100. It represents a proportion or rate per hundred. The percentage symbol (%) is used to denote a percentage. Understanding percentages is crucial for interpreting data, making comparisons, and solving various problems in diverse fields.
Real-World Applications of Percentage Calculations
Percentage calculations are used extensively in many aspects of daily life and professional contexts:
1. Finance:
- Interest Rates: Banks and financial institutions use percentages to calculate interest on loans, savings accounts, and investments. Understanding interest rates is vital for making informed financial decisions. For example, calculating the interest accrued on a loan or the return on an investment involves percentage calculations.
- Discounts and Sales Tax: Stores frequently offer discounts expressed as percentages. Calculating the final price after a discount requires understanding percentage reductions. Similarly, sales tax is calculated as a percentage of the purchase price.
- Profit Margins: Businesses use percentages to calculate profit margins, which represent the percentage of revenue that is profit after deducting expenses. Analyzing profit margins is crucial for assessing the financial health of a business.
- Investment Returns: Investors track the performance of their investments using percentages. Calculating the percentage return on an investment helps in evaluating its success.
2. Science and Statistics:
- Data Analysis: Percentages are fundamental to data analysis and interpretation. Representing data as percentages allows for easy comparison and visualization. For example, expressing survey results as percentages facilitates understanding the distribution of responses.
- Probability and Statistics: Percentages are frequently used to represent probabilities and statistical measures. For instance, expressing the probability of an event occurring as a percentage provides a clear and easily understandable measure of likelihood.
3. Everyday Life:
- Tip Calculation: Calculating a tip in a restaurant often involves determining a percentage of the total bill.
- Recipe Adjustments: When adjusting recipes, percentages can be used to scale ingredients up or down.
- Grade Calculation: In education, grades are often expressed as percentages.
- Sales Commission: Sales professionals often receive commissions based on a percentage of their sales.
4. Business and Marketing:
- Market Share: Companies track their market share, which is the percentage of total market sales they control.
- Conversion Rates: In marketing, conversion rates (the percentage of website visitors who complete a desired action) are vital metrics for evaluating the effectiveness of marketing campaigns.
- Growth Rates: Businesses analyze growth rates (percentage increase in sales, profits, etc.) to assess their performance.
- Cost of Goods Sold: Determining the percentage of revenue spent on producing goods is crucial for pricing strategies.
Beyond the Basics: More Complex Percentage Problems
While calculating 20% of 46 is relatively simple, more complex scenarios involve multiple percentage calculations or require solving for an unknown variable within a percentage problem. For instance:
- Finding the original amount: If you know that 20% of a number is 9.2, you can work backward to find the original number (46). This involves dividing 9.2 by 0.2.
- Calculating percentage increase or decrease: Determining the percentage change between two values requires calculating the difference between the values, dividing by the original value, and multiplying by 100.
- Compounding percentages: In scenarios involving compound interest or multiple percentage changes, the calculations become more complex, requiring sequential percentage calculations.
Conclusion: The Enduring Importance of Percentages
Understanding percentages is an essential skill applicable across numerous fields. While calculating 20% of 46 is a basic calculation, mastering the underlying concepts empowers individuals to confidently tackle more complex percentage problems encountered in finance, science, statistics, and everyday life. Proficiency in percentage calculations enhances decision-making abilities and fosters a deeper understanding of quantitative information. From understanding financial statements to interpreting scientific data, the ability to work with percentages remains a vital skill for success in a data-driven world. The simple question, "What is 20 percent of 46?" opens the door to a much broader understanding of numerical relationships and their significance.
Latest Posts
Latest Posts
-
How Many Hours Until 6 00am Today
May 24, 2025
-
55 000 A Year Is How Much Biweekly
May 24, 2025
-
What Is The Greatest Common Factor Of 12 And 40
May 24, 2025
-
What Time Will It Be In 44 Hours
May 24, 2025
-
What Year Are 17 Year Olds Born
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 20 Percent Of 46 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.