What Is 2 3 Of 32
listenit
May 25, 2025 · 5 min read
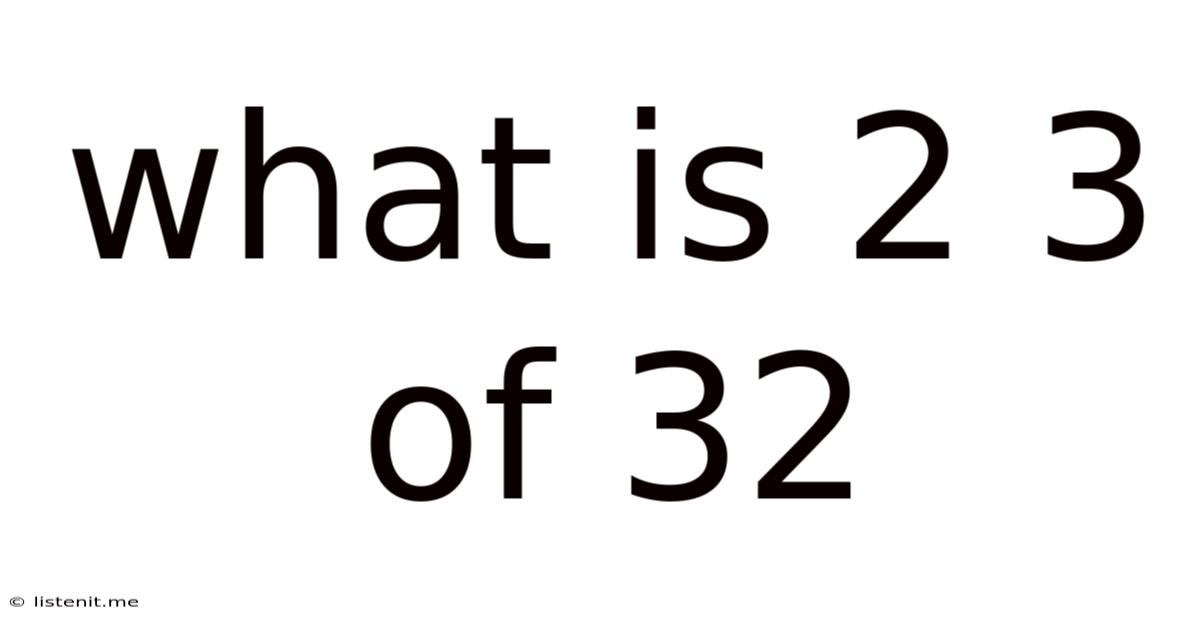
Table of Contents
What is 2/3 of 32? A Comprehensive Guide to Fractions and Their Applications
Finding a fraction of a number is a fundamental concept in mathematics with widespread applications in everyday life, from cooking and budgeting to more complex fields like engineering and finance. This article will delve deep into the calculation of 2/3 of 32, explaining the process step-by-step, exploring different methods of calculation, and highlighting the broader significance of fractional arithmetic. We'll also examine related concepts and explore real-world examples to cement your understanding.
Understanding Fractions: A Quick Refresher
Before we tackle the problem at hand, let's briefly review the essential components of a fraction:
- Numerator: The top number in a fraction (in this case, 2). It represents the number of parts we're considering.
- Denominator: The bottom number in a fraction (in this case, 3). It represents the total number of equal parts a whole is divided into.
A fraction like 2/3 indicates that we have 2 parts out of a total of 3 equal parts.
Method 1: Multiplication
The most straightforward way to find 2/3 of 32 is through multiplication. We can express "of" as multiplication in mathematical terms. Therefore, "2/3 of 32" translates to:
(2/3) * 32
To perform this calculation:
- Multiply the numerator by the whole number: 2 * 32 = 64
- Divide the result by the denominator: 64 / 3 = 21.333...
Therefore, 2/3 of 32 is 21.333... (approximately 21.33). The three dots indicate that the decimal continues infinitely. This is a recurring decimal.
Method 2: Converting to a Decimal
We can also solve this problem by first converting the fraction 2/3 into a decimal.
- Divide the numerator by the denominator: 2 / 3 = 0.666... (a recurring decimal)
- Multiply the decimal by the whole number: 0.666... * 32 ≈ 21.333...
This method yields the same result as Method 1, confirming our answer. The slight difference might arise from rounding during the decimal conversion.
Method 3: Finding a Fraction of a Multiple
Sometimes, recognizing that the whole number is a multiple of the denominator can simplify the calculation. While 32 isn't directly divisible by 3, we can still use this approach.
- Find a multiple of the denominator: The closest multiple of 3 to 32 is 30.
- Calculate the fraction of the multiple: (2/3) * 30 = 20
- Calculate the fraction of the remaining amount: 32 - 30 = 2. Then find (2/3) of 2: (2/3) * 2 ≈ 1.333...
- Add the two results: 20 + 1.333... = 21.333...
This method might seem more complex for this specific problem, but it is a useful strategy when dealing with larger numbers and more complex fractions.
Understanding the Remainder
Notice that in all methods, we end up with a decimal value that includes a recurring decimal (0.333...). This is because 32 is not evenly divisible by 3. The remainder represents the portion of the whole number that doesn't neatly fit into the thirds.
Applications in Real-World Scenarios
Fractional calculations are ubiquitous in everyday life. Here are some examples showing the practical application of understanding how to calculate fractions of numbers:
- Cooking: A recipe calls for 2/3 cup of flour. You need to double the recipe. You would calculate (2/3) * 2 cups = 4/3 cups or 1 and 1/3 cups.
- Shopping: A sale offers 1/3 off an item that costs $32. You would save (1/3) * $32 ≈ $10.67.
- Construction: A builder needs to cut a 32-inch piece of wood into thirds. Each piece would be approximately 10.67 inches long.
- Finance: You need to invest 2/3 of your $32,000 savings. The amount you need to invest is (2/3) * $32,000 = $21,333.33.
- Data Analysis: In data analysis, you might need to find a fraction of a dataset to represent a sample. This calculation is fundamental for statistical analysis.
Expanding on Fractions: Related Concepts
Understanding fractions is key to mastering other mathematical concepts:
- Decimals: As we saw, fractions can easily be converted to decimals, and vice-versa. This conversion is essential for different calculations and representations.
- Percentages: Percentages are essentially fractions with a denominator of 100. For example, 2/3 is approximately 66.67%.
- Ratios and Proportions: Fractions form the basis of understanding ratios and proportions, which are vital in various fields, including scaling, mixing ingredients, and mapping.
- Algebra: Fractions frequently appear in algebraic equations and expressions, requiring a firm grasp of fractional operations for solving.
Advanced Applications: Beyond Simple Calculations
While our example focuses on a simple calculation, understanding fractions extends to more complex mathematical concepts:
- Calculus: Fractions are fundamental to calculus, appearing in derivatives, integrals, and limits.
- Probability and Statistics: Fractions are used extensively to express probabilities and proportions in statistical analysis and probability theory.
- Geometry: Fractions play a role in calculating areas, volumes, and proportions in geometric shapes.
Conclusion: Mastering Fractions for a Brighter Future
The seemingly simple problem of "what is 2/3 of 32?" serves as a gateway to understanding a vast and essential area of mathematics. From everyday tasks to complex scientific calculations, a strong grasp of fractions is invaluable. By mastering the methods outlined in this article and exploring the related concepts, you equip yourself with a crucial skillset for academic success and problem-solving in various real-world scenarios. Remember that practice is key – the more you work with fractions, the more comfortable and confident you will become. And don’t hesitate to explore additional resources and practice problems to solidify your understanding of this fundamental mathematical concept.
Latest Posts
Latest Posts
-
421 Divided By 27 With Remainder
May 25, 2025
-
You Re Compatible With 0 Of The Female Population Test
May 25, 2025
-
What Is The Greatest Common Factor Of 26 And 52
May 25, 2025
-
What Percent Of 25 Is 3
May 25, 2025
-
How Do I Measure My Roof
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Is 2 3 Of 32 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.