What Is 2 3 Of 25
listenit
May 23, 2025 · 5 min read
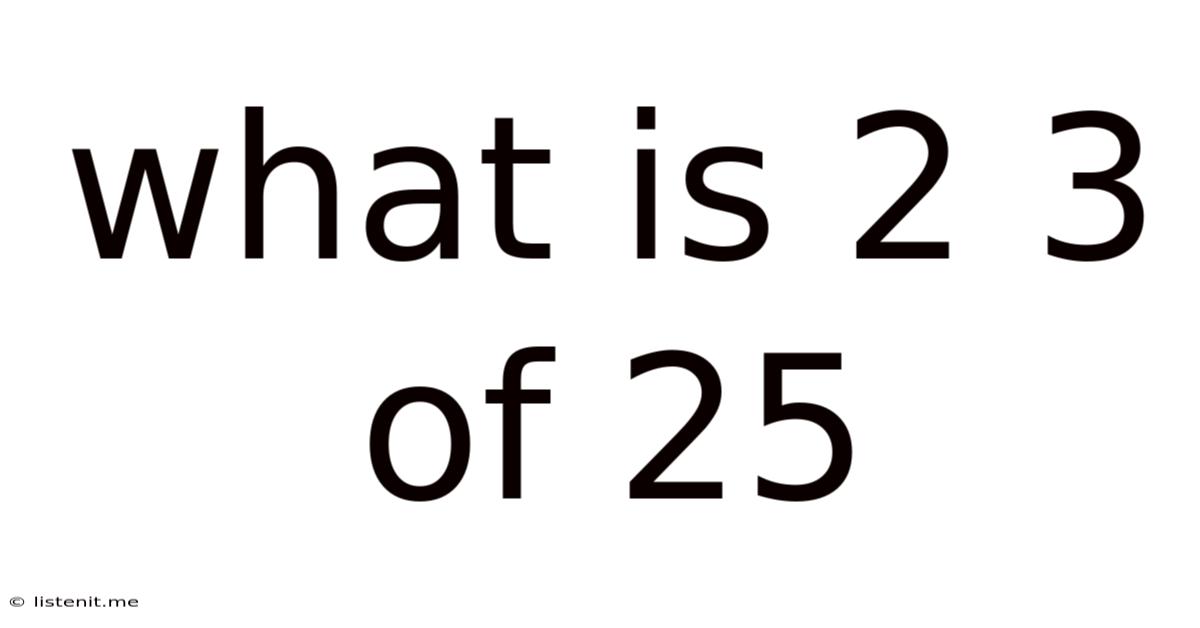
Table of Contents
What is 2/3 of 25? A Comprehensive Guide to Fractions and Percentages
Finding a fraction of a number is a fundamental mathematical skill with broad applications in everyday life, from calculating discounts to determining recipe proportions. This article will comprehensively explore how to calculate 2/3 of 25, explaining the process step-by-step and offering various approaches to solving similar problems. We'll also delve into the broader context of fractions, percentages, and their practical uses.
Understanding Fractions
A fraction represents a part of a whole. It consists of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts you have, while the denominator indicates how many parts make up the whole. In our case, we have the fraction 2/3, where 2 is the numerator and 3 is the denominator.
Key Concepts Related to Fractions:
-
Equivalent Fractions: These are fractions that represent the same value, even though they have different numerators and denominators. For example, 1/2, 2/4, and 3/6 are all equivalent fractions. Understanding equivalent fractions is crucial for simplifying calculations and finding common denominators.
-
Simplifying Fractions: Reducing a fraction to its simplest form involves dividing both the numerator and the denominator by their greatest common divisor (GCD). This makes the fraction easier to understand and work with. For instance, 4/8 can be simplified to 1/2 by dividing both the numerator and denominator by 4.
-
Improper Fractions and Mixed Numbers: An improper fraction has a numerator larger than its denominator (e.g., 7/4). A mixed number combines a whole number and a fraction (e.g., 1 ¾). It's often easier to work with improper fractions when performing calculations.
-
Converting Between Fractions, Decimals, and Percentages: These three represent the same value but in different forms. Understanding how to convert between them expands your problem-solving toolkit. We'll explore this further in the context of our problem.
Calculating 2/3 of 25: The Step-by-Step Approach
There are several ways to calculate 2/3 of 25. Let's explore the most common methods:
Method 1: Direct Multiplication
The most straightforward approach involves multiplying the fraction by the whole number:
(2/3) * 25
To perform this multiplication, we can rewrite 25 as a fraction (25/1):
(2/3) * (25/1)
Now, multiply the numerators together and the denominators together:
(2 * 25) / (3 * 1) = 50/3
This results in an improper fraction. To convert it into a mixed number, we divide the numerator (50) by the denominator (3):
50 ÷ 3 = 16 with a remainder of 2.
Therefore, 50/3 is equivalent to 16 ⅔.
Method 2: Finding One-Third First
Instead of directly multiplying, we can first find one-third of 25 and then multiply that result by 2.
One-third of 25 is:
25/3 = 8⅓
Now, multiply this result by 2:
2 * (8⅓) = 16⅔
This method breaks down the calculation into smaller, potentially easier steps.
Method 3: Using Decimal Equivalents
We can convert the fraction 2/3 into its decimal equivalent:
2 ÷ 3 ≈ 0.6667 (rounded to four decimal places)
Now, multiply the decimal equivalent by 25:
0.6667 * 25 ≈ 16.6675
Rounding to two decimal places, we get 16.67. Note that this method introduces a slight rounding error due to the repeating decimal nature of 2/3.
The Importance of Understanding Fractions in Real-World Scenarios
The ability to calculate fractions is essential in many everyday situations:
-
Cooking and Baking: Recipes often require fractional amounts of ingredients. Understanding fractions is vital for accurate measurements and successful cooking.
-
Shopping and Discounts: Calculating discounts and sales prices often involves working with fractions and percentages. For instance, a 20% discount is equivalent to subtracting 1/5 of the original price.
-
Finance and Budgeting: Managing personal finances requires understanding fractions and percentages for things like interest rates, loan repayments, and investment returns.
-
Construction and Engineering: Precision in construction and engineering depends on accurate measurements and calculations, often expressed as fractions.
-
Data Analysis: Representing data visually and interpreting it often involves using fractions and percentages in graphs and charts.
Converting Fractions to Percentages and Vice Versa
Converting between fractions and percentages provides another way to solve problems involving fractions. A percentage is simply a fraction with a denominator of 100.
To convert a fraction to a percentage, multiply the fraction by 100%:
(2/3) * 100% = 66.67% (approximately)
To convert a percentage to a fraction, divide the percentage by 100 and simplify the resulting fraction:
66.67% ÷ 100 = 0.6667
This decimal can be approximated to 2/3.
Advanced Concepts and Applications
While calculating 2/3 of 25 provides a basic understanding of fractions, more complex scenarios might involve:
-
Calculating fractions of fractions: This involves multiplying fractions together. For example, what is 1/4 of 2/3 of 25?
-
Solving equations involving fractions: Algebraic equations often contain fractions, requiring manipulation to isolate the variable.
-
Working with mixed numbers: Operations involving mixed numbers require careful conversion to improper fractions before performing calculations.
-
Applying fractions in geometry: Calculating areas, volumes, and proportions of geometric shapes often necessitates fractional calculations.
Conclusion: Mastering Fractions for Everyday Success
This comprehensive guide demonstrates how to calculate 2/3 of 25 using various methods and highlights the practical significance of understanding fractions. Whether it's in cooking, shopping, finance, or any other aspect of life, mastering the concepts of fractions, decimals, and percentages is a valuable skill that significantly enhances problem-solving abilities and contributes to success in many endeavors. The ability to confidently work with fractions empowers you to tackle a wide range of challenges with accuracy and efficiency. Remember to practice regularly to solidify your understanding and build confidence in your mathematical skills.
Latest Posts
Latest Posts
-
What Is 1 7 Of 28
May 23, 2025
-
How Many Btus To Cool A Room
May 23, 2025
-
What Is 4 Months From Now
May 23, 2025
-
What Is 30 Dollars With 20 Percent Off
May 23, 2025
-
What Day Of The Week Is June 4th
May 23, 2025
Related Post
Thank you for visiting our website which covers about What Is 2 3 Of 25 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.