What Is 1 7 Of 14
listenit
May 24, 2025 · 5 min read
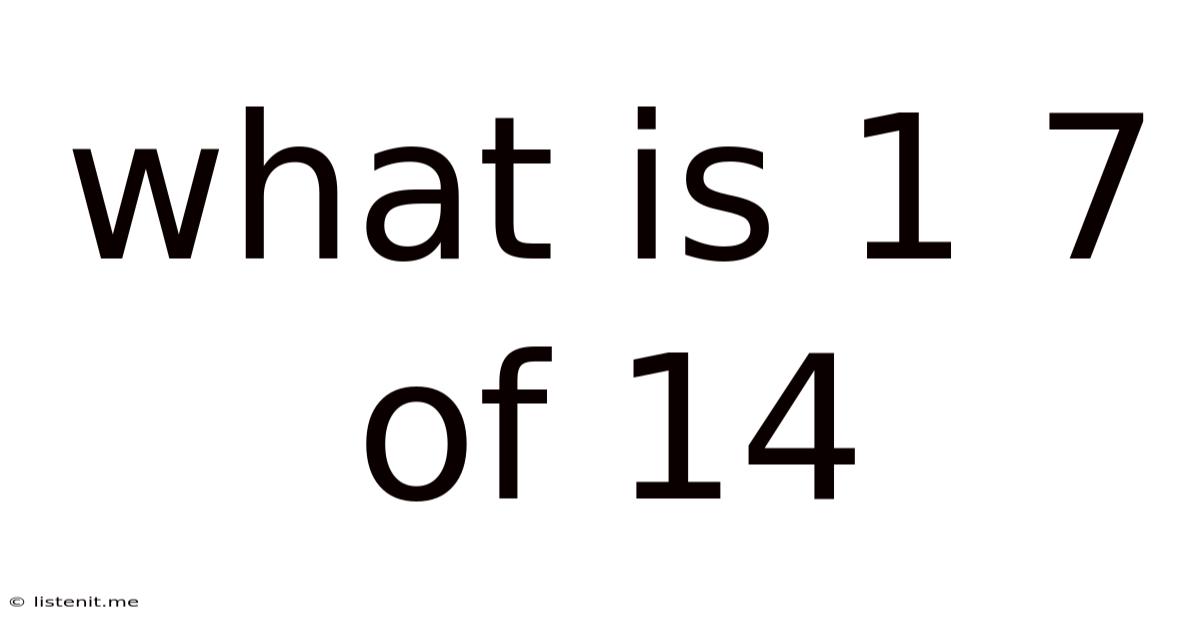
Table of Contents
What is 1/7 of 14? A Deep Dive into Fractions and Their Applications
This seemingly simple question, "What is 1/7 of 14?", opens the door to a fascinating exploration of fractions, their practical applications, and the fundamental principles of mathematics. While the answer itself is straightforward, understanding the underlying concepts provides a strong foundation for tackling more complex mathematical problems. This article will not only solve the problem but also delve into the broader implications of fractional calculations, exploring different methods of solving similar problems and highlighting real-world applications.
Understanding Fractions: A Foundation for Calculation
Before we tackle the specific problem, let's refresh our understanding of fractions. A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (the top number) and the denominator (the bottom number). The numerator indicates how many parts we have, while the denominator indicates how many equal parts the whole is divided into. In our case, we're dealing with the fraction 1/7, meaning one part out of seven equal parts.
Key Concepts in Fraction Arithmetic
Several key concepts are crucial for working with fractions:
-
Multiplication of Fractions: To find a fraction of a number, we multiply the fraction by the number. This involves multiplying the numerator of the fraction by the number and keeping the denominator the same.
-
Simplification of Fractions: After performing calculations, it's often necessary to simplify the resulting fraction to its lowest terms. This involves finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by the GCD.
-
Conversion to Decimals: Fractions can be converted to decimals by dividing the numerator by the denominator. This provides an alternative representation of the fraction, which can be helpful in certain contexts.
Solving the Problem: What is 1/7 of 14?
Now, let's tackle the problem at hand: finding 1/7 of 14. Following the principles of fraction multiplication, we perform the following calculation:
(1/7) * 14 = 14/7
This simplifies to:
14/7 = 2
Therefore, 1/7 of 14 is 2.
Alternative Methods for Solving the Problem
While the direct multiplication method is the most straightforward, let's explore alternative approaches that offer valuable insights into fractional arithmetic:
Method 1: Visual Representation
Imagine a pizza cut into seven equal slices. 1/7 represents one of those slices. If we have 14 slices (two whole pizzas), each representing a unit of 1, then 1/7 of those 14 slices is simply two slices. This visual approach provides an intuitive understanding of the calculation.
Method 2: Using Equivalent Fractions
We can express 14 as a fraction with a denominator of 7: 14/1. Then, the problem becomes:
(1/7) * (14/1) = (1 * 14) / (7 * 1) = 14/7 = 2
This demonstrates the flexibility of working with equivalent fractions.
Real-World Applications of Fraction Calculations
Understanding fractions and their applications extends far beyond theoretical mathematics. Here are a few real-world examples:
Cooking and Baking:
Recipes often use fractions to specify ingredient amounts. For example, a recipe might call for 1/2 cup of sugar or 2/3 cup of flour. Accurately measuring these amounts requires a thorough understanding of fractions.
Construction and Engineering:
Precision is paramount in construction and engineering. Fractions are used extensively in blueprints and measurements to ensure accuracy and structural integrity. The precise dimensions of components are frequently expressed as fractions.
Finance and Economics:
Fractions play a crucial role in financial calculations. Interest rates, stock prices, and other financial figures are often expressed as fractions or decimals.
Data Analysis and Statistics:
Data analysis frequently involves dealing with fractions and percentages. Representing parts of a whole, ratios, and proportions relies heavily on fractional calculations.
Everyday Life:
From sharing equally (dividing something among several people), to calculating discounts, fractions permeate our daily lives. Understanding these concepts simplifies many commonplace tasks.
Expanding the Concept: Beyond 1/7 of 14
Having solved this specific problem, let's generalize the approach to tackle similar problems:
To find a fraction (a/b) of a number (c), we use the following formula:
(a/b) * c = (a * c) / b
This formula provides a universal framework for calculating a fraction of any number.
Advanced Applications and Challenges
While the initial problem was straightforward, the world of fractions extends into far more complex scenarios. Consider these challenges:
-
Working with mixed numbers: Problems involving mixed numbers (whole numbers and fractions combined) require a slightly different approach, often involving conversion to improper fractions before calculation.
-
Dealing with complex fractions: Problems involving fractions within fractions (complex fractions) necessitate a structured approach to simplification.
-
Solving equations involving fractions: Algebraic equations containing fractions demand a systematic method to isolate the unknown variable.
Mastering these advanced applications requires a solid foundation in the fundamental principles of fractions.
Conclusion: The Importance of Understanding Fractions
While the answer to "What is 1/7 of 14?" might appear simple at first glance, a deep dive into the calculation reveals the underlying importance of fractions and their widespread applications. From everyday tasks to complex scientific calculations, understanding fractions is an essential skill that empowers individuals to navigate a wide range of mathematical and real-world problems. The ability to perform these calculations accurately and efficiently is a crucial stepping stone in advancing mathematical literacy and problem-solving abilities. This comprehensive exploration underscores the value of mastering these fundamental mathematical concepts and demonstrates their practical relevance in numerous contexts.
Latest Posts
Latest Posts
-
What Is 20 Of 10 Dollars
May 24, 2025
-
1 3 5 6 1 X
May 24, 2025
-
What Month Is 8 Months From Now
May 24, 2025
-
What Percent Is 30 Out Of 35
May 24, 2025
-
How Do You Calculate Depreciation Of Equipment
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 7 Of 14 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.