What Is 1 4 Of 2
listenit
May 10, 2025 · 5 min read
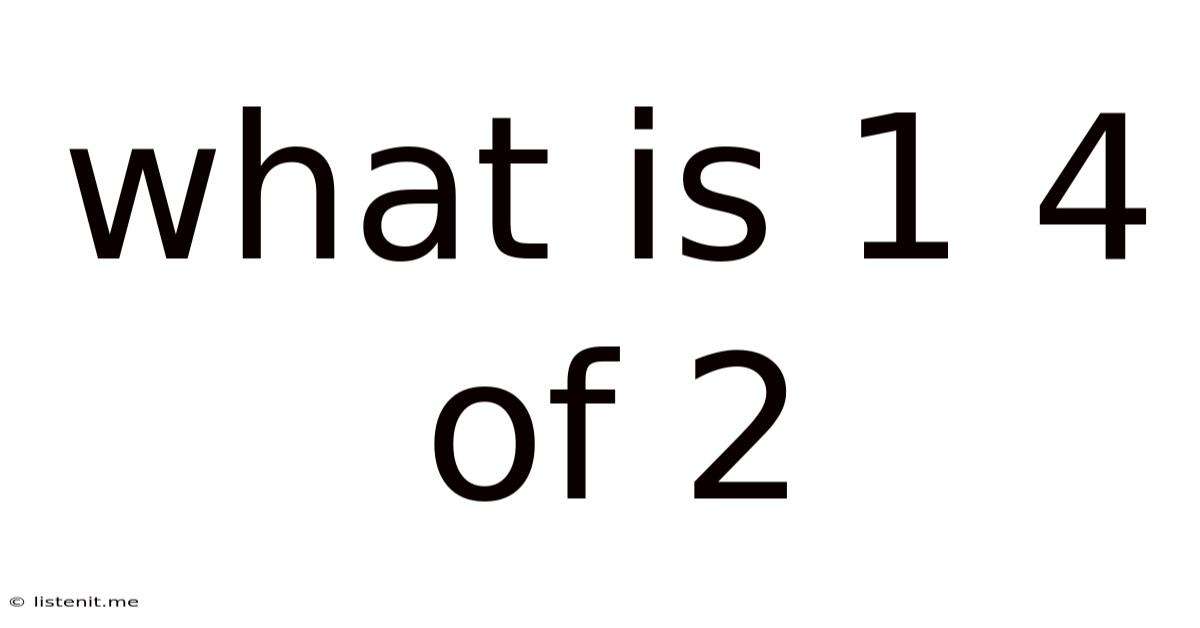
Table of Contents
What is 1/4 of 2? A Deep Dive into Fractions and Their Applications
The seemingly simple question, "What is 1/4 of 2?" opens a door to a fascinating world of mathematical concepts, practical applications, and problem-solving strategies. While the answer itself is straightforward, understanding the underlying principles enhances our ability to tackle more complex fractional calculations and real-world scenarios. This article will delve into the solution, exploring various methods of calculation, discussing the significance of fractions in different fields, and providing practical examples to solidify your understanding.
Understanding Fractions: The Building Blocks of Calculation
Before we tackle the specific problem, let's establish a firm understanding of fractions. A fraction represents a part of a whole. It consists of two essential components:
- Numerator: The top number, indicating the number of parts we are considering.
- Denominator: The bottom number, representing the total number of equal parts the whole is divided into.
In our question, "1/4 of 2," 1 is the numerator and 4 is the denominator. This means we're interested in one out of four equal parts of the whole number 2.
Method 1: Direct Multiplication
The most straightforward way to calculate 1/4 of 2 is through direct multiplication. We multiply the fraction (1/4) by the whole number (2):
(1/4) * 2 = 2/4
This simplifies to 1/2 or 0.5. Therefore, 1/4 of 2 is 1/2 or 0.5.
Method 2: Division First, Then Multiplication
Alternatively, we can approach the problem by first dividing the whole number by the denominator and then multiplying by the numerator.
- Divide: Divide 2 by the denominator (4): 2 ÷ 4 = 0.5
- Multiply: Multiply the result by the numerator (1): 0.5 * 1 = 0.5
This method yields the same answer: 0.5 or 1/2.
Method 3: Visual Representation
For a more intuitive understanding, particularly for beginners, a visual representation can be incredibly helpful. Imagine a rectangle representing the whole number 2. To find 1/4 of 2, we divide the rectangle into four equal parts. Each part represents 1/4 of 2. One of these four parts visually demonstrates that 1/4 of 2 is 1/2.
[Insert image here: A rectangle divided into four equal parts, with one part shaded to represent 1/2]
The Significance of Fractions in Various Fields
Fractions are not merely abstract mathematical concepts; they play a crucial role in numerous fields:
1. Engineering and Construction:
Precision is paramount in engineering and construction. Fractions are essential for accurate measurements, calculations of materials, and ensuring structural integrity. For example, blueprints often utilize fractions for precise dimensions.
2. Cooking and Baking:
Recipes frequently rely on fractions for accurate ingredient measurements. Understanding fractions is vital for achieving desired results in cooking and baking. A recipe calling for 1/4 cup of sugar requires precise measurement to ensure the final product's quality.
3. Finance and Economics:
Fractions and percentages (which are essentially fractions expressed as hundredths) are fundamental to financial calculations. Interest rates, stock prices, and financial ratios all involve fractional calculations. Understanding fractional concepts is essential for sound financial decision-making.
4. Science and Medicine:
Scientific experiments and medical dosages often require precise measurements involving fractions. In chemistry, precise calculations using fractions are critical for accurate mixing of solutions and chemical reactions. In medicine, accurate dosage calculations are critical for patient safety and treatment efficacy.
5. Everyday Life:
Even in our daily lives, fractions are ubiquitous. Sharing equally, calculating discounts, measuring ingredients, and understanding time (e.g., 1/2 hour) all involve fractional concepts.
Solving More Complex Fractional Problems: Building on the Basics
The fundamental principle learned from solving "1/4 of 2" – multiplication of a fraction by a whole number – forms the basis for solving more complex fractional problems. Consider these examples:
- Finding 3/5 of 10: (3/5) * 10 = 30/5 = 6
- Finding 2/3 of 12: (2/3) * 12 = 24/3 = 8
- Finding 1/8 of 16: (1/8) * 16 = 16/8 = 2
These examples highlight that the process remains consistent: multiply the fraction by the whole number and simplify the resulting fraction.
Practical Applications and Real-World Scenarios
Let's explore how this understanding of fractions can be applied in real-world scenarios:
Scenario 1: Sharing Pizza
Imagine you have two pizzas, and you want to divide them equally among four people. Each person gets 1/4 of the total pizza (2 pizzas). This is equivalent to 1/4 * 2 = 1/2 of a pizza per person.
Scenario 2: Discount Calculation
A store offers a 25% discount on a $20 item. A 25% discount is equivalent to 1/4. The discount amount is 1/4 * $20 = $5. The final price after the discount is $20 - $5 = $15.
Scenario 3: Ingredient Measurement
A recipe requires 1/4 cup of sugar for every 2 cups of flour. If you want to double the recipe, you'll need 1/4 * 4 = 1 cup of sugar.
Mastering Fractions: Tips and Techniques
Becoming proficient in fraction calculations requires consistent practice and a strategic approach:
- Simplify Fractions: Always simplify fractions to their lowest terms (e.g., 2/4 simplifies to 1/2).
- Convert to Decimals: Converting fractions to decimals can facilitate calculations, especially when dealing with more complex problems.
- Use Visual Aids: Visual aids like diagrams and drawings can be extremely helpful in understanding and solving fraction problems, particularly for visual learners.
- Practice Regularly: Consistent practice is key to mastering fractions. Work through a variety of problems, ranging from simple to complex, to build your understanding and confidence.
Conclusion: The Enduring Importance of Fractions
The seemingly simple question, "What is 1/4 of 2?" serves as a gateway to understanding the profound importance of fractions in mathematics and various aspects of life. Mastering fractions is not just about solving mathematical problems; it's about cultivating a deeper understanding of proportions, ratios, and the ability to solve real-world problems with accuracy and precision. Through consistent practice and application, anyone can achieve proficiency in fractions and unlock their practical utility in countless scenarios. The ability to calculate fractions accurately is a valuable skill that empowers individuals to tackle complex problems and make informed decisions in various fields, from everyday life to specialized professions.
Latest Posts
Latest Posts
-
How Are The Isotopes Of An Element Similar
May 10, 2025
-
What Is The Mixed Number For 5 3
May 10, 2025
-
Which Molecule Contains A Nonpolar Covalent Bond
May 10, 2025
-
Ground State Electron Configuration For Copper
May 10, 2025
-
Decomposers Are Important To Ecosystems Because They
May 10, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 4 Of 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.