What Is 1 3 Of 40000
listenit
May 24, 2025 · 4 min read
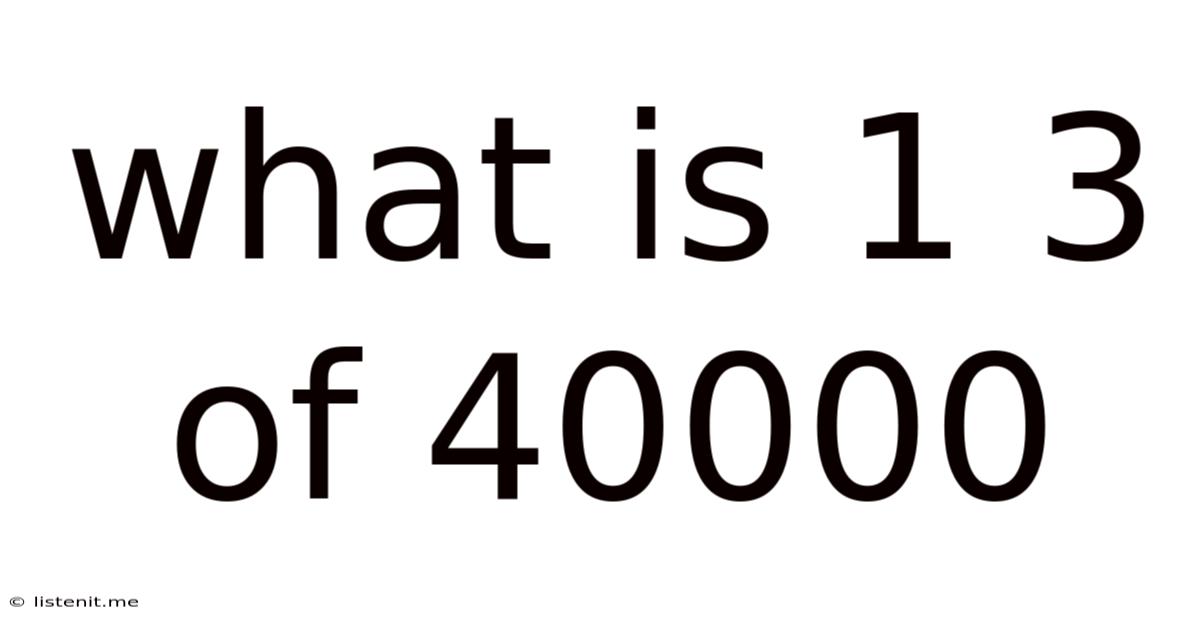
Table of Contents
What is 1/3 of 40,000? A Comprehensive Exploration of Fractions and Their Applications
Finding a fraction of a number is a fundamental mathematical operation with wide-ranging applications in everyday life, from calculating discounts and taxes to understanding proportions in various fields like finance, engineering, and cooking. This article delves into the calculation of 1/3 of 40,000, explaining the process step-by-step, exploring different methods, and highlighting the practical significance of understanding fractions.
Understanding Fractions:
Before tackling the specific problem, let's solidify our understanding of fractions. A fraction represents a part of a whole. It's composed of two numbers: the numerator, which is the top number, and the denominator, which is the bottom number. The denominator indicates the total number of equal parts the whole is divided into, while the numerator shows how many of those parts are being considered.
In our case, we have the fraction 1/3. This means we're interested in one out of three equal parts of a whole.
Calculating 1/3 of 40,000:
There are several ways to calculate 1/3 of 40,000. Let's explore the most common methods:
Method 1: Direct Multiplication:
The simplest method involves direct multiplication. To find 1/3 of 40,000, we multiply 40,000 by 1/3:
40,000 × (1/3) = 40,000 / 3
Performing the division:
40,000 ÷ 3 = 13,333.333...
Therefore, 1/3 of 40,000 is approximately 13,333.33. Note that this result is a recurring decimal.
Method 2: Division First, Then Multiplication:
This method involves dividing 40,000 by the denominator (3) first, and then multiplying the result by the numerator (1). This approach is particularly useful when dealing with larger numbers or more complex fractions.
- Divide 40,000 by 3: 40,000 ÷ 3 ≈ 13,333.33
- Multiply the result by the numerator (1): 13,333.33 × 1 = 13,333.33
Again, we arrive at the approximate answer of 13,333.33.
Method 3: Using Decimal Equivalents:
We can convert the fraction 1/3 into its decimal equivalent, which is approximately 0.3333... Then, we multiply this decimal by 40,000:
40,000 × 0.3333... ≈ 13,333.33
This method yields the same result as the previous ones.
Understanding the Recurring Decimal:
It's important to acknowledge the recurring decimal (3) in the answer. This indicates that the result is not an exact whole number but rather an infinitely repeating decimal. In practical applications, we often round the result to a suitable number of decimal places, depending on the required level of accuracy. For most purposes, rounding to two decimal places (13,333.33) is sufficient.
Practical Applications of Finding Fractions:
The ability to calculate fractions has numerous practical applications across various domains:
1. Finance:
- Calculating interest: Interest calculations often involve fractions. For instance, calculating simple interest on a loan or investment requires understanding fractions of the principal amount.
- Determining discounts: Retail discounts are often expressed as fractions (e.g., 1/3 off, 2/5 off). Calculating the discounted price requires finding the fractional part of the original price.
- Dividing profits or expenses: In partnerships or business ventures, profits or expenses may need to be divided proportionally, which requires calculating fractions of the total amount.
2. Engineering and Construction:
- Scaling blueprints: Engineers and architects frequently work with scaled drawings, using fractions to represent the relationship between the drawing dimensions and the actual dimensions.
- Calculating material quantities: Determining the quantity of materials needed for a construction project often involves calculating fractions of the total volume or area.
3. Cooking and Baking:
- Following recipes: Many recipes call for fractional amounts of ingredients. Understanding fractions is essential for accurate measurements.
- Scaling recipes: Scaling recipes up or down requires accurately calculating the fractional amounts of each ingredient.
4. Data Analysis:
- Representing proportions: Fractions are frequently used to represent proportions or percentages in data analysis and statistics.
- Interpreting statistical results: Many statistical analyses yield results expressed as fractions, which need to be interpreted in the context of the problem.
5. Everyday Life:
- Sharing items: Dividing items equally among a group of people often involves calculating fractions.
- Understanding proportions: Understanding fractions helps in understanding ratios and proportions in various everyday situations.
Beyond 1/3 of 40,000: Extending the Concept:
The principles discussed above can be extended to calculate any fraction of any number. The core concept remains the same: multiply the number by the fraction, which involves dividing the number by the denominator and then multiplying by the numerator.
Conclusion:
Calculating 1/3 of 40,000, which is approximately 13,333.33, demonstrates the fundamental importance of understanding and applying fractional calculations. This seemingly simple operation has profound implications across diverse fields, from managing finances to designing structures and even baking a cake. Mastering fractional arithmetic empowers individuals to solve practical problems, interpret data effectively, and confidently navigate various aspects of life. The ability to work with fractions is a crucial skill that contributes significantly to both numerical literacy and overall problem-solving capabilities. By understanding the various methods available for calculating fractions and appreciating their practical applications, individuals can enhance their mathematical skills and gain a more comprehensive understanding of the world around them.
Latest Posts
Latest Posts
-
How Many Hours In 6 Years
May 24, 2025
-
What Is 30 Off 40 Dollars
May 24, 2025
-
Born 1991 How Old In 2024
May 24, 2025
-
30 Days From February 10 2024
May 24, 2025
-
What Is The Date 15 Days From Today
May 24, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 3 Of 40000 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.