What Is 1 2 Of 25
listenit
May 25, 2025 · 5 min read
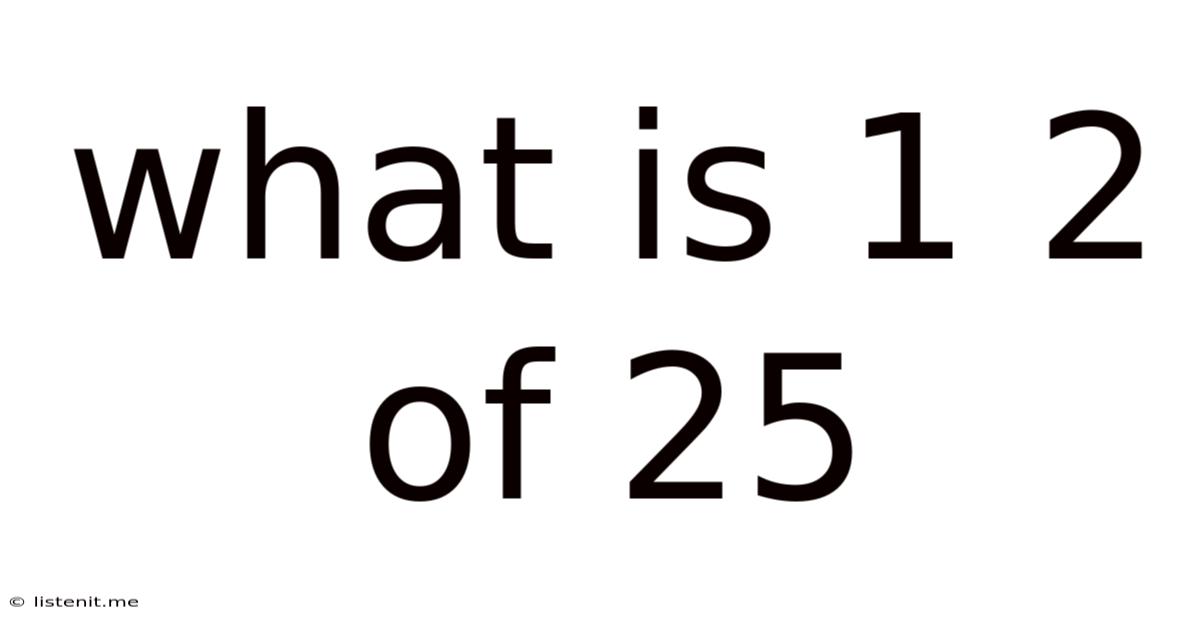
Table of Contents
What is 1/2 of 25? A Deep Dive into Fractions, Percentages, and Practical Applications
Finding half of a number is a fundamental concept in mathematics, crucial for everyday life and advanced calculations. This seemingly simple question, "What is 1/2 of 25?", opens the door to understanding fractions, percentages, decimals, and their practical applications in various fields. Let's explore this concept in detail, covering different approaches, real-world examples, and advanced implications.
Understanding Fractions: The Building Blocks of 1/2 of 25
The expression "1/2 of 25" represents a fraction. A fraction is a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). In the fraction 1/2, 1 is the numerator and 2 is the denominator. The denominator indicates how many equal parts the whole is divided into, while the numerator shows how many of those parts we are considering. In this case, 1/2 signifies one out of two equal parts.
To find 1/2 of 25, we need to divide 25 into two equal parts. This can be represented mathematically as:
(1/2) * 25
Method 1: Direct Multiplication
The most straightforward method involves directly multiplying the fraction by the whole number:
(1/2) * 25 = 25/2
This results in an improper fraction (where the numerator is larger than the denominator). To convert it into a mixed number (a whole number and a fraction), we divide the numerator (25) by the denominator (2):
25 ÷ 2 = 12 with a remainder of 1.
Therefore, 25/2 = 12 1/2 or 12.5
Therefore, 1/2 of 25 is 12.5
Method 2: Division by the Denominator
Another approach involves dividing the whole number (25) by the denominator of the fraction (2) and then multiplying the result by the numerator (1):
25 ÷ 2 = 12.5
12.5 * 1 = 12.5
This method directly yields the answer in decimal form. Again, we arrive at the same conclusion: 1/2 of 25 is 12.5
Method 3: Using Percentages
Fractions and percentages are closely related. The fraction 1/2 is equivalent to 50%. To find 50% of 25, we can use the following formula:
(Percentage/100) * Whole Number = Part
(50/100) * 25 = 0.5 * 25 = 12.5
This method demonstrates the interchangeability between fractions and percentages in solving such problems. Once again, we confirm that 1/2 of 25 is 12.5
Real-World Applications of Finding Half a Number
The ability to find half of a number is incredibly practical in various situations:
-
Sharing Equally: If you have 25 cookies and want to share them equally between two friends, you would give each friend 12.5 cookies. In reality, you'd likely give each friend 12 cookies and keep 1 for yourself or break the remaining cookie in half.
-
Cooking and Baking: Many recipes require halving or doubling ingredients. Knowing how to find half of a quantity (like 25 grams of sugar) is essential for accurate baking.
-
Calculating Discounts: A 50% discount means you pay half the original price. If an item costs $25, a 50% discount reduces the price by $12.50.
-
Geometry and Measurement: Finding the midpoint of a line segment or calculating the area of a triangle often involves finding half of a given measurement.
-
Financial Calculations: Dividing profits or expenses equally between partners frequently requires calculating half of a total amount.
-
Data Analysis: When dealing with datasets, calculating the median often involves finding the middle value, which might necessitate finding half of a total count.
Extending the Concept: Beyond 1/2 of 25
Understanding the principle behind finding 1/2 of 25 allows us to tackle more complex fraction problems. For example:
-
What is 3/4 of 25? We can break this down: 1/4 of 25 is 25/4 = 6.25. Therefore, 3/4 of 25 is 6.25 * 3 = 18.75
-
What is 2/5 of 25? 1/5 of 25 is 25/5 = 5. Therefore, 2/5 of 25 is 5 * 2 = 10
These examples showcase the general principle: To find a fraction of a number, you divide the number by the denominator and multiply the result by the numerator.
Decimals and Their Role
The answer 12.5 is a decimal number. Decimals represent parts of a whole, similar to fractions. The decimal point separates the whole number part from the fractional part. In 12.5, "12" represents the whole number, and ".5" represents one-half (or 5/10). Decimals are frequently used in calculations involving money, measurements, and scientific data.
Advanced Applications: Algebra and Beyond
The concept of finding a fraction of a number forms the basis for many advanced mathematical concepts. In algebra, this is often expressed using variables. For instance, finding "x/2" of "y" would be represented as (x/2)*y = xy/2. This allows for the solution of more complex equations and problems involving unknown quantities. This is fundamental in areas like calculus and other higher-level mathematical disciplines.
Conclusion: The Importance of Mastering Fractions
Understanding how to find a fraction of a number, such as 1/2 of 25, is a fundamental skill with widespread applications. From everyday tasks like sharing resources and following recipes to more advanced mathematical concepts, this skill is crucial for success in various fields. Mastering fractions and decimals lays a solid foundation for more complex mathematical endeavors, making it a critical element of a well-rounded mathematical education. The simple act of calculating half of 25 opens doors to a world of mathematical possibilities and practical problem-solving.
Latest Posts
Latest Posts
-
30 Days From July 13 2024
May 25, 2025
-
Least Common Multiple Of 30 And 75
May 25, 2025
-
867 000 Rounded To The Nearest Hundred Thousand
May 25, 2025
-
What Is 30 Percent Of 85
May 25, 2025
-
How Many Hours Away Is 6 Am
May 25, 2025
Related Post
Thank you for visiting our website which covers about What Is 1 2 Of 25 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.