The Top Number In A Fraction Is Called
listenit
Mar 29, 2025 · 5 min read
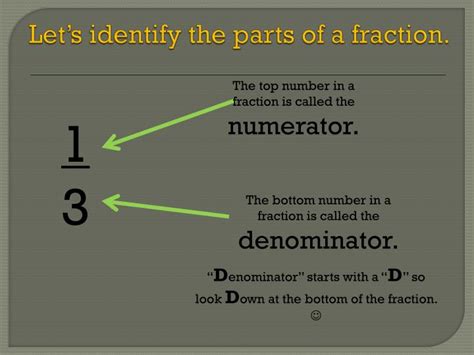
Table of Contents
The Top Number in a Fraction is Called: A Deep Dive into Numerators and Their Importance
The seemingly simple question, "What is the top number in a fraction called?" opens a door to a fascinating world of mathematical concepts. Understanding the terminology and function of this number, the numerator, is fundamental to grasping fractions, performing calculations, and applying this knowledge to various fields. This article delves into the intricacies of numerators, exploring their role in representing parts of a whole, their significance in different mathematical operations, and their applications in real-world scenarios.
Understanding Numerators and Denominators: The Building Blocks of Fractions
A fraction is a representation of a part of a whole. It's expressed as a ratio of two numbers, separated by a horizontal line (or sometimes a slash). The top number, the one we're focusing on, is the numerator. The bottom number is the denominator.
In simpler terms:
- Numerator: Tells us how many parts we have.
- Denominator: Tells us how many equal parts the whole is divided into.
For example, in the fraction ¾, the numerator (3) tells us we have 3 parts, and the denominator (4) tells us the whole is divided into 4 equal parts.
Visualizing Fractions: A Helpful Approach
Visual aids are invaluable for understanding fractions, especially for beginners. Imagine a pizza cut into 4 equal slices. If you eat 3 slices, you've eaten ¾ of the pizza. Here, 3 is the numerator (the number of slices you ate), and 4 is the denominator (the total number of slices).
Beyond Pizza: Real-World Applications of Fractions
Fractions aren't confined to pizza slices. They appear everywhere in daily life:
- Cooking: Following recipes often involves fractions (e.g., ½ cup of sugar).
- Measurements: Lengths, weights, and volumes are frequently expressed as fractions (e.g., 2 ½ inches).
- Time: Telling time involves fractions (e.g., ¼ hour).
- Finance: Understanding percentages and proportions requires a firm grasp of fractions.
The Role of the Numerator in Mathematical Operations
The numerator plays a crucial role in various mathematical operations involving fractions. Let's explore some key examples:
1. Addition and Subtraction of Fractions
Adding or subtracting fractions with the same denominator is straightforward: you simply add or subtract the numerators and keep the denominator the same. For example:
1/5 + 2/5 = (1 + 2)/5 = 3/5
However, adding or subtracting fractions with different denominators requires finding a common denominator before performing the operation. This involves finding the least common multiple (LCM) of the denominators.
2. Multiplication of Fractions
Multiplying fractions is relatively simple. You multiply the numerators together to get the new numerator and multiply the denominators together to get the new denominator. For example:
(2/3) * (4/5) = (2 * 4) / (3 * 5) = 8/15
3. Division of Fractions
Dividing fractions involves inverting the second fraction (swapping the numerator and denominator) and then multiplying. For example:
(1/2) ÷ (3/4) = (1/2) * (4/3) = 4/6 = 2/3
4. Simplifying Fractions
Simplifying, or reducing, a fraction involves dividing both the numerator and the denominator by their greatest common divisor (GCD). This results in an equivalent fraction expressed in its simplest form. For example:
6/9 can be simplified to 2/3 (by dividing both numerator and denominator by 3).
Numerators and Their Significance in Advanced Mathematical Concepts
The numerator's importance extends beyond basic arithmetic. It plays a crucial role in more advanced mathematical concepts:
1. Ratios and Proportions
A ratio is a comparison of two quantities, often expressed as a fraction. The numerator represents one quantity, and the denominator represents the other. Proportions are statements of equality between two ratios. Understanding numerators is essential for solving problems involving ratios and proportions.
2. Probability
In probability, fractions are used to represent the likelihood of an event occurring. The numerator represents the number of favorable outcomes, while the denominator represents the total number of possible outcomes.
3. Algebra
Algebraic expressions often involve fractions. Understanding how numerators behave in different operations (addition, subtraction, multiplication, division) is essential for manipulating and solving algebraic equations.
Numerators in Real-World Applications: Beyond the Classroom
The applications of numerators and fractions extend far beyond the classroom. Here are a few examples:
1. Engineering and Construction
Engineers and builders constantly use fractions for precise measurements and calculations. Understanding fractions is critical for creating accurate blueprints, ensuring structural integrity, and completing projects successfully.
2. Finance and Accounting
Financial calculations frequently involve fractions and percentages. Numerators are used to calculate interest, determine profit margins, and analyze financial data.
3. Data Analysis and Statistics
Data analysis relies heavily on the use of fractions and ratios. Understanding numerators is crucial for interpreting data, identifying trends, and making informed decisions based on statistical analysis.
4. Computer Science and Programming
While often represented differently in code, the underlying logic of fractions is vital in computer graphics, game development, and algorithms that involve proportions and scaling.
Misconceptions and Common Mistakes Involving Numerators
While understanding numerators is fundamental, several common misconceptions can lead to errors:
- Ignoring the denominator: It's crucial to remember that the numerator's value is relative to the denominator. A large numerator doesn't necessarily mean a large fraction if the denominator is even larger.
- Incorrect simplification: Failing to find the greatest common divisor when simplifying fractions can lead to incorrect results.
- Errors in operations: Mistakes in adding, subtracting, multiplying, or dividing fractions are often related to incorrect handling of numerators and denominators.
Conclusion: The Unsung Hero of Fractions - The Numerator
The top number in a fraction, the numerator, is more than just a number; it's a fundamental building block of mathematical understanding. Its role in representing parts of a whole, performing calculations, and applying mathematical concepts to real-world problems is undeniable. A thorough understanding of numerators is crucial for success in various fields, from basic arithmetic to advanced mathematical concepts and their practical applications. Mastering numerators is key to unlocking a deeper understanding of fractions and their pervasive role in our lives. By understanding its significance, we can appreciate the power and utility of this seemingly simple element of mathematics.
Latest Posts
Latest Posts
-
What Are The First 5 Multiples Of 7
Apr 01, 2025
-
What Is 8 10 As A Decimal
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about The Top Number In A Fraction Is Called . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
