The Diagonals Of A Rhombus Are Perpendicular
listenit
Mar 26, 2025 · 6 min read
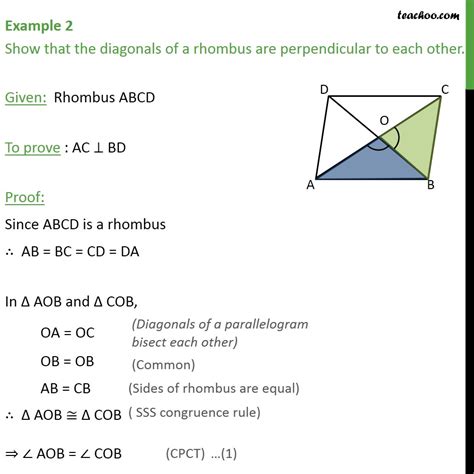
Table of Contents
The Diagonals of a Rhombus are Perpendicular: A Comprehensive Exploration
The geometric properties of a rhombus, a quadrilateral with all sides equal in length, are fascinating and often lead to elegant proofs and applications. One of the most significant properties of a rhombus is that its diagonals are perpendicular. This seemingly simple statement underpins many other geometric relationships and finds practical use in various fields, from engineering to art. This article will delve deep into why this property holds true, exploring multiple proof methods, discussing related theorems, and highlighting its significance in different contexts.
Understanding the Rhombus
Before diving into the proof, let's solidify our understanding of a rhombus. A rhombus is defined as a quadrilateral with four equal sides. This definition, while concise, implies several crucial features:
- Equilateral: All four sides are congruent (equal in length).
- Parallelogram: Opposite sides are parallel. This is a direct consequence of the equal sides and the properties of quadrilaterals.
- Possible Angles: While all sides are equal, the angles can vary, resulting in different types of rhombi, from squares (with all angles equal to 90 degrees) to more elongated shapes.
Proof 1: Using Triangle Congruence
This proof elegantly leverages the properties of congruent triangles. Consider a rhombus ABCD, where AB = BC = CD = DA. Let the diagonals AC and BD intersect at point O.
Steps:
-
Consider triangles ΔABO and ΔCBO: Since AB = BC (sides of the rhombus), AO = CO (diagonals bisect each other in a parallelogram), and BO is a common side, we have SAS congruence (Side-Angle-Side). Therefore, ΔABO ≅ ΔCBO.
-
Angles are Equal: Because the triangles are congruent, their corresponding angles are equal. Specifically, ∠AOB = ∠COB.
-
Linear Pair: ∠AOB and ∠COB form a linear pair, meaning they are adjacent angles on a straight line, and their sum is 180 degrees.
-
Conclusion: Since ∠AOB = ∠COB and ∠AOB + ∠COB = 180°, we can deduce that ∠AOB = ∠COB = 90°. This proves that the diagonals are perpendicular.
Proof 2: Using Vectors
This approach utilizes the power of vector algebra. Let's represent the vertices of the rhombus ABCD using vectors:
- A: Vector a
- B: Vector b
- C: Vector c
- D: Vector d
Since it's a rhombus, the following vector relationships hold true:
- AB = b - a
- BC = c - b
- CD = d - c
- DA = a - d
Furthermore, because opposite sides are parallel and equal in length:
- AB = DC => b - a = d - c
- BC = AD => c - b = a - d
The diagonals are represented by:
- AC = c - a
- BD = d - b
The dot product of two perpendicular vectors is zero. Let's calculate the dot product of AC and BD:
( c - a ) . ( d - b ) = c.d - c.b - a.d + a.b
Using the parallelogram rule (which applies to rhombi), and carefully rearranging the terms, one can show that this dot product simplifies to zero. Therefore, AC and BD are perpendicular. The detailed algebraic manipulation is beyond the scope of this introductory explanation but illustrates the power of vector methods in geometric proofs.
Proof 3: Using the Properties of Parallelograms and Isosceles Triangles
This method combines the properties of parallelograms and isosceles triangles.
-
Parallelogram Properties: Recall that a rhombus is a parallelogram. In a parallelogram, diagonals bisect each other. Therefore, in rhombus ABCD, diagonals AC and BD bisect each other at point O.
-
Isosceles Triangles: Because all sides of a rhombus are equal (AB = BC = CD = DA), consider triangles ΔABC and ΔADC. These are isosceles triangles since AB=BC and AD=DC respectively.
-
Angle Bisectors: The diagonals of the rhombus are angle bisectors in the corresponding isosceles triangles. This means that AC bisects ∠A and ∠C, and BD bisects ∠B and ∠D.
-
Angle Sum: Let's focus on the angles around point O. The sum of angles around a point is 360 degrees. We have four angles around O: ∠AOB, ∠BOC, ∠COD, and ∠DOA. Since the diagonals bisect the angles, these four angles are equal.
-
Deduction: Since the four angles are equal and sum to 360 degrees, each angle must be 90 degrees. This conclusively proves that the diagonals are perpendicular.
Significance and Applications
The perpendicularity of the diagonals in a rhombus is not merely a theoretical curiosity. It has profound implications and numerous practical applications:
-
Area Calculation: The area of a rhombus can be easily calculated using the lengths of its diagonals. The formula is: Area = (1/2) * d1 * d2, where d1 and d2 are the lengths of the diagonals. This simple formula is a direct consequence of the diagonals being perpendicular, creating four congruent right-angled triangles.
-
Construction and Engineering: The property is utilized in various construction and engineering projects. For instance, the design of certain bridges and trusses relies on the inherent stability and strength offered by the rhombus's geometric properties, particularly the perpendicularity of its diagonals.
-
Computer Graphics and Game Development: The rhombus and its properties are crucial in computer graphics and game development. Many 2D and 3D models involve rhombuses, and the knowledge of their diagonal relationships simplifies calculations related to rotation, scaling, and collision detection.
-
Tessellations and Art: Rhombi are frequently used in tessellations, creating aesthetically pleasing and mathematically interesting patterns. The perpendicularity of diagonals is essential in designing and understanding such patterns.
-
Crystallography: In crystallography, the arrangement of atoms in certain crystalline structures can be modeled using rhombuses. The perpendicularity of the diagonals plays a key role in understanding and predicting the properties of these structures.
Further Exploration: Relationship to Other Quadrilaterals
Understanding the rhombus and its diagonal property helps clarify its relationship to other quadrilaterals:
-
Square: A square is a special case of a rhombus where all angles are 90 degrees. Thus, the diagonals of a square are also perpendicular, and they are also equal in length.
-
Rectangle: A rectangle has all four angles equal to 90 degrees but doesn't necessarily have equal sides. While its diagonals bisect each other, they are not necessarily perpendicular unless it is a square.
-
Parallelogram: A parallelogram is a more general quadrilateral with opposite sides parallel. Its diagonals bisect each other but are not necessarily perpendicular unless it is a rhombus or a square.
-
Kite: A kite has two pairs of adjacent sides equal. The diagonals of a kite are perpendicular, but only one diagonal bisects the other. This contrasts with the rhombus, where both diagonals bisect each other.
Conclusion
The seemingly simple statement – "the diagonals of a rhombus are perpendicular" – underpins a rich tapestry of mathematical relationships and practical applications. This article has explored multiple ways to prove this fundamental property, demonstrating the versatility of different mathematical approaches. Understanding this property not only enhances one's geometric knowledge but also provides valuable insights applicable to various fields, highlighting the interconnectedness of mathematics and the real world. The elegance and power of this property continue to inspire mathematicians, engineers, artists, and anyone fascinated by the beauty of geometry.
Latest Posts
Latest Posts
-
Sound Waves Move The Slowest Through Which Medium
Mar 29, 2025
-
Chemical Digestion Of Carbohydrates Begins In The
Mar 29, 2025
-
At What Point During Mitosis Has The Nuclear Membrane Reformed
Mar 29, 2025
-
Solve X 1 X 2 0
Mar 29, 2025
-
What Does Mean At The End Of A Sentence
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about The Diagonals Of A Rhombus Are Perpendicular . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
