Is The Square Root Of 4 Rational
listenit
Mar 29, 2025 · 5 min read
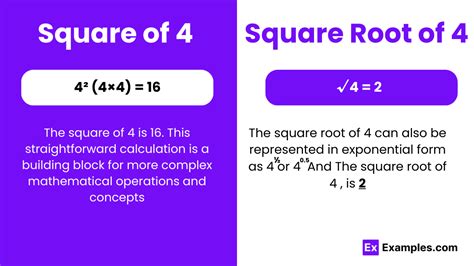
Table of Contents
- Is The Square Root Of 4 Rational
- Table of Contents
- Is the Square Root of 4 Rational? A Deep Dive into Number Theory
- Understanding Rational and Irrational Numbers
- Rational Numbers: The Fractions
- Irrational Numbers: The Unfractions
- Solving the Mystery: Is √4 Rational?
- Expanding the Understanding: Properties of Rational Numbers
- Contrasting with Irrational Numbers: Why Some Square Roots are Irrational
- Real Numbers: The Big Picture
- Practical Applications: Rational Numbers in Everyday Life
- Further Exploration: Beyond the Basics
- Conclusion: A Simple Question, a Deep Understanding
- Latest Posts
- Latest Posts
- Related Post
Is the Square Root of 4 Rational? A Deep Dive into Number Theory
The question, "Is the square root of 4 rational?" might seem trivial at first glance. However, exploring this seemingly simple question provides a fantastic opportunity to delve into fundamental concepts in number theory, specifically rational and irrational numbers. This article will not only answer the question definitively but also explore the broader implications and related mathematical ideas.
Understanding Rational and Irrational Numbers
Before we tackle the square root of 4, let's establish a solid understanding of rational and irrational numbers. This forms the bedrock of our exploration.
Rational Numbers: The Fractions
A rational number is any number that can be expressed as a fraction p/q, where 'p' and 'q' are integers (whole numbers), and 'q' is not zero. Examples of rational numbers abound:
- 1/2: One-half
- 3/4: Three-quarters
- -2/5: Negative two-fifths
- 5: (Can be expressed as 5/1)
- 0: (Can be expressed as 0/1)
Notice that all integers are also rational numbers because they can be expressed with a denominator of 1.
Irrational Numbers: The Unfractions
Irrational numbers, on the other hand, cannot be expressed as a simple fraction of two integers. Their decimal representation is non-terminating (it goes on forever) and non-repeating (it doesn't have a recurring pattern). Famous examples include:
- π (pi): Approximately 3.14159..., the ratio of a circle's circumference to its diameter.
- e (Euler's number): Approximately 2.71828..., the base of the natural logarithm.
- √2 (the square root of 2): Approximately 1.41421..., which cannot be expressed as a fraction.
Understanding the distinction between rational and irrational numbers is crucial for answering our central question.
Solving the Mystery: Is √4 Rational?
Now, let's finally address the question: Is the square root of 4 rational?
The square root of a number 'x' is a value that, when multiplied by itself, equals 'x'. In this case, we're looking for a number that, when multiplied by itself, equals 4. That number is 2.
Therefore, √4 = 2.
Since 2 can be expressed as the fraction 2/1, it perfectly fits the definition of a rational number. Therefore, the square root of 4 is indeed rational.
Expanding the Understanding: Properties of Rational Numbers
The fact that √4 is rational highlights several important properties of rational numbers:
- Closure under addition: The sum of two rational numbers is always a rational number. (e.g., 1/2 + 1/3 = 5/6)
- Closure under subtraction: The difference between two rational numbers is always a rational number. (e.g., 2/3 - 1/4 = 5/12)
- Closure under multiplication: The product of two rational numbers is always a rational number. (e.g., 2/3 * 3/4 = 1/2)
- Closure under division: The quotient of two rational numbers (excluding division by zero) is always a rational number. (e.g., 1/2 ÷ 1/3 = 3/2)
These closure properties are fundamental in algebra and other branches of mathematics. They ensure that rational numbers form a consistent and predictable system.
Contrasting with Irrational Numbers: Why Some Square Roots are Irrational
While the square root of 4 is rational, it's important to understand why the square roots of many other numbers are irrational. Consider √2:
It's been proven that √2 cannot be expressed as a fraction p/q where p and q are integers. The proof, often done through proof by contradiction, demonstrates that any attempt to express √2 as a fraction will inevitably lead to a contradiction. This is a classic example in mathematics illustrating the elegance and power of proof by contradiction.
Real Numbers: The Big Picture
Rational and irrational numbers together form the set of real numbers. Real numbers encompass all numbers that can be plotted on a number line. This includes all integers, rational numbers, and irrational numbers.
Practical Applications: Rational Numbers in Everyday Life
While seemingly abstract, rational numbers are fundamental to countless aspects of our daily lives:
- Measurement: We use fractions and decimals (which are essentially rational numbers) to measure lengths, weights, volumes, and more.
- Finance: Money calculations involve rational numbers, from calculating interest to budgeting expenses.
- Cooking: Recipes often use fractions (e.g., 1/2 cup of flour).
- Construction: Building projects rely heavily on precise measurements, using rational numbers for dimensions and angles.
Without a thorough understanding of rational numbers, many aspects of modern life would be significantly more challenging.
Further Exploration: Beyond the Basics
The exploration of rational and irrational numbers extends far beyond the simple question of whether √4 is rational. Here are some avenues for further exploration:
- Continued Fractions: These offer a unique way to represent both rational and irrational numbers.
- Transcendental Numbers: A subset of irrational numbers that are not roots of any polynomial equation with rational coefficients (examples: π and e).
- Approximating Irrational Numbers: Techniques for approximating irrational numbers with rational numbers to a desired degree of accuracy.
- The Density of Rational and Irrational Numbers: Exploring how these numbers are distributed across the real number line.
Conclusion: A Simple Question, a Deep Understanding
The seemingly simple question of whether the square root of 4 is rational has served as a gateway to a deeper exploration of number theory. Understanding the difference between rational and irrational numbers, their properties, and their applications is crucial for anyone pursuing a deeper understanding of mathematics and its role in the world around us. While the answer to our initial question is a straightforward "yes," the journey to that answer has unveiled a wealth of mathematical concepts and their profound implications. This exploration underscores the beauty of mathematics—even seemingly basic questions can lead to fascinating discoveries.
Latest Posts
Latest Posts
-
What Is It Called When Homologous Chromosomes Exchange Genes
Apr 02, 2025
-
Whats The Square Root Of 39
Apr 02, 2025
-
What Is The Square Root Of 122
Apr 02, 2025
-
The Unit Of Energy In Si Units Is
Apr 02, 2025
-
How To Find The Mean Of A Probability Distribution
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about Is The Square Root Of 4 Rational . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
