Is Square Root Of 4 A Rational Number
listenit
Apr 06, 2025 · 5 min read
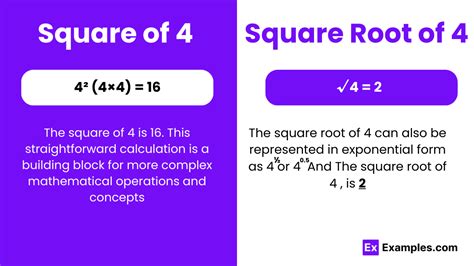
Table of Contents
Is the Square Root of 4 a Rational Number? A Deep Dive into Number Theory
The question, "Is the square root of 4 a rational number?" might seem deceptively simple. However, exploring this question allows us to delve into the fascinating world of number theory, clarifying the distinctions between rational and irrational numbers, and solidifying our understanding of fundamental mathematical concepts. This comprehensive guide will not only answer the question definitively but also explore the broader implications and related topics.
Understanding Rational and Irrational Numbers
Before we tackle the square root of 4, let's establish a firm grasp on the definitions of rational and irrational numbers.
Rational Numbers: The Fractions
A rational number is any number that can be expressed as a fraction p/q, where p and q are integers, and q is not equal to zero. This means it can be represented as a ratio of two whole numbers. Examples of rational numbers include:
- 1/2: One-half
- 3/4: Three-quarters
- -2/5: Negative two-fifths
- 5: (Can be expressed as 5/1)
- 0: (Can be expressed as 0/1)
- 0.75: (Can be expressed as 3/4)
- -2.333...: (Can be expressed as -7/3 – a recurring decimal is still rational)
Rational numbers, when expressed as decimals, either terminate (end) or repeat in a predictable pattern.
Irrational Numbers: Beyond Fractions
An irrational number cannot be expressed as a fraction of two integers. Their decimal representations neither terminate nor repeat. Famous examples include:
- π (pi): Approximately 3.14159..., the ratio of a circle's circumference to its diameter.
- e (Euler's number): Approximately 2.71828..., the base of the natural logarithm.
- √2 (the square root of 2): Approximately 1.41421..., the length of the diagonal of a unit square.
The decimal expansions of irrational numbers continue infinitely without ever settling into a repeating pattern.
Solving the Mystery: Is √4 Rational?
Now, let's address the central question: Is √4 a rational number?
The square root of a number is a value that, when multiplied by itself, gives the original number. In this case, we're looking for a number that, when multiplied by itself, equals 4.
That number is 2.
Since 2 can be expressed as the fraction 2/1 (where both 2 and 1 are integers, and the denominator is not zero), the square root of 4 (which is 2) is indeed a rational number.
Expanding Our Understanding: Perfect Squares and Rationality
The square root of 4 is a special case; 4 is a perfect square. A perfect square is a number that results from squaring an integer. Other perfect squares include 9 (3²), 16 (4²), 25 (5²), and so on. The square roots of all perfect squares are rational numbers because they can always be expressed as an integer (which is itself a rational number).
This understanding allows us to broaden our analysis: the square root of any perfect square is rational.
Exploring Further: Non-Perfect Squares and Irrationality
However, the square root of a non-perfect square is almost always irrational. For example, √2, √3, √5, √6, and so on, are all irrational numbers. Their decimal expansions continue infinitely without repeating. This is a crucial distinction in understanding the relationship between perfect squares and the rationality of their square roots.
Proof by Contradiction: A Rigorous Approach
We can rigorously prove that the square root of a non-perfect square is irrational using a method called proof by contradiction. Let's consider the case of √2:
-
Assumption: Let's assume, for the sake of contradiction, that √2 is rational. This means it can be expressed as a fraction p/q, where p and q are integers, q ≠ 0, and the fraction is simplified to its lowest terms (meaning p and q share no common factors other than 1).
-
Squaring: If √2 = p/q, then squaring both sides gives us 2 = p²/q².
-
Rearrangement: This can be rearranged to 2q² = p².
-
Even Numbers: This equation tells us that p² is an even number (because it's equal to 2 times another integer). If p² is even, then p must also be even (because the square of an odd number is always odd).
-
Substitution: Since p is even, we can express it as 2k, where k is another integer. Substituting this into the equation 2q² = p², we get 2q² = (2k)² = 4k².
-
Simplification: Dividing both sides by 2 gives us q² = 2k².
-
Contradiction: This means that q² is also an even number, and therefore q must also be even.
-
Conclusion: We've reached a contradiction. We initially assumed that p/q was in its simplest form (meaning p and q share no common factors). However, we've shown that both p and q are even, meaning they share a common factor of 2. This contradiction proves our initial assumption (that √2 is rational) must be false. Therefore, √2 is irrational.
This proof by contradiction can be adapted to prove the irrationality of the square roots of many other non-perfect squares.
Practical Applications and Significance
Understanding the difference between rational and irrational numbers isn't just an academic exercise. It has profound implications across various fields:
- Computer Science: Representing irrational numbers in computers requires approximations, which can introduce errors in calculations.
- Engineering: Precision in engineering designs often depends on understanding the limits of rational approximations of irrational numbers.
- Physics: Many physical constants, such as the speed of light, are often approximated using rational numbers for practical calculations.
- Mathematics: The study of rational and irrational numbers is fundamental to advanced mathematical concepts like calculus and real analysis.
Conclusion: Rationality and Beyond
The simple question of whether the square root of 4 is rational has led us on a journey through the fascinating world of number theory. We've established that √4, being equal to 2, is indeed rational. Furthermore, we've explored the broader context of rational and irrational numbers, learned how to distinguish between them, and even applied a rigorous proof method. Understanding this distinction is not merely an intellectual pursuit but is foundational to various fields of science, technology, and mathematics. The exploration highlights the elegance and interconnectedness of mathematical concepts, revealing the underlying principles that govern our understanding of numbers and their properties.
Latest Posts
Latest Posts
-
An Automatic Response To Some Stimulus Is Called
Apr 07, 2025
-
What Is 2 11 As A Decimal
Apr 07, 2025
-
Which Quadrilaterals Always Have Diagonals That Are Congruent
Apr 07, 2025
-
Elements In Groups 3 Through 12 Are Called
Apr 07, 2025
-
Only One Pair Of Parallel Sides
Apr 07, 2025
Related Post
Thank you for visiting our website which covers about Is Square Root Of 4 A Rational Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.