Is 35 Prime Or Composite Number
listenit
Mar 29, 2025 · 5 min read
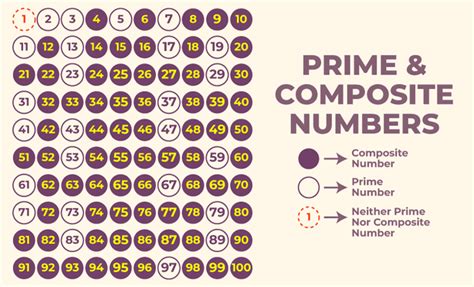
Table of Contents
Is 35 a Prime or Composite Number? A Deep Dive into Number Theory
Determining whether a number is prime or composite is a fundamental concept in number theory. This seemingly simple question—is 35 prime or composite?—opens the door to understanding the fascinating world of prime numbers, their properties, and their significance in mathematics and beyond. Let's explore this question thoroughly, delving into the definitions, methods for determining primality, and the broader implications of prime and composite numbers.
Understanding Prime and Composite Numbers
Before we classify 35, let's establish a clear understanding of the definitions:
-
Prime Number: A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, it's only divisible by 1 and itself without leaving a remainder. Examples include 2, 3, 5, 7, 11, and so on.
-
Composite Number: A composite number is a natural number greater than 1 that is not a prime number. This means it has at least one positive divisor other than 1 and itself. Examples include 4 (divisible by 1, 2, and 4), 6 (divisible by 1, 2, 3, and 6), 9, 10, and many more.
-
Neither Prime nor Composite: The number 1 is neither prime nor composite. It's a special case that stands alone in the classification of natural numbers.
Determining if 35 is Prime or Composite
Now, let's focus on the number 35. To determine if it's prime or composite, we need to check if it has any divisors other than 1 and itself. We can use several methods:
1. Trial Division
The most straightforward method is trial division. We systematically check if 35 is divisible by any prime number less than its square root (√35 ≈ 5.9). The prime numbers less than 5.9 are 2, 3, and 5.
- Divisibility by 2: 35 is not divisible by 2 (it's an odd number).
- Divisibility by 3: The sum of the digits of 35 (3 + 5 = 8) is not divisible by 3, so 35 is not divisible by 3.
- Divisibility by 5: 35 is divisible by 5 (35 / 5 = 7).
Since we found that 35 is divisible by 5 (and 7), it has divisors other than 1 and itself. Therefore, 35 is a composite number.
2. Factorization
Another method is to find the prime factorization of 35. This involves expressing the number as a product of its prime factors.
35 = 5 x 7
Since 35 can be expressed as a product of prime numbers (5 and 7), it confirms that 35 is a composite number. The presence of factors other than 1 and itself immediately disqualifies it from being a prime number.
The Significance of Prime and Composite Numbers
The classification of numbers as prime or composite is not merely an academic exercise. Prime numbers form the building blocks of all other integers through factorization. This fundamental property has far-reaching implications:
1. Cryptography
Prime numbers play a crucial role in modern cryptography. Many encryption algorithms, such as RSA, rely on the difficulty of factoring large composite numbers into their prime factors. The security of these systems depends on the computational infeasibility of factoring extremely large numbers, making them essential for secure online transactions, data protection, and more.
2. Number Theory Research
Prime numbers are a central subject of ongoing research in number theory. Many unsolved problems, such as the twin prime conjecture (infinitely many pairs of primes differing by 2) and Goldbach's conjecture (every even integer greater than 2 is the sum of two primes), continue to fascinate mathematicians and drive advancements in the field.
3. Applications in Computer Science
Besides cryptography, prime numbers find applications in various areas of computer science, including hash functions, pseudorandom number generators, and algorithm design. The properties of prime numbers are exploited to create efficient and secure algorithms used in diverse computational tasks.
4. Distribution of Prime Numbers
The way prime numbers are distributed among integers is a complex and fascinating topic. While there's no simple formula to predict the next prime number, the prime number theorem provides an approximation of the number of primes less than a given integer. This distribution pattern has implications for various mathematical and computational problems.
Further Exploration of Prime Numbers
Beyond the basic definition and methods of identification, the world of prime numbers offers many intriguing areas for exploration:
-
Mersenne Primes: These are prime numbers of the form 2<sup>p</sup> - 1, where p is also a prime number. The search for Mersenne primes continues to be a significant area of research in computational number theory.
-
Twin Primes: These are pairs of prime numbers that differ by 2 (e.g., 3 and 5, 11 and 13). The twin prime conjecture posits that there are infinitely many twin prime pairs, yet this remains an unsolved problem.
-
Prime Factorization Algorithms: Efficient algorithms for finding the prime factors of large composite numbers are crucial for cryptography and number theory. Algorithms like the general number field sieve are used to tackle this computationally challenging task.
-
Prime Number Sieves: These are algorithms used to efficiently generate lists of prime numbers within a given range. The Sieve of Eratosthenes is a classic example of a prime number sieve.
Conclusion
The question of whether 35 is prime or composite is a stepping stone to a deeper understanding of number theory. By applying simple methods like trial division or prime factorization, we can definitively conclude that 35 is a composite number. However, the broader significance of prime and composite numbers extends far beyond this simple classification. Their properties are fundamental to cryptography, computer science, and ongoing research in mathematics, making them a captivating subject for continued exploration and study. The seemingly simple act of classifying a number as prime or composite unveils a rich and complex world of mathematical patterns and implications, highlighting the enduring fascination with these fundamental building blocks of arithmetic.
Latest Posts
Latest Posts
-
What Percent Is A 26 Out Of 30
Mar 31, 2025
-
Which Is Denser Oceanic Or Continental Crust
Mar 31, 2025
-
Root 3 Divided By Root 3
Mar 31, 2025
-
The Shoulder Is Blank To The Elbow
Mar 31, 2025
-
Write 2 5 As A Decimal Number
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Is 35 Prime Or Composite Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
