Is 1 1/3 A Rational Number
listenit
Apr 14, 2025 · 5 min read
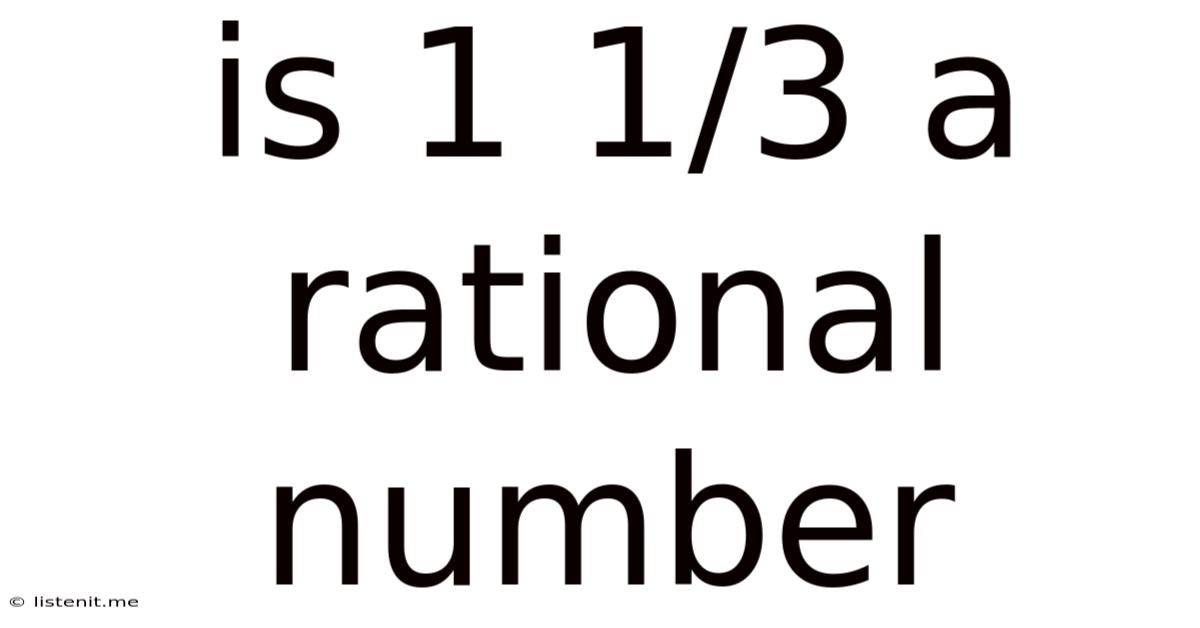
Table of Contents
Is 1 1/3 a Rational Number? A Deep Dive into Rational and Irrational Numbers
The question, "Is 1 1/3 a rational number?" might seem simple at first glance. However, understanding the answer requires a deeper understanding of what constitutes a rational number and how to identify them. This article will not only definitively answer this question but also explore the broader concepts of rational and irrational numbers, providing a comprehensive guide for anyone looking to strengthen their understanding of number systems.
What are Rational Numbers?
At its core, a rational number is any number that can be expressed as a fraction p/q, where both 'p' and 'q' are integers, and 'q' is not equal to zero. The key here is the ability to represent the number as a ratio of two whole numbers. This seemingly simple definition has profound implications for the types of numbers that fall under this category.
Let's break down the components:
-
Integers: These are whole numbers, including both positive and negative numbers (e.g., -3, -2, -1, 0, 1, 2, 3...). Decimals and fractions are not integers.
-
Fraction: A fraction represents a part of a whole. It is expressed as a numerator (the top number) divided by a denominator (the bottom number).
-
q ≠ 0: The denominator (q) cannot be zero. Division by zero is undefined in mathematics.
Examples of Rational Numbers:
- 1/2: This is a simple fraction representing one-half.
- -3/4: A negative fraction representing negative three-quarters.
- 5: The whole number 5 can be expressed as 5/1, fulfilling the definition of a rational number.
- 0.75: This decimal can be written as the fraction 3/4.
- -2.25: This decimal can be written as the fraction -9/4.
These examples illustrate the versatility of rational numbers. They encompass whole numbers, fractions, and terminating or repeating decimals.
What are Irrational Numbers?
In contrast to rational numbers, irrational numbers cannot be expressed as a fraction p/q, where p and q are integers and q is not zero. These numbers have decimal representations that are neither terminating (ending) nor repeating. Their decimal expansions continue infinitely without any discernible pattern.
Examples of Irrational Numbers:
- π (Pi): The ratio of a circle's circumference to its diameter, approximately 3.14159..., but its decimal representation goes on forever without repeating.
- √2 (Square root of 2): This number cannot be expressed as a simple fraction. Its decimal representation is approximately 1.414213..., also non-terminating and non-repeating.
- e (Euler's number): The base of the natural logarithm, approximately 2.71828..., an irrational number with an infinite, non-repeating decimal expansion.
Back to the Question: Is 1 1/3 a Rational Number?
Now, armed with a solid understanding of rational and irrational numbers, let's revisit the original question: Is 1 1/3 a rational number?
The answer is a resounding yes.
Here's why:
-
Convert to an Improper Fraction: The mixed number 1 1/3 can be easily converted into an improper fraction. To do this, we multiply the whole number (1) by the denominator (3) and add the numerator (1). This result (1*3 + 1 = 4) becomes the new numerator, while the denominator remains the same. Thus, 1 1/3 is equivalent to 4/3.
-
Integers as Components: Both the numerator (4) and the denominator (3) are integers.
-
Satisfies the Definition: Since 1 1/3 can be expressed as a fraction (4/3) where both the numerator and denominator are integers, and the denominator is not zero, it perfectly fits the definition of a rational number.
Further Exploration: Decimal Representation of Rational Numbers
All rational numbers have decimal representations that are either terminating or repeating. Let's examine this further in the context of 1 1/3:
To find the decimal representation of 4/3, we perform the division:
4 ÷ 3 = 1.3333...
Notice the repeating decimal: the digit '3' repeats infinitely. This repeating pattern is characteristic of many rational numbers. While the decimal representation is infinite, it's still considered rational because it follows a predictable, repeating pattern. This is a crucial distinction between rational and irrational numbers. Irrational numbers have infinite, non-repeating decimal expansions.
Distinguishing Rational and Irrational Numbers: A Practical Approach
Identifying whether a number is rational or irrational can sometimes be challenging. Here's a practical approach to help you determine the nature of a number:
-
Look for Fraction Representation: If the number can be expressed as a fraction p/q where p and q are integers and q ≠ 0, it's rational.
-
Examine the Decimal Representation: If the decimal representation terminates (ends) or repeats in a pattern, it's rational. If it's non-terminating and non-repeating, it's irrational.
-
Use a Calculator (with Caution): Calculators can help with decimal representation, but be aware that they often provide truncated (shortened) versions of decimals. A calculator might show π as 3.14159, but this is only an approximation. The true value of π is an infinitely long, non-repeating decimal.
The Importance of Understanding Rational and Irrational Numbers
The distinction between rational and irrational numbers is fundamental in mathematics. It forms the basis for many advanced mathematical concepts and has applications in various fields, including:
- Calculus: Understanding rational and irrational numbers is crucial for dealing with limits and continuity.
- Geometry: Irrational numbers often appear in geometric calculations, such as the diagonal of a square or the circumference of a circle.
- Computer Science: Representing and handling different number types efficiently is critical in programming.
- Physics: Many physical constants, such as the speed of light and gravitational constant, involve irrational numbers.
Conclusion: 1 1/3 is a Rational Number, and Understanding Why Matters
We've definitively established that 1 1/3 is a rational number because it can be expressed as the fraction 4/3, fulfilling the definition of a rational number. Beyond simply answering the initial question, this exploration has delved into the fundamental concepts of rational and irrational numbers, providing a robust understanding of their properties and significance in various mathematical and scientific fields. The ability to confidently distinguish between these number types is an essential skill for anyone pursuing further mathematical studies or working in fields that rely on mathematical principles. Remember, the key lies in the ability to represent a number as a ratio of two integers – that's the defining characteristic of a rational number.
Latest Posts
Latest Posts
-
How Does Acceleration Relate To Velocity
May 10, 2025
-
Water Evaporating Chemical Or Physical Change
May 10, 2025
-
Write The Chemical Formula For Barium Phosphide
May 10, 2025
-
How Do Nonmetals Tend To Form Bonds
May 10, 2025
-
If A Cell Is Placed In A Hypertonic Solution
May 10, 2025
Related Post
Thank you for visiting our website which covers about Is 1 1/3 A Rational Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.