Integral Of Cos X Sin X
listenit
May 10, 2025 · 5 min read
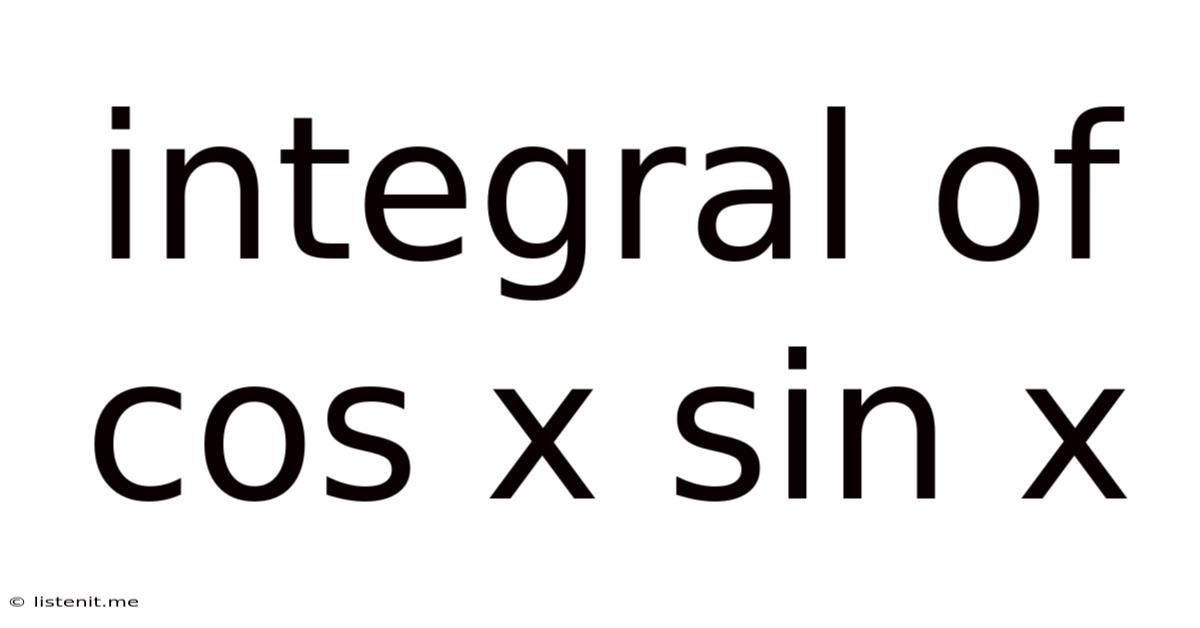
Table of Contents
The Integral of cos x sin x: A Comprehensive Guide
The integral of cos x sin x, a seemingly simple trigonometric expression, offers a rich landscape for exploring various integration techniques and their applications. This comprehensive guide will delve into different methods for solving this integral, highlighting their strengths and weaknesses, and expanding on related concepts within calculus. We'll also explore practical applications and contextualize the integral within broader mathematical frameworks.
Understanding the Problem: ∫cos x sin x dx
Our objective is to evaluate the indefinite integral: ∫cos x sin x dx. This integral represents the area under the curve of the function y = cos x sin x. Understanding the nature of this function is crucial before embarking on integration. The function is periodic, oscillating between positive and negative values, and crossing the x-axis at regular intervals. This periodicity will influence the characteristics of its antiderivative.
Method 1: Using u-Substitution
One of the most straightforward methods for solving this integral is u-substitution. This technique involves substituting a portion of the integrand with a new variable 'u' to simplify the expression.
Implementing u-Substitution
Let's choose u = sin x. Then, the derivative of u with respect to x is: du/dx = cos x, which implies du = cos x dx. Substituting these into our original integral, we get:
∫cos x sin x dx = ∫u du
This simplified integral is easily solvable:
∫u du = (1/2)u² + C
Where 'C' is the constant of integration. Substituting back for u, we obtain the final result:
∫cos x sin x dx = (1/2)sin²x + C
Verification through Differentiation
To verify our result, we can differentiate (1/2)sin²x + C with respect to x. Using the chain rule, we get:
d/dx [(1/2)sin²x + C] = (1/2) * 2sin x * cos x = sin x cos x
This confirms that our integration is correct.
Method 2: Using Trigonometric Identities
Another effective approach involves leveraging trigonometric identities to simplify the integrand before integration. Specifically, we can use the double-angle identity:
sin 2x = 2sin x cos x
This identity allows us to rewrite the integrand as:
cos x sin x = (1/2)sin 2x
Therefore, the integral becomes:
∫cos x sin x dx = ∫(1/2)sin 2x dx
Now, we can integrate directly:
∫(1/2)sin 2x dx = -(1/4)cos 2x + C
Comparing Results: (1/2)sin²x + C vs -(1/4)cos 2x + C
At first glance, these two results appear different. However, remember that trigonometric identities can relate seemingly disparate expressions. Using the double-angle identity cos 2x = 1 - 2sin²x, we can transform -(1/4)cos 2x + C into:
-(1/4)(1 - 2sin²x) + C = (1/2)sin²x - (1/4) + C
Since C represents an arbitrary constant, -(1/4) + C is also an arbitrary constant. Therefore, both results are equivalent, demonstrating the flexibility of different approaches.
Method 3: Integration by Parts
While less efficient for this specific integral, integration by parts provides a valuable alternative approach and illustrates its applicability in more complex scenarios. The formula for integration by parts is:
∫u dv = uv - ∫v du
Let's choose:
u = sin x => du = cos x dx dv = cos x dx => v = sin x
Applying integration by parts:
∫cos x sin x dx = sin x sin x - ∫sin x cos x dx
Notice that the integral on the right-hand side is the same as the original integral. Let's denote this integral as I:
I = sin²x - I
Solving for I:
2I = sin²x I = (1/2)sin²x + C
This method, although slightly more convoluted for this particular problem, reinforces the understanding of integration by parts and its potential utility in more challenging integrals involving products of trigonometric functions.
Extending the Concept: Definite Integrals
So far, we've focused on indefinite integrals. Let's consider definite integrals, which involve integrating over a specific interval [a, b]:
∫(from a to b) cos x sin x dx
Using either of the previously derived antiderivatives, we can evaluate this definite integral. For example, using (1/2)sin²x + C:
∫(from a to b) cos x sin x dx = = (1/2)[sin²b - sin²a]
This gives us the exact area under the curve of cos x sin x between points 'a' and 'b'.
Practical Applications
The integral of cos x sin x, while seemingly abstract, finds applications in various fields:
-
Physics: Oscillatory motion, such as that of a pendulum or a spring-mass system, often involves trigonometric functions. The integral can be used to calculate quantities like average displacement or energy over a cycle.
-
Signal Processing: Many signals in nature are periodic and can be represented using trigonometric functions. This integral is helpful in analyzing the properties of such signals.
-
Engineering: In areas like electrical engineering, the integral finds use in analyzing alternating current circuits where sinusoidal waveforms are prevalent.
-
Probability and Statistics: In probability density functions involving trigonometric functions, this integral might appear in calculations of expected values or probabilities.
Advanced Considerations and Related Integrals
The integral of cos x sin x serves as a foundation for understanding more complex trigonometric integrals. Consider the following extensions:
-
∫cos²x sin x dx: This can be solved using u-substitution, letting u = cos x.
-
∫cos x sin²x dx: Similarly, u-substitution with u = sin x solves this integral.
-
∫cos mx sin nx dx: This involves the product of sines and cosines with different frequencies (m and n). This integral often requires the use of trigonometric product-to-sum formulas.
-
Integrals involving higher powers of sine and cosine: These integrals often involve reduction formulas or recursive techniques to simplify the expression before integration.
Conclusion: Mastering the Integral of cos x sin x
The seemingly simple integral ∫cos x sin x dx opens doors to a deeper understanding of integration techniques and their diverse applications. Through u-substitution, trigonometric identities, and even integration by parts (though less efficient here), we've explored multiple paths to arrive at the same solution. This reinforces the idea that problem-solving in mathematics often benefits from exploring diverse strategies. The ability to solve this integral forms a critical stepping stone to tackling more complex trigonometric and calculus problems, showcasing its importance in both theoretical understanding and practical application across various scientific and engineering domains. Remember to always check your work by differentiating your result to ensure accuracy. Mastering this fundamental integral lays a strong foundation for further exploration in the fascinating world of calculus.
Latest Posts
Latest Posts
-
How To Do Absolute Value On Ti 84 Plus Ce
May 10, 2025
-
1 8 To The Power Of
May 10, 2025
-
How Many Right Angles Can A Quadrilateral Have
May 10, 2025
-
Determine The Isotope Symbol That Fits Each Description
May 10, 2025
-
Does Water Sink Or Float In Gas
May 10, 2025
Related Post
Thank you for visiting our website which covers about Integral Of Cos X Sin X . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.