How To Go From Pka To Ph
listenit
Apr 05, 2025 · 6 min read
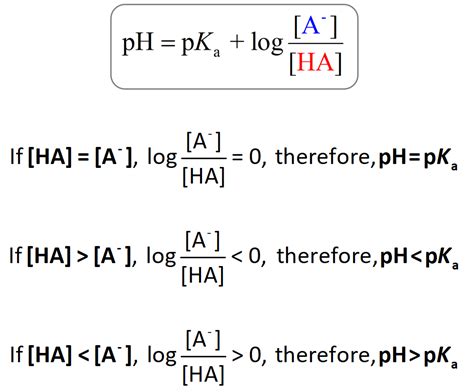
Table of Contents
How to Go From pKa to pH: A Comprehensive Guide
Understanding the relationship between pKa and pH is crucial in various scientific fields, including chemistry, biochemistry, and environmental science. While seemingly simple at first glance, mastering this concept unlocks a deeper understanding of acid-base equilibria and their implications. This comprehensive guide will delve into the intricacies of this relationship, providing you with the tools and knowledge to confidently navigate calculations and interpretations.
Understanding pKa and pH: The Fundamentals
Before diving into the conversion process, let's solidify our understanding of the fundamental concepts.
What is pKa?
pKa, or the acid dissociation constant, is a measure of the strength of an acid. It represents the negative logarithm (base 10) of the acid dissociation constant (Ka). A lower pKa value indicates a stronger acid, meaning it readily donates a proton (H⁺). Conversely, a higher pKa value signifies a weaker acid. The pKa is a characteristic property of a specific acid, remaining constant at a given temperature.
The equation for pKa is:
pKa = -log₁₀(Ka)
What is pH?
pH, or the potential of hydrogen, measures the acidity or alkalinity of a solution. It represents the negative logarithm (base 10) of the hydrogen ion concentration ([H⁺]) in moles per liter (M). A pH of 7 is considered neutral, while values below 7 indicate acidity and values above 7 indicate alkalinity.
The equation for pH is:
pH = -log₁₀([H⁺])
The Relationship Between pKa and pH
The relationship between pKa and pH becomes most apparent when considering the Henderson-Hasselbalch equation, a cornerstone of acid-base chemistry. This equation provides a powerful tool for calculating the pH of a buffer solution, a solution that resists changes in pH upon the addition of small amounts of acid or base.
The Henderson-Hasselbalch Equation: The Bridge Between pKa and pH
The Henderson-Hasselbalch equation is derived from the equilibrium expression for a weak acid dissociation and is expressed as:
pH = pKa + log₁₀([A⁻]/[HA])
Where:
- pH is the pH of the solution
- pKa is the pKa of the weak acid
- [A⁻] is the concentration of the conjugate base
- [HA] is the concentration of the weak acid
This equation highlights the crucial link between pKa and pH: the pH of a solution containing a weak acid and its conjugate base is determined by the pKa of the acid and the ratio of the concentrations of the conjugate base and the weak acid.
Calculating pH from pKa: Different Scenarios
The method of calculating pH from pKa varies depending on the context. Let's explore several common scenarios:
1. Calculating the pH of a buffer solution:
This is the most straightforward application of the Henderson-Hasselbalch equation. If you know the pKa of the weak acid and the concentrations of the weak acid and its conjugate base, you can directly calculate the pH.
Example:
A buffer solution contains 0.1 M acetic acid (CH₃COOH, pKa = 4.76) and 0.2 M sodium acetate (CH₃COONa). Calculate the pH of the solution.
Using the Henderson-Hasselbalch equation:
pH = pKa + log₁₀([CH₃COO⁻]/[CH₃COOH])
pH = 4.76 + log₁₀(0.2/0.1)
pH = 4.76 + log₁₀(2)
pH ≈ 4.76 + 0.30
pH ≈ 5.06
Therefore, the pH of the buffer solution is approximately 5.06.
2. Calculating the pH of a weak acid solution:
If you only have a weak acid and no conjugate base, you need to use the equilibrium expression and an ICE (Initial, Change, Equilibrium) table to solve for [H⁺] and then calculate the pH.
Example:
Calculate the pH of a 0.1 M solution of benzoic acid (C₆H₅COOH, pKa = 4.20).
First, find the Ka from the pKa:
Ka = 10⁻⁴·²⁰ ≈ 6.31 × 10⁻⁵
Then construct an ICE table:
| C₆H₅COOH | C₆H₅COO⁻ | H⁺ | |
|---|---|---|---|
| Initial (I) | 0.1 | 0 | 0 |
| Change (C) | -x | +x | +x |
| Equilibrium (E) | 0.1 - x | x | x |
Now, use the Ka expression:
Ka = [C₆H₅COO⁻][H⁺]/[C₆H₅COOH]
6.31 × 10⁻⁵ = (x)(x)/(0.1 - x)
Since Ka is small, we can approximate 0.1 - x ≈ 0.1:
6.31 × 10⁻⁵ ≈ x²/0.1
x² ≈ 6.31 × 10⁻⁶
x ≈ 2.51 × 10⁻³
Therefore, [H⁺] ≈ 2.51 × 10⁻³ M
pH = -log₁₀(2.51 × 10⁻³) ≈ 2.60
The pH of the 0.1 M benzoic acid solution is approximately 2.60.
3. Calculating the pH at different stages of a titration:
During a titration, the pH changes as the titrant is added. The Henderson-Hasselbalch equation is particularly useful for calculating the pH at various points, especially near the equivalence point. The calculations become more complex as the titration progresses and require consideration of the moles of acid and base present at each step. Detailed examples of titration calculations require a more extensive treatment and are beyond the scope of this simplified overview.
Beyond the Henderson-Hasselbalch Equation: Dealing with Polyprotic Acids
The Henderson-Hasselbalch equation is primarily applicable to monoprotic acids (acids that can donate only one proton). For polyprotic acids (acids that can donate more than one proton), the calculations become more involved as multiple equilibrium steps need to be considered. Each dissociation step will have its own pKa value, and the overall pH will depend on the relative contributions of each step. Iterative methods and advanced calculation techniques may be necessary for accurate pH determination in these scenarios.
Factors Affecting pH and pKa
Several factors can influence both pH and pKa values:
-
Temperature: Both pKa and pH are temperature-dependent. An increase in temperature usually leads to a decrease in pKa (stronger acidity) and can affect pH depending on the nature of the solution.
-
Ionic Strength: The presence of ions in a solution can affect the activity of the species involved in the acid-base equilibrium, leading to variations in both pKa and pH. The Debye-Hückel equation is often used to account for these ionic strength effects.
-
Solvent: The solvent used also significantly impacts pKa and pH. The dielectric constant of the solvent plays a crucial role in determining the strength of interactions between ions and molecules.
Applications of pKa and pH Calculations
The ability to accurately calculate pH from pKa and vice-versa has numerous practical applications across various fields:
-
Medicine: Understanding the pKa of drugs is essential for predicting their absorption, distribution, metabolism, and excretion (ADME) properties in the body. The pH of bodily fluids also plays a critical role in drug efficacy and toxicity.
-
Environmental Science: pH measurements are crucial for assessing water quality and monitoring environmental pollution. The pKa of various pollutants can help predict their behavior and fate in the environment.
-
Food Science: pH control is critical in food processing and preservation. Understanding the pKa of food components helps maintain desired acidity and prevents microbial growth.
-
Chemical Engineering: pH and pKa calculations are essential for controlling chemical reactions, designing efficient separation processes, and optimizing industrial processes.
Conclusion
The relationship between pKa and pH is a cornerstone of acid-base chemistry. Mastering this relationship, through the understanding and application of the Henderson-Hasselbalch equation and associated principles, provides a powerful tool for analyzing and predicting the behavior of acids and bases in various systems. While the calculations can become complex, especially for polyprotic acids or in solutions with significant ionic strength, a thorough understanding of the fundamentals is essential for successful applications across many scientific and technological disciplines. This guide provides a solid foundation for further exploration and advanced applications of these essential concepts. Remember to always consider the specific context and factors influencing your system for the most accurate and meaningful results.
Latest Posts
Latest Posts
-
2x 1 2x 3 X 2
Apr 06, 2025
-
Function Of A Fruit In A Plant
Apr 06, 2025
-
What Number Is 0 5 Of 8
Apr 06, 2025
-
What Is The Difference Between Balanced Forces And Action Reaction Forces
Apr 06, 2025
-
The Atomic Number Is Equal To The
Apr 06, 2025
Related Post
Thank you for visiting our website which covers about How To Go From Pka To Ph . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
