How To Combine Like Terms With Exponents
listenit
Mar 31, 2025 · 5 min read
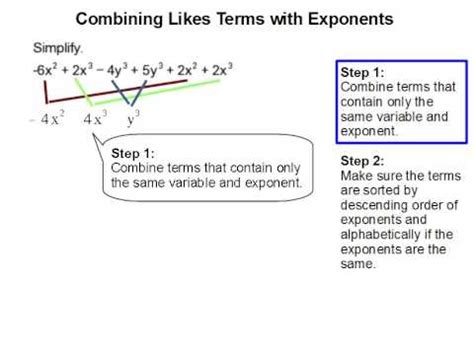
Table of Contents
How to Combine Like Terms with Exponents: A Comprehensive Guide
Combining like terms is a fundamental algebraic operation crucial for simplifying expressions and solving equations. While straightforward with simple terms, it becomes slightly more complex when exponents are involved. This comprehensive guide will delve into the intricacies of combining like terms with exponents, equipping you with the knowledge and skills to tackle even the most challenging algebraic expressions.
Understanding Like Terms and Exponents
Before we dive into combining like terms with exponents, let's refresh our understanding of these core concepts.
What are Like Terms?
Like terms are terms in an algebraic expression that have the same variables raised to the same powers. Consider these examples:
- Like terms: 3x², 5x², -2x² (same variable 'x', same exponent '2')
- Unlike terms: 3x², 5x, -2x³ (different exponents or variables)
The coefficients (the numbers in front of the variables) can be different, but the variables and their exponents must be identical for terms to be considered like terms.
Understanding Exponents
Exponents represent repeated multiplication. For example, x³ means x * x * x. The exponent indicates how many times the base (x) is multiplied by itself.
Understanding the rules of exponents is critical for combining like terms involving exponents. These rules include:
- Product Rule: xᵃ * xᵇ = x⁽ᵃ⁺ᵇ⁾ (When multiplying terms with the same base, add the exponents)
- Quotient Rule: xᵃ / xᵇ = x⁽ᵃ⁻ᵇ⁾ (When dividing terms with the same base, subtract the exponents)
- Power Rule: (xᵃ)ᵇ = x⁽ᵃ*ᵇ⁾ (When raising a power to a power, multiply the exponents)
- Zero Exponent Rule: x⁰ = 1 (Any non-zero base raised to the power of zero equals 1)
- Negative Exponent Rule: x⁻ᵃ = 1/xᵃ (A negative exponent indicates a reciprocal)
Combining Like Terms with Exponents: Step-by-Step Guide
Combining like terms with exponents involves applying the rules of exponents and the principles of combining like terms. Let's break down the process with examples.
Step 1: Identify Like Terms
The first step is to meticulously identify all like terms within the expression. Remember, like terms must have the same variables raised to the same powers.
Example 1: 3x² + 5x - 2x² + 7x + 4
Like terms: 3x², -2x² and 5x, 7x
Step 2: Group Like Terms
Once identified, group the like terms together. This makes the simplification process more organized and less error-prone.
Example 1 (continued): (3x² - 2x²) + (5x + 7x) + 4
Step 3: Combine Coefficients
Now, combine the coefficients of the like terms. This involves adding or subtracting the coefficients as indicated by the operation signs.
Example 1 (continued): (3 - 2)x² + (5 + 7)x + 4 = 1x² + 12x + 4 or simply x² + 12x + 4
Step 4: Simplify (Optional)
The final step is to simplify the expression by removing any unnecessary ones. In our example, 1x² simplifies to x².
Example 2: More complex scenario
Let's consider a more complex example involving multiple variables and higher exponents.
2x³y² + 5xy - 3x³y² + 2xy² + 7xy - 4x³y²
Step 1: Identify Like Terms:
- Like terms with x³y²: 2x³y², -3x³y², -4x³y²
- Like terms with xy: 5xy, 7xy
- Like terms with xy²: 2xy²
Step 2: Group Like Terms:
(2x³y² - 3x³y² - 4x³y²) + (5xy + 7xy) + 2xy²
Step 3: Combine Coefficients:
(2 - 3 - 4)x³y² + (5 + 7)xy + 2xy² = -5x³y² + 12xy + 2xy²
Step 4: Simplify:
The expression is already simplified. There are no more like terms to combine.
Dealing with Parentheses and Fractions
When dealing with parentheses or fractions, remember the order of operations (PEMDAS/BODMAS) and the rules for distributing or simplifying fractions.
Example 3: Expression with parentheses:
3x(2x² + 4x - 1) + 2x³
Step 1: Distribute:
First, distribute 3x into the parentheses: 6x³ + 12x² - 3x
Step 2: Identify Like Terms:
Like terms: 6x³, 2x³ and 12x² (no other like terms)
Step 3: Group and Combine:
(6x³ + 2x³) + 12x² - 3x = 8x³ + 12x² - 3x
Example 4: Expression with fractions:
(1/2)x² + (2/3)x² - (1/4)x
Step 1: Find Common Denominators:
To add or subtract fractions, you need a common denominator. For the x² terms, the least common multiple of 2 and 3 is 6.
Step 2: Rewrite with Common Denominators:
(3/6)x² + (4/6)x² - (1/4)x
Step 3: Combine Coefficients:
((3 + 4)/6)x² - (1/4)x = (7/6)x² - (1/4)x
Advanced Techniques and Considerations
Polynomials
Combining like terms is a fundamental skill when working with polynomials. Polynomials are algebraic expressions consisting of variables and coefficients, involving only the operations of addition, subtraction, multiplication, and non-negative integer exponents of variables. The techniques discussed above apply directly to simplifying polynomials.
Scientific Notation
When dealing with very large or very small numbers, scientific notation is often employed. Combining like terms in scientific notation requires careful attention to the exponents of 10. Remember to only combine the coefficients if the powers of 10 are the same.
Complex Numbers
Combining like terms also extends to complex numbers. Remember to separate the real and imaginary parts and combine like terms within each part.
Practical Applications
Combining like terms with exponents is a crucial skill across many areas of mathematics and science:
- Algebra: Simplifying expressions, solving equations, factoring
- Calculus: Finding derivatives and integrals
- Physics: Formulating and solving equations to model physical phenomena
- Engineering: Designing and analyzing systems
Mastering this skill significantly improves problem-solving capabilities and opens doors to more advanced mathematical concepts.
Conclusion
Combining like terms with exponents is a powerful tool in simplifying algebraic expressions and solving equations. By understanding the rules of exponents and systematically following the steps outlined in this guide, you can confidently tackle even the most complex expressions. Consistent practice is key to developing fluency and accuracy in this essential algebraic skill. Remember to review the rules of exponents regularly and to work through various examples to solidify your understanding. With dedication and practice, you’ll master this fundamental aspect of algebra.
Latest Posts
Latest Posts
-
Animals That Feed Exclusively On Plants Are Called
Apr 02, 2025
-
What Is The Equivalent Fraction Of 3 4
Apr 02, 2025
-
Least Common Multiple Of 12 And 4
Apr 02, 2025
-
What Does 2 1 2 Mean
Apr 02, 2025
-
One Goal Of Nativist Groups In The Late 1800s Was
Apr 02, 2025
Related Post
Thank you for visiting our website which covers about How To Combine Like Terms With Exponents . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
