How Many Radians In One Revolution
listenit
Mar 30, 2025 · 5 min read
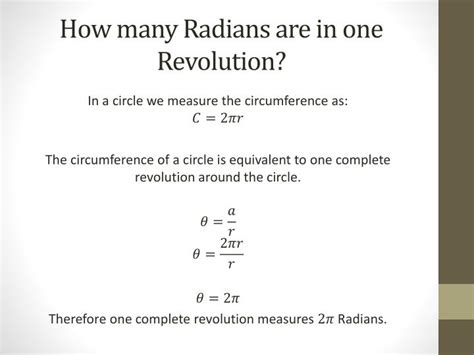
Table of Contents
How Many Radians in One Revolution? A Comprehensive Guide
Understanding radians is crucial for anyone working with circles, trigonometry, calculus, and various aspects of physics and engineering. While degrees are a familiar unit for measuring angles, radians offer a more natural and mathematically elegant approach. This comprehensive guide will delve into the concept of radians, explain how many radians constitute one revolution, and explore its significance in various applications.
What are Radians?
Radians are a unit of measurement for angles, based on the radius of a circle. Unlike degrees, which arbitrarily divide a circle into 360 parts, radians directly relate the angle to the arc length it subtends on the circle's circumference.
One radian is defined as the angle subtended at the center of a circle by an arc equal in length to the radius of the circle. Imagine a circle with radius r. If you draw an arc along the circumference of length r, the angle subtended at the center by this arc is exactly one radian.
Key takeaway: A radian is a ratio of arc length to radius.
The Relationship Between Radians and the Circle's Circumference
The circumference (C) of a circle is given by the formula: C = 2πr, where 'r' is the radius.
Since one radian corresponds to an arc length equal to the radius, we can determine how many radians are in a complete revolution. A complete revolution covers the entire circumference. Therefore, the number of radians in one revolution is the ratio of the circumference to the radius:
Number of radians = Circumference / Radius = (2πr) / r = 2π
Therefore, there are 2π radians in one revolution. This is a fundamental constant in mathematics and physics.
Why Radians are Preferred in Advanced Mathematics and Physics
The use of radians simplifies many mathematical formulas and relationships, making them more elegant and easier to work with. Here are some key reasons:
-
Simplifies Calculus: Derivatives and integrals of trigonometric functions are significantly simpler when using radians. For instance, the derivative of sin(x) is cos(x) only when x is measured in radians. Using degrees introduces cumbersome conversion factors that complicate calculations.
-
Natural Relationship with Arc Length: As mentioned earlier, radians directly relate the angle to the arc length. This simplifies formulas in physics and engineering related to circular motion, rotational mechanics, and wave phenomena.
-
Small Angle Approximation: For small angles, the sine and tangent of the angle (in radians) are approximately equal to the angle itself. This simplification is crucial in many physics applications, particularly in optics and mechanics.
-
Power Series Expansions: The Taylor and Maclaurin series expansions for trigonometric functions are much cleaner and simpler when using radians. These expansions are fundamental in numerical analysis and approximation techniques.
Converting Between Radians and Degrees
Since both radians and degrees measure angles, it's essential to be able to convert between the two units.
-
Radians to Degrees: To convert radians to degrees, multiply the radian measure by
180/π. -
Degrees to Radians: To convert degrees to radians, multiply the degree measure by
π/180.
For example:
-
Converting π/2 radians to degrees: (π/2) * (180/π) = 90 degrees
-
Converting 45 degrees to radians: 45 * (π/180) = π/4 radians
Radians in Different Contexts
Radians find widespread applications across various fields:
1. Trigonometry:
Radians are the natural unit for trigonometric functions in calculus and advanced mathematics. The relationships between trigonometric functions are most easily expressed using radians. For example, the unit circle, a fundamental concept in trigonometry, is best understood using radians.
2. Circular Motion and Rotational Mechanics:
In physics, radians are essential for describing angular velocity, angular acceleration, and torque. Angular velocity, for instance, is often measured in radians per second (rad/s). The use of radians simplifies the equations governing circular motion.
3. Wave Phenomena:
Radians are integral to understanding wave motion, including sound waves and light waves. The phase of a wave is often expressed in radians, and the angular frequency (ω) is commonly measured in radians per second.
4. Calculus and Analysis:
As discussed earlier, the use of radians significantly simplifies calculus, particularly when working with derivatives and integrals of trigonometric functions. This simplification extends to more advanced concepts like Fourier analysis and Laplace transforms.
5. Computer Graphics and Animation:
In computer graphics and animation, radians are often used to represent rotations and transformations. The use of radians ensures smooth and accurate rotations in 2D and 3D graphics.
Common Radian Measures and Their Equivalent Degrees
It's helpful to memorize some common radian measures and their equivalent degrees:
| Radians | Degrees |
|---|---|
| 0 | 0 |
| π/6 | 30 |
| π/4 | 45 |
| π/3 | 60 |
| π/2 | 90 |
| π | 180 |
| 3π/2 | 270 |
| 2π | 360 |
Beyond One Revolution: Multiple Revolutions
The concept of radians extends seamlessly to angles larger than one revolution. For instance, an angle of 4π radians represents two complete revolutions (4π = 2 * 2π). Similarly, 6π radians represent three revolutions, and so on. This allows for a consistent and unified representation of angles, regardless of their magnitude.
Conclusion: The Importance of Understanding Radians
Radians, while initially less intuitive than degrees, are fundamentally more significant in mathematics, physics, and engineering. Their inherent connection to the circle's geometry and their simplification of various mathematical formulas make them the preferred unit for advanced applications. Understanding the concept of 2π radians in one revolution is a cornerstone of grasping the elegance and power of this system of angular measurement. By mastering the conversion between radians and degrees and recognizing the applications of radians in various fields, you'll solidify your foundation in mathematics and its many applications.
Latest Posts
Latest Posts
-
Circumference Of A Circle With A Diameter Of 10
Apr 01, 2025
-
What Transition Metals Have A Fixed Charge
Apr 01, 2025
-
What Are Biotic Factors And Abiotic Factors
Apr 01, 2025
-
Common Multiples Of 4 And 9
Apr 01, 2025
-
How Many Quarts In 5 Gallon Bucket
Apr 01, 2025
Related Post
Thank you for visiting our website which covers about How Many Radians In One Revolution . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
