How Many 3/5 Are In 2
listenit
May 12, 2025 · 5 min read
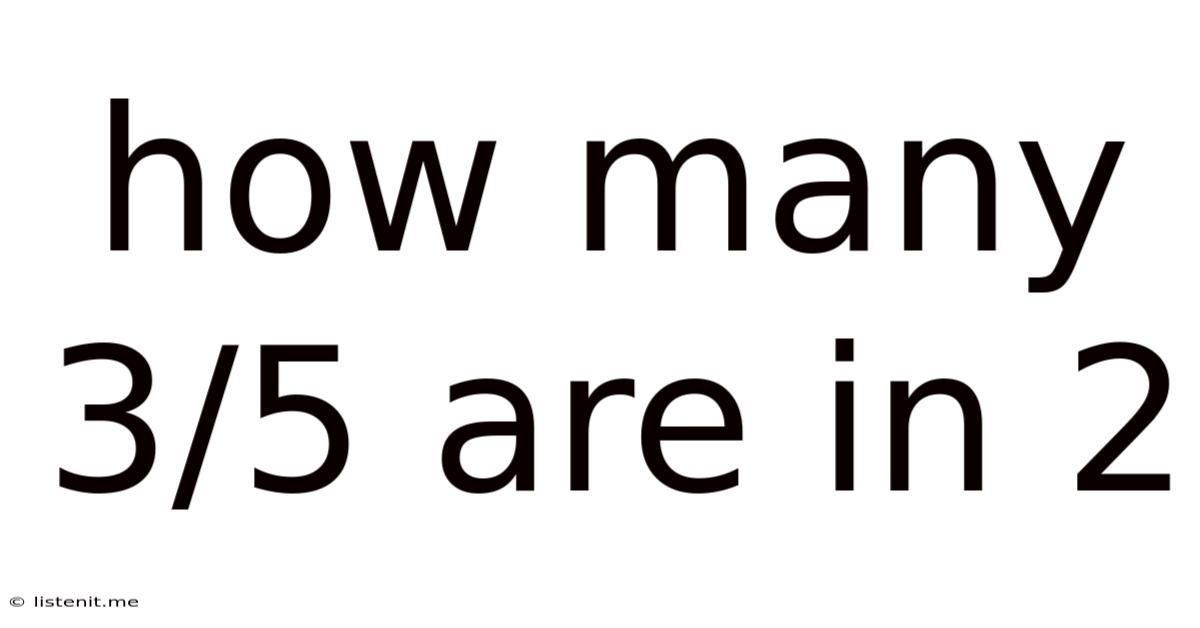
Table of Contents
How Many 3/5s Are in 2? A Deep Dive into Fraction Division
This seemingly simple question, "How many 3/5s are in 2?", opens the door to a deeper understanding of fraction division, a crucial concept in mathematics. While the answer might seem immediately obvious to some, exploring the different methods of solving this problem and understanding the underlying principles will solidify your grasp of fractional arithmetic and enhance your problem-solving skills. This comprehensive guide will dissect this question, providing multiple approaches, practical examples, and real-world applications.
Understanding the Problem: Visualizing Fractions
Before we delve into the calculations, let's visualize the problem. We're essentially asking how many times the fraction 3/5 fits into the whole number 2. Imagine you have two whole pizzas, and each slice represents 1/5 of a pizza. The fraction 3/5 represents three of these slices. The question becomes: how many sets of three slices (3/5) can you get from ten slices (the total in two pizzas)?
This visualization helps to intuitively grasp the problem and understand the logic behind the mathematical operations.
Method 1: Converting to Improper Fractions
One efficient method to solve this problem involves converting the whole number into an improper fraction and then dividing the fractions.
Step 1: Convert the Whole Number to an Improper Fraction
The whole number 2 can be represented as an improper fraction with the same denominator as 3/5. Since 3/5 has a denominator of 5, we can express 2 as 10/5 (because 10 divided by 5 equals 2).
Step 2: Divide the Fractions
Now, we have a division problem: (10/5) ÷ (3/5). Remember that dividing by a fraction is the same as multiplying by its reciprocal. The reciprocal of 3/5 is 5/3. Therefore, the equation becomes:
(10/5) x (5/3)
Step 3: Simplify and Solve
We can simplify this equation before multiplying: The 5 in the numerator and the 5 in the denominator cancel each other out. This leaves us with:
10/3
Step 4: Convert to a Mixed Number (Optional)
The improper fraction 10/3 can be converted into a mixed number. 3 goes into 10 three times with a remainder of 1. Therefore, 10/3 is equal to 3 1/3.
Therefore, there are 3 1/3 sets of 3/5 in 2.
Method 2: Using Decimal Equivalents
Another approach involves converting the fractions to decimals and then performing the division.
Step 1: Convert Fractions to Decimals
The fraction 3/5 is equivalent to 0.6 (because 3 divided by 5 equals 0.6). The whole number 2 remains 2.
Step 2: Perform Decimal Division
Now, divide 2 by 0.6:
2 ÷ 0.6 = 3.333...
Step 3: Interpret the Result
The result, 3.333..., indicates that there are approximately 3.33 sets of 3/5 in 2. This decimal result is equivalent to the mixed number 3 1/3 obtained in the previous method.
Method 3: Repeated Subtraction
This method provides a more intuitive understanding of the process. We repeatedly subtract 3/5 from 2 until we can no longer subtract without going into negative numbers.
Step 1: Repeated Subtraction
- 2 - 3/5 = 1 2/5
- 1 2/5 - 3/5 = 1 2/5 - 3/5 = 4/5
- 4/5 - 3/5 = 1/5
We have subtracted 3/5 three times. The remaining amount is 1/5.
Step 2: Interpret the Remainder
The fact that we have 1/5 left signifies that we didn't have another full 3/5 to subtract. We subtracted three full sets of 3/5, with 1/5 remaining. This demonstrates that there are 3 sets of 3/5 in 2, with a remaining fraction of 1/5. This 1/5 represents one-third of a full 3/5, confirming the answer as 3 1/3.
Real-World Applications: Why This Matters
Understanding fraction division isn't just an abstract mathematical exercise. It has numerous practical applications in everyday life and various professions:
-
Cooking and Baking: Scaling recipes up or down requires precise fraction calculations. If a recipe calls for 3/5 of a cup of flour and you want to double the recipe, you need to know how much flour you'll need (2 x 3/5 = 1 1/5 cups).
-
Construction and Engineering: Precise measurements are crucial in these fields. Calculating material quantities, determining the length of pipes, and many other tasks involve fraction division.
-
Finance and Budgeting: Managing finances effectively requires understanding fractions and percentages. Calculating interest, dividing expenses, or determining proportions of savings involves fractional arithmetic.
-
Sewing and Tailoring: Cutting fabric accurately for clothing or other projects necessitates precise calculations involving fractions of inches or centimeters.
-
Data Analysis: Many data analysis tasks involve calculating proportions and averages, which often involve working with fractions and percentages.
Beyond the Basics: Extending Your Understanding
This exploration of how many 3/5s are in 2 serves as a stepping stone to a deeper understanding of fraction manipulation. Consider these extensions to enhance your mathematical skills:
-
Working with More Complex Fractions: Practice solving similar problems with more complex fractions and mixed numbers. The same principles apply, but the calculations might be slightly more involved.
-
Solving Word Problems: Practice applying these concepts to word problems to hone your problem-solving skills. Word problems often require translating real-world scenarios into mathematical equations.
-
Exploring Different Methods: Experiment with different methods of solving these types of problems. Familiarity with various approaches broadens your problem-solving toolkit.
-
Mastering Fraction Operations: Strengthen your understanding of all fraction operations—addition, subtraction, multiplication, and division—to handle a wider range of mathematical challenges.
Conclusion: Mastering Fraction Division
This detailed exploration has demonstrated multiple methods for solving the question, "How many 3/5s are in 2?" The answer, 3 1/3, highlights the importance of mastering fraction division. This skill is not only crucial for academic success but also essential for navigating various real-world situations. By understanding the different approaches and practicing regularly, you can build confidence and proficiency in handling fractions and enhance your overall mathematical capabilities. Remember to visualize the problem, choose the method you find most efficient, and always check your work to ensure accuracy. The journey to mastering fractions is a rewarding one, empowering you with the skills to tackle a wide range of mathematical challenges.
Latest Posts
Latest Posts
-
How Are The Elements In The Modern Periodic Table Arranged
May 12, 2025
-
What Is The Decimal Of 13 20
May 12, 2025
-
Difference Between Atomic And Molar Mass
May 12, 2025
-
When An Iron Ring Is Heated The Hole Becomes
May 12, 2025
-
How Many S Sp2 Sigma Bonds Are In The Following Compound
May 12, 2025
Related Post
Thank you for visiting our website which covers about How Many 3/5 Are In 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.