How Do You Find The Perimeter Of An Equilateral Triangle
listenit
Apr 02, 2025 · 6 min read
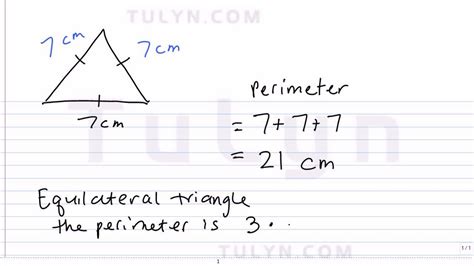
Table of Contents
How Do You Find the Perimeter of an Equilateral Triangle? A Comprehensive Guide
Understanding how to calculate the perimeter of an equilateral triangle is a fundamental concept in geometry. This guide provides a comprehensive explanation, covering various methods and delving into the properties of equilateral triangles that make these calculations straightforward. We'll explore different scenarios and provide practical examples to solidify your understanding. By the end, you'll be able to confidently determine the perimeter of any equilateral triangle, regardless of the information provided.
Understanding Equilateral Triangles
Before diving into perimeter calculations, let's establish a firm understanding of what defines an equilateral triangle. An equilateral triangle is a polygon with three equal sides and three equal angles. Each interior angle measures 60 degrees. This consistent property is the key to simplifying perimeter calculations. Unlike other triangles, where you might need to know the length of each side individually, the equilateral triangle's uniformity allows for efficient calculations using minimal information.
Method 1: Using the Length of One Side
This is the most straightforward method. Since all sides of an equilateral triangle are equal in length, you only need to know the length of one side to calculate the perimeter.
Formula: Perimeter = 3 * side length
Example:
Let's say we have an equilateral triangle with one side measuring 5 cm. To find the perimeter, we simply multiply the side length by 3:
Perimeter = 3 * 5 cm = 15 cm
Therefore, the perimeter of this equilateral triangle is 15 cm.
This method is incredibly efficient and is applicable whenever the length of a single side is known. Remember, this simplicity stems directly from the defining characteristic of an equilateral triangle: equal sides.
Method 2: Using the Altitude (Height)
While less direct, you can also calculate the perimeter using the altitude (height) of the equilateral triangle. This method requires a bit more mathematical manipulation but demonstrates the interconnectivity of different triangle properties.
Understanding Altitude: The altitude of a triangle is a line segment drawn from a vertex perpendicular to the opposite side. In an equilateral triangle, the altitude also bisects the base, creating two congruent 30-60-90 right-angled triangles.
Formula Derivation:
-
30-60-90 Triangle Properties: In a 30-60-90 triangle, the sides are in a ratio of 1:√3:2. Let's denote the shorter leg (opposite the 30-degree angle) as 'x'. Then the hypotenuse (the side of the equilateral triangle) is '2x', and the longer leg (the altitude) is 'x√3'.
-
Relating Altitude and Side Length: From the 30-60-90 triangle, we can express the side length (hypotenuse) in terms of the altitude: side length = (2/√3) * altitude
-
Calculating Perimeter: Once we have the side length, we can use the standard perimeter formula: Perimeter = 3 * side length = 3 * [(2/√3) * altitude]
Example:
Suppose the altitude of an equilateral triangle is 6 cm. Let's calculate the perimeter:
-
Side length = (2/√3) * 6 cm ≈ 6.93 cm
-
Perimeter = 3 * 6.93 cm ≈ 20.79 cm
The perimeter of the equilateral triangle is approximately 20.79 cm.
Method 3: Using the Area and Altitude
The area of an equilateral triangle can also be used to determine its perimeter, providing yet another avenue for calculation. This method demonstrates the relationship between area and perimeter within the context of an equilateral triangle.
Formula:
The area (A) of an equilateral triangle is given by: A = (√3/4) * side length²
We can rearrange this formula to solve for the side length: side length = √[(4A)/√3]
Once you have the side length, you can calculate the perimeter using the standard formula: Perimeter = 3 * side length
Example:
Let's assume the area of an equilateral triangle is 25 cm².
-
Side length = √[(4 * 25 cm²)/√3] ≈ 7.64 cm
-
Perimeter = 3 * 7.64 cm ≈ 22.92 cm
Therefore, the perimeter of this equilateral triangle is approximately 22.92 cm. Note that the accuracy depends on the accuracy of the area measurement.
Method 4: Using the Inradius (Incircle Radius)
The inradius is the radius of the inscribed circle within an equilateral triangle. This method offers an alternative approach, highlighting the geometrical properties related to inscribed circles.
Formula Derivation:
-
Relationship between Inradius (r) and Side Length (s): The inradius of an equilateral triangle is related to its side length by the formula: r = (s√3)/6
-
Solving for Side Length: Rearranging the formula, we get: s = (6r)/√3
-
Calculating Perimeter: Substitute the value of 's' into the standard perimeter formula: Perimeter = 3 * [(6r)/√3]
Example:
If the inradius of an equilateral triangle is 2 cm, let's calculate the perimeter:
-
Side length = (6 * 2 cm)/√3 ≈ 6.93 cm
-
Perimeter = 3 * 6.93 cm ≈ 20.79 cm
The perimeter of the equilateral triangle is approximately 20.79 cm.
Method 5: Using the Circumradius (Circumcircle Radius)
The circumradius is the radius of the circumscribed circle around the equilateral triangle. This method provides another perspective, showing the connection between the circumcircle and the triangle's perimeter.
Formula Derivation:
-
Relationship between Circumradius (R) and Side Length (s): The circumradius of an equilateral triangle is related to its side length by the formula: R = (s√3)/3
-
Solving for Side Length: Rearranging the formula gives: s = (3R)/√3
-
Calculating Perimeter: Substitute the value of 's' into the standard perimeter formula: Perimeter = 3 * [(3R)/√3]
Example:
If the circumradius of an equilateral triangle is 4 cm, let's calculate the perimeter:
-
Side length = (3 * 4 cm)/√3 ≈ 6.93 cm
-
Perimeter = 3 * 6.93 cm ≈ 20.79 cm
The perimeter of the equilateral triangle is approximately 20.79 cm.
Solving Real-World Problems
Understanding these methods allows you to tackle various real-world scenarios. For example, you might need to calculate the amount of fencing required to enclose a triangular garden (assuming it's equilateral), or determine the total length of material needed to construct a triangular frame. The principles remain the same – identify the known information (side length, altitude, area, inradius, or circumradius), apply the relevant formula, and solve for the perimeter.
Conclusion: Mastering Equilateral Triangle Perimeter Calculations
Calculating the perimeter of an equilateral triangle is a straightforward process, thanks to the consistent properties of this geometric shape. This guide has presented five different methods, demonstrating the interconnectivity of various triangle properties. Whether you're dealing with side lengths, altitudes, area, inradius, or circumradius, you now possess the knowledge and tools to efficiently solve for the perimeter. Remember to choose the method that best suits the information provided in your specific problem. By understanding these calculations, you've taken a significant step in mastering fundamental geometric concepts.
Latest Posts
Latest Posts
-
What Is 6 8 As A Decimal
Apr 03, 2025
-
What Is 0 1 Repeating As A Fraction
Apr 03, 2025
-
What Percent Of 18 Is 27
Apr 03, 2025
-
How Many Ounces Are In A 1 4 Pound
Apr 03, 2025
-
What Percent Is 13 Of 16
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about How Do You Find The Perimeter Of An Equilateral Triangle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
