Half Of 5 8 In Fraction
listenit
May 24, 2025 · 4 min read
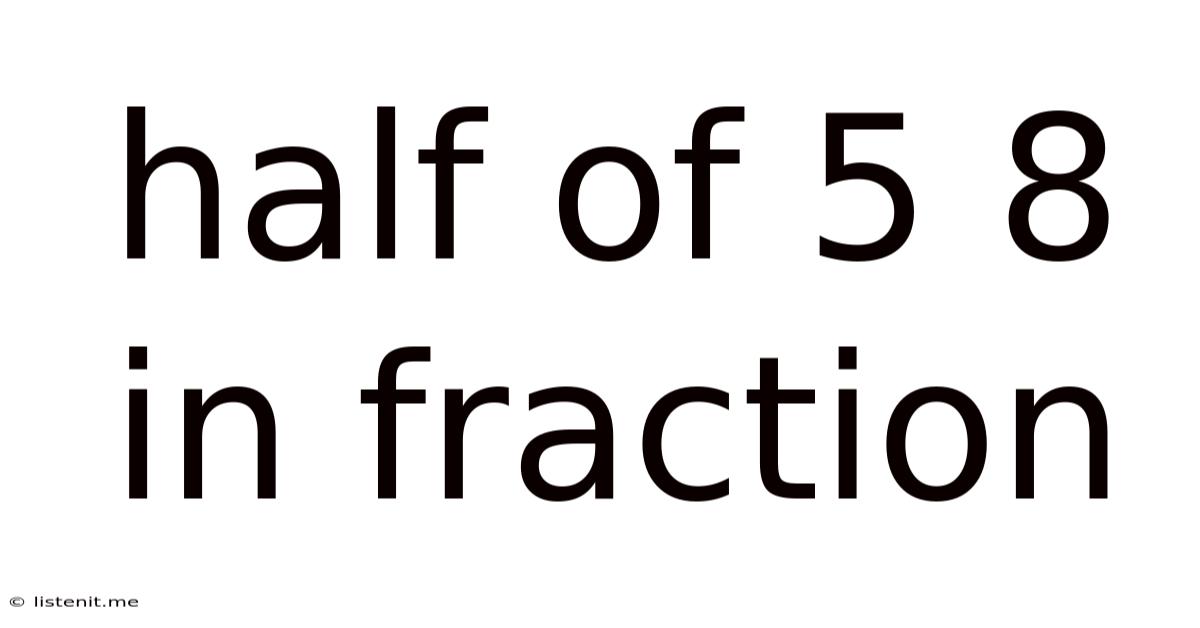
Table of Contents
Half of 5/8: A Comprehensive Guide to Fraction Division
Understanding fractions is a fundamental skill in mathematics, impacting various aspects of life from cooking and construction to advanced scientific calculations. This comprehensive guide delves into the process of finding half of 5/8, exploring the underlying concepts, providing step-by-step solutions, and offering practical examples to solidify your understanding. We'll also discuss related fraction concepts to broaden your mathematical knowledge.
What does "half of 5/8" mean?
The phrase "half of 5/8" essentially translates to finding one-half (1/2) of the fraction 5/8. In mathematical terms, this means we need to perform the operation of multiplication: (1/2) * (5/8). This is because "of" in this context signifies multiplication.
Step-by-Step Calculation: Finding Half of 5/8
Let's break down the calculation into easily digestible steps:
Step 1: Write the expression.
The problem is represented mathematically as: (1/2) × (5/8)
Step 2: Multiply the numerators.
The numerators are the top numbers in each fraction. Multiplying them together gives us: 1 × 5 = 5
Step 3: Multiply the denominators.
The denominators are the bottom numbers in each fraction. Multiplying them gives us: 2 × 8 = 16
Step 4: Form the new fraction.
Combine the results from steps 2 and 3 to create a new fraction: 5/16
Therefore, half of 5/8 is 5/16.
Visualizing the Solution
Imagine a pizza cut into 8 equal slices. 5/8 represents 5 of those slices. To find half of 5/8, we're essentially dividing those 5 slices in half. This results in 5 smaller pieces, each representing 1/16 of the original pizza. This visual representation helps solidify the concept that half of 5/8 is indeed 5/16.
Simplifying Fractions: A Necessary Skill
While 5/16 is already in its simplest form (meaning the numerator and denominator have no common factors other than 1), understanding fraction simplification is crucial for solving more complex problems. A fraction is simplified by finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by that number. For example, if we had the fraction 10/20, the GCD is 10, so simplifying would give us 1/2.
Real-World Applications of Fraction Division
Understanding fraction division isn't just an academic exercise; it's a practical skill with numerous real-world applications:
- Cooking: Recipes often require fractions. If a recipe calls for 5/8 cup of flour, and you want to halve the recipe, you need to find half of 5/8.
- Construction: Precise measurements are essential in construction. Dividing lengths and quantities often involves fractions.
- Sewing: Patterns and fabric cutting frequently use fractional measurements.
- Finance: Calculating portions of budgets or investments involves fractional calculations.
Expanding on Fraction Concepts: Beyond Half of 5/8
Let's explore related fraction concepts to further enhance your understanding:
Adding and Subtracting Fractions:
To add or subtract fractions, they must have a common denominator. If they don't, you need to find the least common multiple (LCM) of the denominators and convert the fractions accordingly. For example:
1/2 + 1/4 = 2/4 + 1/4 = 3/4
Multiplying Fractions:
Multiplying fractions is straightforward: multiply the numerators together and the denominators together. Simplifying the result is often necessary. For example:
(2/3) × (3/4) = (2 × 3) / (3 × 4) = 6/12 = 1/2
Dividing Fractions:
Dividing fractions involves inverting the second fraction (the divisor) and then multiplying. For example:
(1/2) ÷ (1/4) = (1/2) × (4/1) = 4/2 = 2
Converting Fractions to Decimals and Percentages:
Fractions can be converted to decimals by dividing the numerator by the denominator. To convert a decimal to a percentage, multiply by 100. For example:
5/8 = 0.625 = 62.5%
Solving More Complex Fraction Problems
Let's look at some more advanced problems involving fractions:
Problem 1: What is 3/4 of 5/8?
Solution: (3/4) × (5/8) = (3 × 5) / (4 × 8) = 15/32
Problem 2: What is 5/8 divided by 1/4?
Solution: (5/8) ÷ (1/4) = (5/8) × (4/1) = 20/8 = 5/2 = 2 1/2
Problem 3: What is the sum of 1/2, 1/4, and 5/8?
Solution: Find a common denominator (8): 4/8 + 2/8 + 5/8 = 11/8 = 1 3/8
Troubleshooting Common Fraction Mistakes
Many common mistakes arise when working with fractions. Here are some to watch out for:
- Forgetting to find a common denominator when adding or subtracting: Remember, you cannot directly add or subtract fractions with different denominators.
- Incorrectly multiplying or dividing numerators and denominators: Always multiply numerators together and denominators together when multiplying fractions. When dividing, remember to invert the second fraction.
- Not simplifying the result: Always simplify your answer to its lowest terms.
Conclusion: Mastering Fractions for Success
Understanding fractions is a cornerstone of mathematical proficiency. This guide has provided a comprehensive exploration of finding half of 5/8, highlighting the steps involved, and offering practical applications and related concepts. By mastering these fundamental skills and avoiding common pitfalls, you'll build a solid foundation for tackling more complex mathematical problems and successfully applying fractions in various real-world scenarios. Remember practice is key – the more you work with fractions, the more confident and proficient you'll become.
Latest Posts
Latest Posts
-
Greatest Common Factor 16 And 36
May 24, 2025
-
Least Common Multiple 3 5 7
May 24, 2025
-
What Is 2 3 Divided By 8
May 24, 2025
-
20 Out Of 21 As A Percentage
May 24, 2025
-
If Your 48 What Year Was You Born
May 24, 2025
Related Post
Thank you for visiting our website which covers about Half Of 5 8 In Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.