Half Of 5/8 As A Fraction
listenit
May 24, 2025 · 4 min read
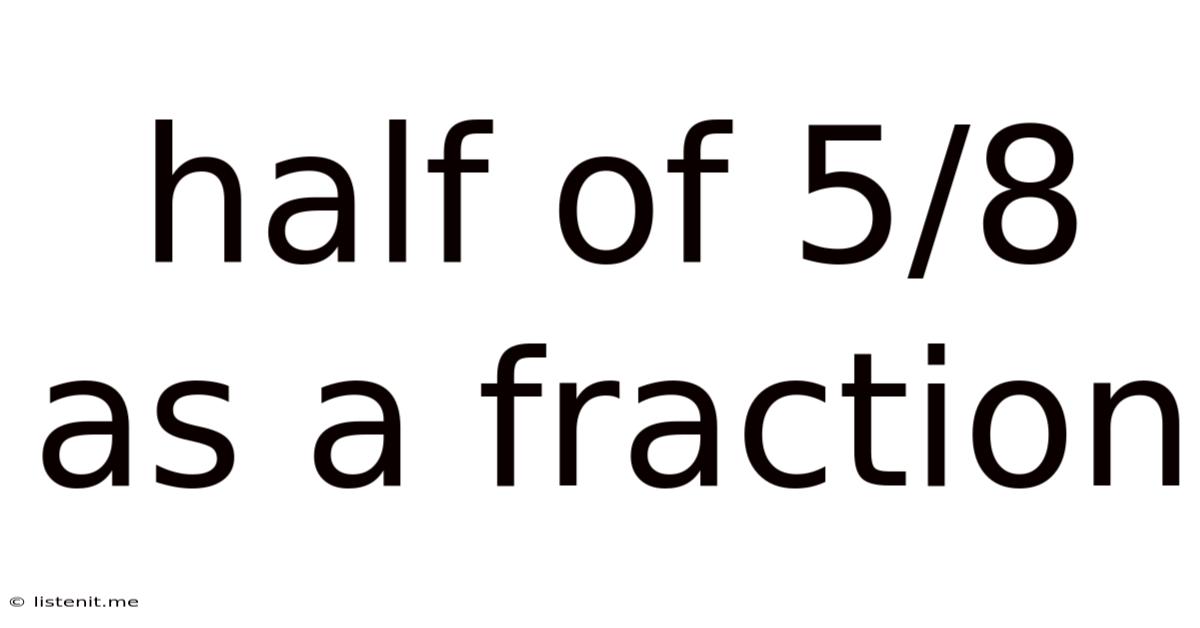
Table of Contents
Half of 5/8 as a Fraction: A Comprehensive Guide
Finding half of a fraction might seem like a simple task, but understanding the underlying principles is crucial for mastering more complex mathematical concepts. This comprehensive guide will delve into calculating half of 5/8 as a fraction, exploring various methods and highlighting the importance of simplifying fractions to their lowest terms. We'll also touch upon practical applications and explore related fraction problems. This detailed explanation will ensure you not only understand this specific problem but also build a stronger foundation in fraction arithmetic.
Understanding Fractions: A Quick Refresher
Before we dive into calculating half of 5/8, let's refresh our understanding of fractions. A fraction represents a part of a whole. It's composed of two main parts:
- Numerator: The top number, indicating the number of parts you have.
- Denominator: The bottom number, indicating the total number of equal parts the whole is divided into.
For example, in the fraction 5/8, 5 is the numerator and 8 is the denominator. This means we have 5 parts out of a total of 8 equal parts.
Method 1: Multiplying by 1/2
The most straightforward method to find half of a fraction is to multiply the fraction by 1/2. This is because multiplying by 1/2 is equivalent to dividing by 2.
Step 1: Set up the multiplication:
We want to find half of 5/8, so we write this as:
(1/2) * (5/8)
Step 2: Multiply the numerators:
Multiply the numerators together: 1 * 5 = 5
Step 3: Multiply the denominators:
Multiply the denominators together: 2 * 8 = 16
Step 4: Write the result as a fraction:
The result is 5/16. Therefore, half of 5/8 is 5/16.
Method 2: Dividing by 2
Another approach is to directly divide the fraction by 2. This involves dividing the numerator by 2. However, this method only works if the numerator is an even number. In cases where the numerator is odd, you'll need to use the multiplication method described above, or convert to a decimal, find half and then convert back to a fraction.
In this case, since the numerator (5) is odd, this method isn't directly applicable.
Simplifying Fractions
It's crucial to always simplify fractions to their lowest terms. This means finding the greatest common divisor (GCD) of the numerator and denominator and dividing both by it. The GCD is the largest number that divides both the numerator and denominator without leaving a remainder.
In our case, the fraction 5/16 is already in its simplest form because 5 and 16 share no common divisors other than 1.
Practical Applications
Understanding fractions and the ability to perform calculations like finding half of a fraction are essential in various real-world scenarios:
-
Cooking and Baking: Recipes often require fractions of ingredients. Knowing how to calculate half of a fraction is crucial for adjusting recipes to suit different serving sizes. For example, if a recipe calls for 5/8 of a cup of flour, and you want to halve the recipe, you'll need to calculate half of 5/8.
-
Construction and Engineering: Many construction and engineering projects rely on precise measurements. Fractions are frequently used in blueprints and calculations, and the ability to manipulate fractions accurately is vital for achieving the desired results.
-
Data Analysis: In data analysis, fractions are often used to represent proportions and percentages. Understanding fraction manipulation is key to interpreting data correctly.
-
Finance: Fractions are used extensively in financial calculations, including interest rates, stock prices, and bond yields.
Related Fraction Problems
Let's explore some related problems to further solidify your understanding of fraction manipulation:
1. Finding a third of 5/8:
To find a third of 5/8, we multiply 5/8 by 1/3:
(1/3) * (5/8) = 5/24
2. Finding double of 5/8:
To find double of 5/8, we multiply 5/8 by 2:
2 * (5/8) = 10/8 = 5/4 (Simplified)
3. Adding 5/8 and 1/4:
To add 5/8 and 1/4, we first need a common denominator:
5/8 + 1/4 = 5/8 + 2/8 = 7/8
4. Subtracting 1/2 from 5/8:
To subtract 1/2 from 5/8, we again need a common denominator:
5/8 - 1/2 = 5/8 - 4/8 = 1/8
Mastering Fractions: Beyond the Basics
Understanding half of 5/8 is just one step in mastering fraction arithmetic. Proficiency in fraction manipulation requires consistent practice and a solid grasp of fundamental concepts. By understanding the underlying principles of numerators, denominators, simplification, and the different methods of calculation, you'll be well-equipped to tackle more complex fraction problems. Remember to always check your work and simplify your answers to their lowest terms for accuracy and clarity. Consistent practice and application will build your confidence and expertise in this crucial mathematical area. Further exploration of topics like improper fractions, mixed numbers, and operations with mixed numbers will solidify your understanding even further. Don't be afraid to explore different methods and find the approach that best suits your learning style. The more you practice, the more intuitive these calculations will become.
Latest Posts
Latest Posts
-
3 1 7 As An Improper Fraction
May 25, 2025
-
What Is The Square Root Of 1 Million
May 25, 2025
-
What Is 3 8 Of 16
May 25, 2025
-
How To Calculate Current In Resistor
May 25, 2025
-
How Much Is A Car After Taxes
May 25, 2025
Related Post
Thank you for visiting our website which covers about Half Of 5/8 As A Fraction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.