Determine The Rate Law For The Overall Reaction
listenit
Mar 29, 2025 · 6 min read
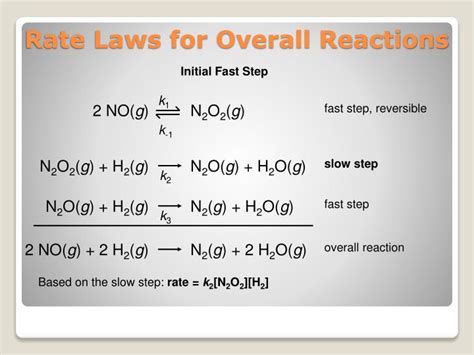
Table of Contents
Determining the Rate Law for the Overall Reaction: A Comprehensive Guide
Determining the rate law for a chemical reaction is crucial in understanding its mechanism and predicting its behavior under different conditions. The rate law expresses the relationship between the reaction rate and the concentrations of the reactants. Unlike stoichiometry, which is derived from the balanced chemical equation, the rate law is determined experimentally. This article will provide a comprehensive guide on how to determine the rate law for an overall reaction, covering various methods and considerations.
Understanding Rate Laws and Reaction Orders
Before diving into the methods, let's clarify some fundamental concepts. The rate law generally takes the form:
Rate = k[A]<sup>m</sup>[B]<sup>n</sup>...
Where:
- Rate: Represents the speed at which the reaction proceeds, typically expressed as the change in concentration of a reactant or product per unit time (e.g., M/s).
- k: Is the rate constant, a proportionality constant specific to the reaction at a given temperature. It reflects the intrinsic reactivity of the reactants.
- [A], [B], ...: Represent the molar concentrations of the reactants.
- m, n, ...: Are the reaction orders with respect to each reactant. These are exponents that are not necessarily equal to the stoichiometric coefficients in the balanced chemical equation. They must be determined experimentally.
The overall reaction order is the sum of the individual reaction orders (m + n + ...). For instance, if m = 1 and n = 2, the reaction is first order with respect to A, second order with respect to B, and third order overall.
Experimental Methods for Determining Rate Laws
Several experimental techniques are employed to determine rate laws. The most common are:
1. Method of Initial Rates
This is the most straightforward method. It involves conducting a series of experiments where the initial concentrations of reactants are varied while keeping other factors (temperature, pressure, etc.) constant. By comparing the initial rates, we can deduce the reaction orders.
Steps:
- Conduct multiple experiments: Vary the initial concentration of one reactant at a time, keeping the others constant. Measure the initial rate of the reaction for each experiment.
- Analyze the data: Compare the rates obtained. If doubling the concentration of a reactant doubles the rate, the reaction is first order with respect to that reactant (rate is directly proportional to concentration). If doubling the concentration quadruples the rate, it's second order (rate is proportional to the square of the concentration). No change in rate suggests zero order (rate is independent of concentration).
- Determine the rate constant (k): Once the reaction orders are known, substitute the data from one of the experiments into the rate law equation to solve for k.
Example:
Let's consider a reaction: A + B → Products. The following data is obtained:
| Experiment | [A] (M) | [B] (M) | Initial Rate (M/s) |
|---|---|---|---|
| 1 | 0.1 | 0.1 | 0.005 |
| 2 | 0.2 | 0.1 | 0.020 |
| 3 | 0.1 | 0.2 | 0.010 |
Analyzing the data:
- Comparing Experiments 1 and 2 (doubling [A]): The rate quadruples, indicating the reaction is second order with respect to A.
- Comparing Experiments 1 and 3 (doubling [B]): The rate doubles, indicating the reaction is first order with respect to B.
Therefore, the rate law is: Rate = k[A]<sup>2</sup>[B]<sup>1</sup>
Substitute the data from Experiment 1 to find k:
0.005 M/s = k(0.1 M)<sup>2</sup>(0.1 M) => k = 50 M<sup>-2</sup>s<sup>-1</sup>
2. Graphical Method
This method involves plotting concentration versus time data. The shape of the graph reveals the reaction order.
- Zero-order: A plot of [Reactant] vs. time yields a straight line with a negative slope (-k).
- First-order: A plot of ln[Reactant] vs. time yields a straight line with a negative slope (-k).
- Second-order: A plot of 1/[Reactant] vs. time yields a straight line with a positive slope (k).
This method is particularly useful when dealing with reactions involving only one reactant, or when the concentration of one reactant is significantly greater than the others (pseudo-first-order conditions).
3. Half-Life Method
The half-life (t<sub>1/2</sub>) of a reaction is the time it takes for the concentration of a reactant to decrease to half its initial value. The relationship between half-life and reaction order is:
- Zero-order: t<sub>1/2</sub> = [A]<sub>0</sub> / 2k
- First-order: t<sub>1/2</sub> = 0.693 / k (independent of initial concentration)
- Second-order: t<sub>1/2</sub> = 1 / k[A]<sub>0</sub>
By measuring the half-lives at different initial concentrations, the reaction order can be determined. If the half-life is independent of initial concentration, it's first order.
Complications and Considerations
Determining rate laws can be more complex in real-world scenarios:
- Complex reactions: Many reactions proceed through multiple elementary steps. The overall rate law may not directly reflect the stoichiometry of the overall reaction.
- Side reactions: Competing reactions can affect the observed rate law.
- Catalyst effects: Catalysts can alter the reaction mechanism and rate law.
- Temperature dependence: The rate constant (k) is temperature-dependent, following the Arrhenius equation. Rate laws are typically determined at a constant temperature.
- Non-ideal behavior: At high concentrations, deviations from ideal behavior can occur, affecting the accuracy of the rate law.
Advanced Techniques
For more complex reactions or situations where simple methods are insufficient, more sophisticated techniques might be necessary:
- Relaxation methods: These techniques perturb the system from equilibrium and monitor its return to equilibrium, allowing the determination of rate constants for fast reactions.
- Stopped-flow techniques: These allow the rapid mixing of reactants and the monitoring of fast reactions.
- Computational methods: Computational chemistry can be used to model reaction mechanisms and predict rate laws.
Conclusion
Determining the rate law for an overall reaction is a crucial step in understanding reaction kinetics. The choice of method depends on the complexity of the reaction and the available experimental data. While the method of initial rates and graphical methods are commonly used for simpler reactions, more sophisticated techniques are often necessary for complex systems. Careful experimental design and data analysis are essential for obtaining accurate and reliable rate laws. Understanding the limitations and potential complications is crucial for interpreting the results correctly. By mastering these techniques, researchers can gain valuable insights into reaction mechanisms and predict reaction behavior under various conditions, fostering advancements in diverse fields ranging from chemical engineering to medicine.
Latest Posts
Latest Posts
-
Why Do Chemical Equations Have To Be Balanced
Mar 31, 2025
-
What Percent Is A 26 Out Of 30
Mar 31, 2025
-
Which Is Denser Oceanic Or Continental Crust
Mar 31, 2025
-
Root 3 Divided By Root 3
Mar 31, 2025
-
The Shoulder Is Blank To The Elbow
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Determine The Rate Law For The Overall Reaction . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
