Common Factors Of 30 And 48
listenit
May 23, 2025 · 6 min read
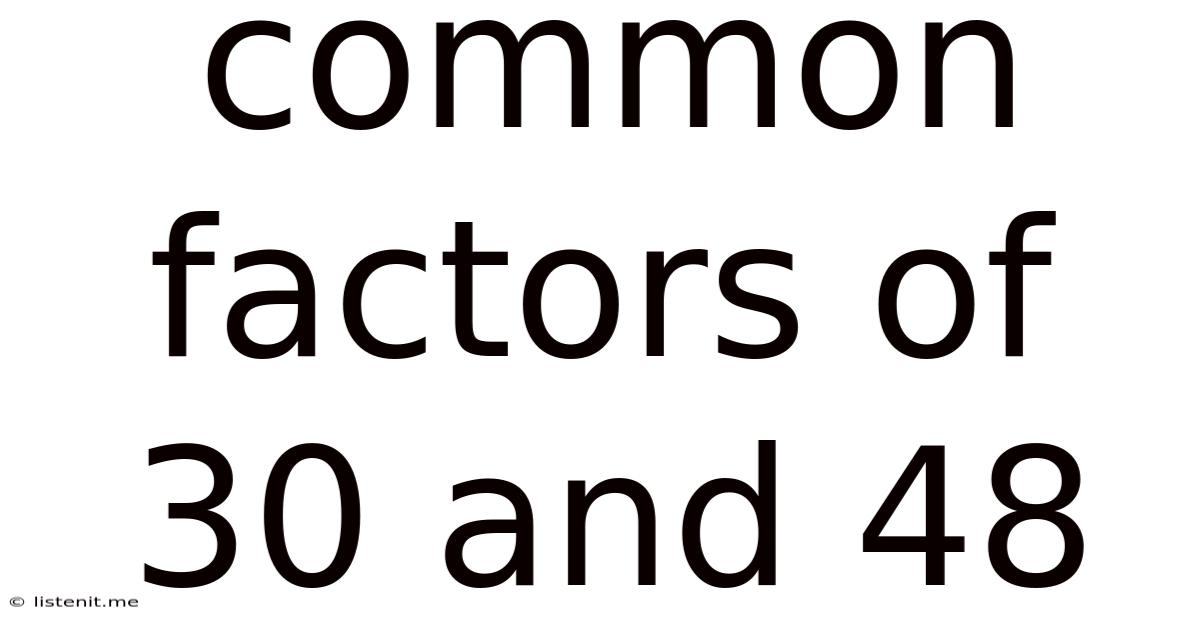
Table of Contents
Unveiling the Secrets of Common Factors: A Deep Dive into 30 and 48
Finding the common factors of two numbers might seem like a simple arithmetic exercise, but it's a fundamental concept in mathematics with applications far beyond the classroom. Understanding common factors is crucial in various fields, from simplifying fractions to solving complex algebraic equations and even optimizing computer algorithms. This comprehensive guide will explore the common factors of 30 and 48, delving into the methods used to identify them, their significance, and their broader mathematical implications.
Understanding Factors and Common Factors
Before we delve into the specifics of 30 and 48, let's establish a clear understanding of the core terminology.
Factors: Factors of a number are whole numbers that divide the number evenly, leaving no remainder. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12 because each of these numbers divides 12 without leaving a remainder.
Common Factors: When we consider two or more numbers, their common factors are the numbers that are factors of all of them. These shared factors represent the numbers that can evenly divide each of the original numbers.
Finding the Common Factors of 30 and 48: A Step-by-Step Approach
There are several methods to determine the common factors of 30 and 48. Let's explore two primary techniques:
1. Listing Factors
This method involves listing all the factors of each number individually and then identifying the factors that appear in both lists.
Factors of 30: 1, 2, 3, 5, 6, 10, 15, 30
Factors of 48: 1, 2, 3, 4, 6, 8, 12, 16, 24, 48
By comparing the two lists, we can easily see the common factors: 1, 2, 3, and 6.
2. Prime Factorization
This method utilizes the prime factorization of each number to find the common factors efficiently. Prime factorization involves expressing a number as a product of its prime factors (numbers divisible only by 1 and themselves).
Prime Factorization of 30: 2 x 3 x 5
Prime Factorization of 48: 2 x 2 x 2 x 2 x 3 = 2<sup>4</sup> x 3
To find the common factors, we identify the prime factors that appear in both factorizations. Both 30 and 48 have a 2 and a 3 as prime factors. Therefore, the common factors are all possible combinations of these prime factors:
- 1: (No prime factors)
- 2: (One 2)
- 3: (One 3)
- 6: (One 2 and one 3)
This method is particularly useful for larger numbers where listing all factors becomes cumbersome. It provides a systematic way to find all common factors without missing any.
Greatest Common Factor (GCF) of 30 and 48
Among the common factors, the greatest common factor (GCF) holds special significance. The GCF is simply the largest number that divides both 30 and 48 without leaving a remainder. In this case, the GCF of 30 and 48 is 6. The GCF is vital in various mathematical operations, as we will explore further.
Applications of Common Factors and GCF
The concept of common factors and the GCF extends far beyond simple number theory. Its applications are widespread across different mathematical areas and even real-world scenarios.
1. Simplifying Fractions
Simplifying fractions involves reducing them to their lowest terms. This is achieved by dividing both the numerator and denominator by their GCF. For example, consider the fraction 30/48. Since the GCF of 30 and 48 is 6, we can simplify the fraction as follows:
30/48 = (30 ÷ 6) / (48 ÷ 6) = 5/8
This process is crucial for representing fractions in their simplest and most manageable form.
2. Solving Algebraic Equations
Common factors play a critical role in solving algebraic equations, particularly those involving factorization. Factoring out common factors can simplify equations, making them easier to solve. For example:
6x + 12y = 6(x + 2y)
By factoring out the GCF (6), the equation becomes more manageable and easier to analyze.
3. Geometry and Measurement
Common factors are essential in geometry problems involving area calculations, perimeter, and similar shapes. For instance, when determining the dimensions of a rectangle with a given area and a constraint on the side lengths being whole numbers, the concept of common factors comes into play to find possible side lengths.
4. Computer Science and Algorithms
In computer science, the GCF is used in various algorithms, including the Euclidean algorithm, which is an efficient method for computing the GCF of two integers. This algorithm is fundamental in cryptography and other computationally intensive tasks.
5. Real-World Applications
Beyond the purely mathematical realm, common factors find practical applications in everyday life. For example:
-
Dividing resources equally: If you have 30 apples and 48 oranges to distribute evenly among groups of people, the GCF (6) determines the maximum number of equal groups you can create. Each group would get 5 apples and 8 oranges.
-
Arranging items in arrays: If you have 30 square tiles and want to arrange them into a rectangular array, finding the factors of 30 helps you determine the possible dimensions of the rectangle (e.g., 3 x 10, 5 x 6). Similarly, with 48 tiles, you can explore various rectangular arrangements.
-
Scheduling: Consider scenarios where you need to coordinate events that occur at regular intervals. The GCF helps find the earliest time when the events coincide again.
Beyond the Basics: Exploring Further Concepts
Understanding the common factors of 30 and 48 opens the door to a deeper exploration of related mathematical concepts. Here are some areas to delve into:
-
Least Common Multiple (LCM): While we focused on GCF, the LCM (Least Common Multiple) is another important concept. The LCM is the smallest number that is a multiple of both 30 and 48. Understanding both GCF and LCM is critical for various mathematical operations, including working with fractions and solving problems related to cycles and patterns.
-
Modular Arithmetic: The concepts of factors and multiples are fundamental to modular arithmetic, a branch of number theory with applications in cryptography and computer science.
-
Number Theory: Exploring the properties of numbers, including primes, composite numbers, and their relationships, expands the understanding of common factors and their significance within the broader context of number theory.
Conclusion: The Enduring Relevance of Common Factors
The common factors of 30 and 48, seemingly simple in isolation, provide a gateway to a wide array of mathematical concepts and practical applications. From simplifying fractions to optimizing algorithms, the understanding of common factors and the GCF is a fundamental skill with far-reaching implications across various disciplines. Mastering these concepts not only strengthens your mathematical foundation but also provides valuable tools for problem-solving in diverse fields. The journey into the world of numbers is a continuous exploration, and understanding these fundamental building blocks is crucial for further progress.
Latest Posts
Latest Posts
-
If I Was Born In 1954 How Old Am I
May 24, 2025
-
What Day Is In 36 Days
May 24, 2025
-
16 To The Power Of 5
May 24, 2025
-
What Is 25 Of 500 000
May 24, 2025
-
What Is Four Hours From Now
May 24, 2025
Related Post
Thank you for visiting our website which covers about Common Factors Of 30 And 48 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.