Common Factors Of 16 And 20
listenit
Apr 07, 2025 · 5 min read
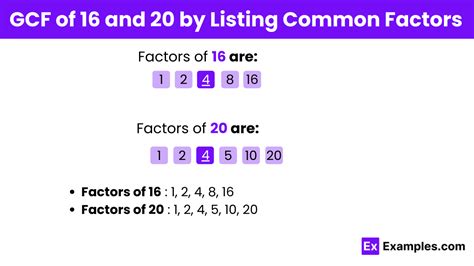
Table of Contents
Unlocking the Secrets of Numbers: Finding the Common Factors of 16 and 20
Finding the common factors of two numbers might seem like a simple arithmetic exercise, but understanding this concept unlocks a deeper appreciation for number theory and its applications in various fields. This comprehensive guide delves into the common factors of 16 and 20, exploring different methods to identify them, their significance in mathematics, and practical examples showcasing their relevance.
Understanding Factors and Common Factors
Before we dive into the specifics of 16 and 20, let's solidify our understanding of fundamental terms.
What are Factors?
Factors, also known as divisors, are whole numbers that divide evenly into a given number without leaving a remainder. For instance, the factors of 12 are 1, 2, 3, 4, 6, and 12, because each of these numbers divides 12 without leaving any remainder.
Identifying Factors: A Step-by-Step Approach
Finding the factors of a number can be done systematically. One method involves testing each whole number, starting from 1, to see if it divides the number evenly. Another efficient approach is to use factor pairs. For example, for the number 12:
- 1 x 12 = 12
- 2 x 6 = 12
- 3 x 4 = 12
This method ensures that we don't miss any factors.
What are Common Factors?
When we have two or more numbers, common factors are the numbers that are factors of all the given numbers. These shared factors are crucial in simplifying fractions, solving equations, and understanding the relationships between different numbers.
Finding the Common Factors of 16 and 20
Now, let's focus on our target numbers: 16 and 20. We'll employ several methods to uncover their common factors.
Method 1: Listing Factors
- Factors of 16: 1, 2, 4, 8, 16
- Factors of 20: 1, 2, 4, 5, 10, 20
By comparing the two lists, we can easily identify the common factors: 1, 2, and 4.
Method 2: Prime Factorization
Prime factorization involves breaking down a number into its prime factors (numbers divisible only by 1 and themselves). This method is particularly useful for larger numbers.
- Prime factorization of 16: 2 x 2 x 2 x 2 = 2⁴
- Prime factorization of 20: 2 x 2 x 5 = 2² x 5
The common prime factor is 2, and the lowest power of 2 present in both factorizations is 2². Therefore, the common factors are 2⁰ (which is 1), 2¹, (which is 2), and 2² (which is 4).
Method 3: Greatest Common Factor (GCF)
The greatest common factor (GCF), also known as the highest common factor (HCF), is the largest number that divides both numbers evenly. Finding the GCF helps us identify all common factors because all other common factors will be divisors of the GCF.
Using either the listing method or prime factorization, we find that the GCF of 16 and 20 is 4. Since the common factors are always divisors of the GCF, we can deduce that the common factors of 16 and 20 are 1, 2, and 4.
Significance of Common Factors
Understanding common factors isn't just an academic exercise; it has significant implications across diverse mathematical applications.
Simplifying Fractions
Common factors are essential when simplifying fractions. To simplify a fraction, we divide both the numerator and denominator by their greatest common factor. For example, if we have the fraction 16/20, we can simplify it by dividing both the numerator and denominator by their GCF, which is 4:
16/20 = (16 ÷ 4) / (20 ÷ 4) = 4/5
Solving Equations
Common factors play a crucial role in solving algebraic equations. Factoring expressions often involves identifying common factors to simplify equations and find solutions.
Number Theory and Cryptography
In number theory, the concept of common factors is fundamental to various theorems and concepts, such as the Euclidean algorithm for finding the GCF and applications in cryptography which rely on the difficulty of finding the common factors of very large numbers.
Geometry and Measurement
Common factors are used in geometry when dealing with problems involving scaling, ratios, and proportions. For example, when determining if two shapes are similar, the ratios of their corresponding sides must be equal, and this often involves simplifying fractions using common factors.
Real-World Applications
The concept of common factors extends far beyond the theoretical realm of mathematics. Here are some real-world examples:
-
Dividing resources equally: If you have 16 apples and 20 oranges, and you want to distribute them equally among several people, the common factors (1, 2, and 4) determine the number of people you can divide the fruits among without having any remainder.
-
Construction and design: In construction and design projects, understanding common factors helps with tasks like arranging tiles or creating patterns where the dimensions need to be divisible by certain numbers.
-
Scheduling and planning: In project planning and scheduling, the concept of common factors aids in determining the optimal time intervals for repeating tasks or synchronizing multiple activities.
Exploring Further: Beyond the Basics
While finding the common factors of 16 and 20 is straightforward, the principles involved extend to more complex scenarios.
Finding Common Factors of Larger Numbers
The methods we've discussed—listing factors, prime factorization, and finding the GCF—remain applicable for larger numbers. However, for significantly larger numbers, more sophisticated algorithms and computational tools become necessary.
Least Common Multiple (LCM)
The least common multiple (LCM) is the smallest number that is a multiple of two or more given numbers. The LCM and GCF are inversely related; knowing one helps determine the other.
Advanced Number Theory Concepts
The study of common factors leads to more advanced number theory concepts, including modular arithmetic, Diophantine equations, and the exploration of prime numbers and their properties.
Conclusion: The Power of Common Factors
The seemingly simple concept of common factors holds immense power and significance in mathematics and its applications in the real world. Understanding how to find common factors, particularly through methods such as prime factorization and the GCF, equips you with a fundamental tool for solving problems across numerous disciplines. From simplifying fractions to tackling complex equations and even aiding in real-world applications like resource allocation and project planning, the ability to identify common factors remains a valuable asset in navigating the numerical landscape. By mastering this concept, you unlock a deeper understanding of the interconnectedness and beauty of numbers.
Latest Posts
Latest Posts
-
How Many Chromosomes In A Daughter Cell
Apr 08, 2025
-
How Many Valence Electrons In Ne
Apr 08, 2025
-
Why Do Bones Heal Quicker Than Cartilage
Apr 08, 2025
-
What Is The Area Of Triangle Rst
Apr 08, 2025
-
New Ocean Crust Is Formed At
Apr 08, 2025
Related Post
Thank you for visiting our website which covers about Common Factors Of 16 And 20 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.