Circumference Of A 9 Inch Circle
listenit
Apr 02, 2025 · 6 min read
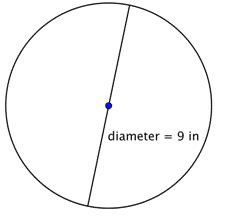
Table of Contents
Circumference of a 9-Inch Circle: A Deep Dive into Geometry and its Applications
The seemingly simple question of calculating the circumference of a 9-inch circle opens a door to a fascinating world of mathematical concepts and their real-world applications. While the basic formula is straightforward, understanding its derivation and exploring its uses in various fields offers a richer appreciation of geometry and its importance. This comprehensive guide will delve into the calculation, practical examples, and related mathematical concepts, ensuring a thorough understanding of the circumference of a 9-inch circle.
Understanding Circumference
The circumference of a circle is the distance around its edge. It's a fundamental concept in geometry, closely tied to the circle's diameter and radius. The diameter is the straight line passing through the center of the circle and connecting two points on the opposite side. The radius is half the length of the diameter. These three components – circumference, diameter, and radius – are intrinsically linked through a crucial mathematical constant: π (pi).
The Formula: C = 2πr or πd
The most common formula for calculating circumference is C = 2πr, where:
- C represents the circumference
- r represents the radius of the circle
- π (pi) is a mathematical constant, approximately equal to 3.14159. It represents the ratio of a circle's circumference to its diameter.
An alternative formula, equally valid, is C = πd, where:
- d represents the diameter of the circle.
Both formulas yield the same result, providing flexibility depending on whether the radius or diameter is known.
Calculating the Circumference of a 9-Inch Circle
For a 9-inch circle, we can utilize either formula. Since the diameter is given implicitly (9 inches), the second formula is more convenient:
C = πd = π * 9 inches
Using the approximation of π as 3.14159, we get:
C ≈ 3.14159 * 9 inches ≈ 28.27431 inches
Therefore, the circumference of a 9-inch circle is approximately 28.27 inches. The precision will depend on the value of π used in the calculation. Using a more precise value of π will yield a more accurate circumference. However, for most practical purposes, 28.27 inches is sufficiently accurate.
The Significance of Pi (π)
The constant π is central to understanding circular geometry. It's an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation goes on infinitely without repeating. This seemingly simple constant has profound implications across various scientific disciplines.
Approximations of Pi
Throughout history, mathematicians have dedicated significant effort to calculating π to ever-increasing precision. While 3.14 is a widely used approximation, more accurate values are available for specialized calculations. The availability of computing power has allowed for the calculation of π to trillions of digits, showcasing the depth and complexity of this fundamental constant.
Pi in Different Cultures
The understanding and appreciation of π transcends geographical boundaries. Ancient civilizations, including the Babylonians and Egyptians, developed their own approximations of π, reflecting the universal fascination with circles and their properties. The symbol π itself, adopted in the 18th century, has become a globally recognized symbol representing this fundamental mathematical constant.
Practical Applications of Circumference Calculations
The ability to calculate circumference is not merely an academic exercise. It has practical applications across a vast range of fields:
Engineering and Design
- Designing circular components: Engineers frequently use circumference calculations in designing wheels, gears, pipes, and other circular components. Accurate circumference calculations are crucial for ensuring proper fit and functionality.
- Calculating the length of materials: Knowing the circumference is essential when determining the length of materials needed to create circular structures or enclosures, like a circular fence or a ring.
- Robotics and automation: Precise circumference calculations are critical in robotics for tasks involving circular motion, such as robotic arms maneuvering around circular pathways or robotic wheels traversing circular routes.
Construction and Architecture
- Calculating material requirements: Construction projects often involve circular elements. Accurate circumference calculations are essential to determine the quantity of materials needed for constructing circular features like circular pools, foundations, or dome-like structures.
- Laying out circular pathways: Architects and landscape designers utilize circumference calculations to plan and lay out circular paths, driveways, or other circular features in their projects.
- Estimating material costs: Accurate circumference calculations contribute significantly to precise cost estimations for materials used in projects involving circular components.
Manufacturing and Production
- Manufacturing circular products: Industries producing items with circular components, such as cans, plates, and discs, rely heavily on circumference calculations for optimal production processes and quality control.
- Cutting and shaping materials: Precision cutting and shaping of circular materials necessitate precise circumference calculations to ensure that the final product meets the desired specifications.
- Automation and robotics in manufacturing: Precise circumference calculations are crucial in automated manufacturing processes involving robots that handle and manipulate circular parts.
Everyday Applications
The concept of circumference is also relevant in everyday situations:
- Calculating the distance of a circular track: Knowing the circumference of a circular running track or a circular walking path is essential for determining the distance covered during exercise or physical activity.
- Estimating the length of a circular object: Estimating the length of a circular object like a necklace, a bracelet, or a belt often involves an approximation of its circumference.
- Cooking and Baking: When baking pies or cakes with a circular shape, understanding circumference can help in determining the appropriate amount of ingredients or the size of the baking dish.
Beyond the Basics: Exploring Related Concepts
Understanding the circumference of a circle opens avenues to explore more complex geometrical concepts:
Area of a Circle
The area of a circle (A) is related to its radius (r) by the formula A = πr². This formula is closely related to the circumference formula and is frequently used in conjunction with circumference calculations in various applications.
Arc Length
An arc is a portion of a circle's circumference. Calculating the length of an arc requires knowing the central angle subtended by the arc and the radius of the circle. This involves using a proportional relationship between the arc length and the total circumference.
Sectors and Segments
A sector is a region of a circle bounded by two radii and an arc. A segment is a region of a circle bounded by a chord and an arc. Calculating the area of sectors and segments involves the use of the circle's radius, circumference, and the central angle.
Conclusion: The Enduring Importance of Circumference
The seemingly simple calculation of the circumference of a 9-inch circle underscores the importance of fundamental geometrical concepts in a wide range of applications. From engineering marvels to everyday tasks, the ability to accurately calculate circumference is essential. This deep dive into the topic reveals the power and relevance of geometry, highlighting the practical implications of a concept often viewed as merely an academic exercise. Understanding the intricacies of circumference not only enhances mathematical literacy but also equips individuals with valuable problem-solving skills applicable across diverse fields. The enduring importance of this simple concept is undeniable, proving its relevance across various disciplines and applications. Further exploration of related concepts like area, arc length, sectors, and segments will solidify a comprehensive understanding of circular geometry and its far-reaching impact.
Latest Posts
Latest Posts
-
60 Percent Of 40 Is What Number
Apr 03, 2025
-
How Does Friction Affect An Objects Motion
Apr 03, 2025
-
How Many Gallons In 12 Pints
Apr 03, 2025
-
Whats The Derivative Of A Constant
Apr 03, 2025
-
The Unit Of Energy In S I Units Is
Apr 03, 2025
Related Post
Thank you for visiting our website which covers about Circumference Of A 9 Inch Circle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
