Area Of A 18 Inch Circle
listenit
Mar 28, 2025 · 5 min read
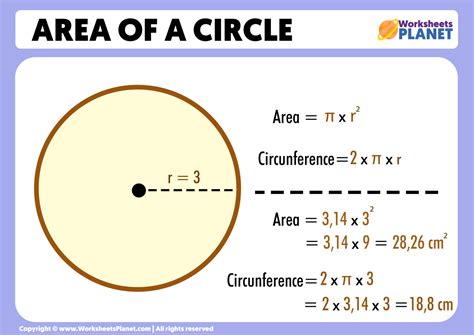
Table of Contents
Calculating the Area of an 18-Inch Circle: A Comprehensive Guide
Determining the area of a circle is a fundamental concept in geometry with widespread applications in various fields, from engineering and design to everyday life. This comprehensive guide will delve into the calculation of the area of an 18-inch circle, exploring the underlying formula, practical applications, and related concepts. We'll also address common misconceptions and provide helpful tips to ensure accuracy in your calculations.
Understanding the Formula: Pi, Radius, and Area
The area of any circle is calculated using a simple yet powerful formula:
Area = πr²
Where:
- A represents the area of the circle.
- π (pi) is a mathematical constant, approximately equal to 3.14159. Pi represents the ratio of a circle's circumference to its diameter. For high-precision calculations, you can use a more accurate value for pi, such as 3.14159265359.
- r represents the radius of the circle, which is the distance from the center of the circle to any point on its circumference.
In our case, we are dealing with an 18-inch circle. This means the diameter of the circle is 18 inches. To use the formula, we need the radius. The radius is half of the diameter.
Calculating the Radius
Since the diameter is 18 inches, the radius (r) is:
r = Diameter / 2 = 18 inches / 2 = 9 inches
Calculating the Area of the 18-Inch Circle
Now that we have the radius, we can plug it into the area formula:
Area = πr² = π * (9 inches)² = π * 81 square inches
Using the approximation of π ≈ 3.14159:
Area ≈ 3.14159 * 81 square inches ≈ 254.469 square inches
Therefore, the area of an 18-inch circle is approximately 254.47 square inches. Remember that this is an approximation due to the use of an approximate value for pi. Using a calculator or computer software with higher precision for pi will yield a more precise result.
Practical Applications of Circle Area Calculations
Understanding how to calculate the area of a circle has numerous practical applications in various fields:
1. Engineering and Design:
- Civil Engineering: Calculating the area of circular foundations, pipes, and other components is crucial for structural design and material estimations.
- Mechanical Engineering: Determining the cross-sectional area of circular shafts, pistons, and other machine parts is essential for stress analysis and performance calculations.
- Electrical Engineering: Calculating the area of circular conductors helps in determining their current-carrying capacity and resistance.
2. Architecture and Construction:
- Floor Planning: Architects use circle area calculations to determine the space required for circular features like rotunda designs or decorative elements.
- Material Estimation: Calculating the area of circular features assists in determining the quantity of materials needed for flooring, tiling, or painting.
3. Landscaping and Gardening:
- Irrigation Systems: Designing efficient irrigation systems requires calculating the area covered by circular sprinklers.
- Garden Planning: Determining the area of circular flowerbeds or garden plots aids in planting and material planning.
4. Everyday Life:
- Baking and Cooking: Determining the area of a pizza or a cake helps in adjusting recipes and serving sizes.
- Crafting and DIY Projects: Calculating circle areas is vital for creating circular designs, cutting materials, or determining the amount of material needed for projects.
Beyond the Basic Formula: Exploring Related Concepts
While the basic formula provides a straightforward method for calculating the area of a circle, exploring related concepts can enhance understanding and problem-solving skills.
1. Circumference:
The circumference of a circle is the distance around it. It's calculated using the formula:
Circumference = 2πr
For our 18-inch circle:
Circumference = 2 * π * 9 inches ≈ 56.55 inches
2. Sector Area:
A sector is a portion of a circle enclosed by two radii and an arc. The area of a sector is calculated using the formula:
Sector Area = (θ/360°) * πr²
Where θ is the central angle of the sector in degrees.
3. Segment Area:
A segment is the area between a chord and an arc. Calculating the area of a segment involves subtracting the area of a triangle from the area of a sector. The specific formula depends on the information available (e.g., the chord length, the height of the segment).
4. Annulus Area:
An annulus is the region between two concentric circles. The area of an annulus is calculated by subtracting the area of the smaller circle from the area of the larger circle.
Common Mistakes to Avoid
Several common mistakes can lead to inaccurate area calculations:
- Confusing radius and diameter: Remember that the radius is half the diameter. Using the diameter directly in the formula will result in a significantly larger, incorrect area.
- Incorrect use of pi: Using an overly simplified value for pi can lead to minor inaccuracies, especially in larger circles. Using the full value or at least 3.14159 is recommended for higher precision.
- Units: Always pay attention to the units. If the radius is given in inches, the area will be in square inches.
Tips for Accurate Calculations
- Use a calculator: For efficient and precise calculations, always use a calculator or computer software, especially for more complex problems involving sectors, segments, or annuli.
- Double-check your work: After completing the calculation, review each step to ensure accuracy.
- Round appropriately: When rounding your answer, consider the level of precision needed for the specific application.
Conclusion: Mastering Circle Area Calculations
Calculating the area of an 18-inch circle, or any circle for that matter, is a fundamental skill with far-reaching implications across various disciplines. By understanding the formula, its applications, and potential pitfalls, you can confidently tackle these calculations and utilize them effectively in your personal and professional endeavors. Remember to always double-check your work and use appropriate precision for optimal results. This knowledge empowers you to solve problems efficiently and accurately, making it a valuable asset in numerous contexts.
Latest Posts
Latest Posts
-
How Many Ounces Is 1 4 Pound
Mar 31, 2025
-
What Is 65 As A Fraction
Mar 31, 2025
-
What Number Is 60 Of 145
Mar 31, 2025
-
The Ability Of An Organism To Survive And Reproduce
Mar 31, 2025
-
What Is A Master Level Clinician
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about Area Of A 18 Inch Circle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
