93 Out Of 150 As A Percentage
listenit
May 11, 2025 · 5 min read
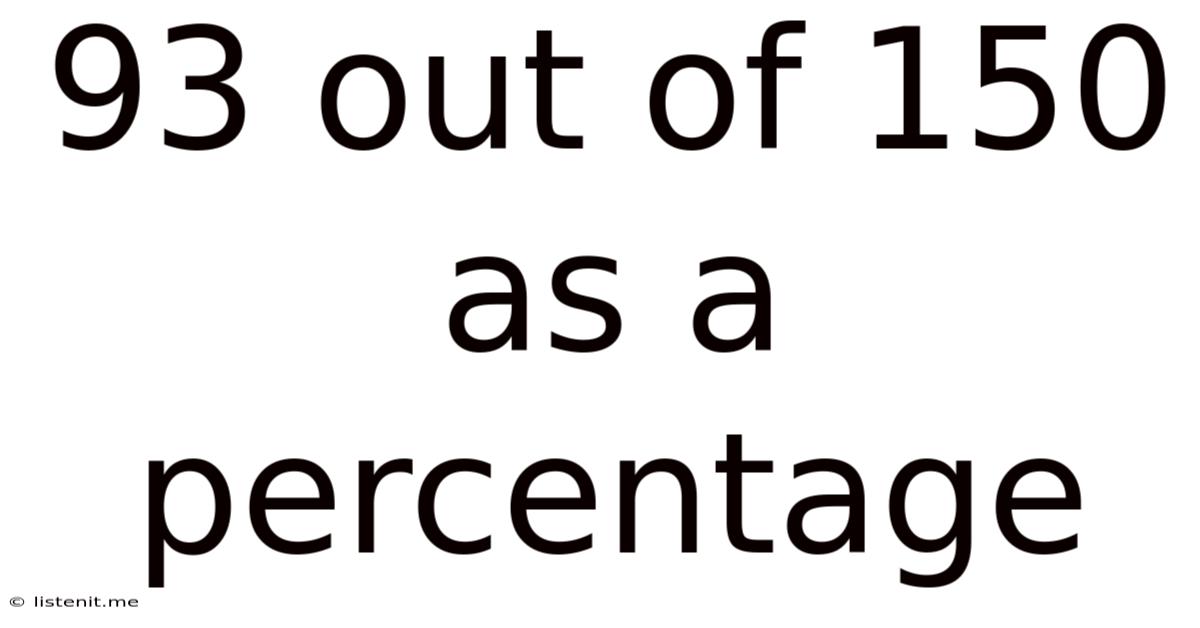
Table of Contents
93 out of 150 as a Percentage: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with broad applications in various aspects of life, from academic assessments and financial transactions to everyday decision-making. Understanding how to convert fractions and ratios into percentages is crucial for interpreting data, making comparisons, and understanding proportions. This article dives deep into calculating 93 out of 150 as a percentage, explaining the process step-by-step and providing valuable insights into percentage calculations in general.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "per hundred." Therefore, 50% means 50 out of 100, which simplifies to one-half (1/2) or 0.5 in decimal form. Percentages are used to represent parts of a whole and provide a standardized way to compare different proportions.
Calculating 93 out of 150 as a Percentage: The Method
To calculate 93 out of 150 as a percentage, we follow these straightforward steps:
Step 1: Set up the Fraction
First, represent the given numbers as a fraction. The number representing the part (93) is the numerator, and the number representing the whole (150) is the denominator:
93/150
Step 2: Convert the Fraction to a Decimal
To convert the fraction to a decimal, divide the numerator (93) by the denominator (150):
93 ÷ 150 = 0.62
Step 3: Convert the Decimal to a Percentage
To convert the decimal to a percentage, multiply the decimal by 100 and add the percentage symbol (%):
0.62 × 100 = 62%
Therefore, 93 out of 150 is 62%.
Alternative Calculation Methods
While the method above is the most common and straightforward, there are alternative approaches to arrive at the same result:
Method 1: Using Proportions
We can set up a proportion to solve for the percentage:
x/100 = 93/150
Cross-multiplying, we get:
150x = 9300
Dividing both sides by 150:
x = 9300/150 = 62
Therefore, x = 62%, confirming our previous result.
Method 2: Simplifying the Fraction First
Before converting to a decimal, we can simplify the fraction 93/150 by finding the greatest common divisor (GCD) of 93 and 150. The GCD of 93 and 150 is 3. Dividing both the numerator and denominator by 3, we get:
93 ÷ 3 = 31 150 ÷ 3 = 50
This simplifies the fraction to 31/50. Now, converting this simplified fraction to a decimal:
31 ÷ 50 = 0.62
Converting the decimal to a percentage:
0.62 × 100 = 62%
This method demonstrates that simplifying the fraction beforehand can sometimes make the calculation easier.
Practical Applications of Percentage Calculations
Understanding percentage calculations is essential in numerous real-world scenarios. Here are a few examples:
1. Academic Performance
In education, percentages are used extensively to represent student performance on tests and assignments. A score of 93 out of 150, representing a grade of 62%, provides a clear indication of the student's understanding of the subject matter.
2. Financial Calculations
Percentages are crucial in finance for calculating interest rates, discounts, tax rates, and profit margins. For instance, understanding percentage increases or decreases in investment values is vital for making informed financial decisions.
3. Data Analysis and Statistics
In data analysis and statistics, percentages are used to represent proportions and probabilities. For example, percentages can help visualize the distribution of data in various categories or represent the likelihood of certain events occurring.
4. Sales and Marketing
Percentages are commonly used in sales and marketing to represent discounts, sales growth, and market share. Understanding these percentages is essential for making strategic business decisions.
Beyond the Basics: More Complex Percentage Calculations
While the calculation of 93 out of 150 as a percentage is relatively simple, let's explore some more complex scenarios that build upon this fundamental understanding:
Calculating Percentage Increase or Decrease
Imagine a scenario where a value increases or decreases by a certain percentage. For example, if a product's price increases by 10%, you need to calculate the new price. Similarly, calculating percentage discounts involves finding the final price after a discount is applied.
Finding the Original Value
Sometimes, you know the final value after a percentage increase or decrease and need to determine the original value. For instance, if a product is discounted by 20% and sells for $80, you need to calculate the original price before the discount.
Working with Multiple Percentages
Calculations can become more complex when dealing with multiple percentages. For instance, imagine calculating the final price after two successive discounts (e.g., 10% and 5%). You need to carefully apply the percentages sequentially.
Understanding Percentage Points
It's important to differentiate between percentage changes and percentage points. A change of 5 percentage points is different from a 5% increase. For example, if a score increases from 50% to 55%, the percentage point increase is 5, but the percentage increase is 10% (5/50 x 100 = 10%).
Conclusion: Mastering Percentage Calculations
Calculating percentages is a fundamental mathematical skill with far-reaching applications. Understanding the methods and processes involved, as demonstrated by the calculation of 93 out of 150 as 62%, empowers you to interpret data, make informed decisions, and effectively navigate various aspects of your personal and professional life. By mastering these skills, you'll be better equipped to analyze information, solve problems, and succeed in a wide range of situations. From academic achievements to financial literacy, and from data analysis to business strategies, the ability to work confidently with percentages is a valuable asset in today's world. Remember to practice regularly and explore more complex scenarios to solidify your understanding and build confidence in your ability to handle percentage calculations with ease.
Latest Posts
Latest Posts
-
Why Do Electric Field Lines Never Cross
May 13, 2025
-
1 10 As A Percent And Decimal
May 13, 2025
-
Can All Minerals Be A Gemstone
May 13, 2025
-
Multicellular Heterotrophs Without A Cell Wall
May 13, 2025
-
What Are The Gcf Of 48
May 13, 2025
Related Post
Thank you for visiting our website which covers about 93 Out Of 150 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.