8 Of 50 Is What Percent
listenit
May 24, 2025 · 4 min read
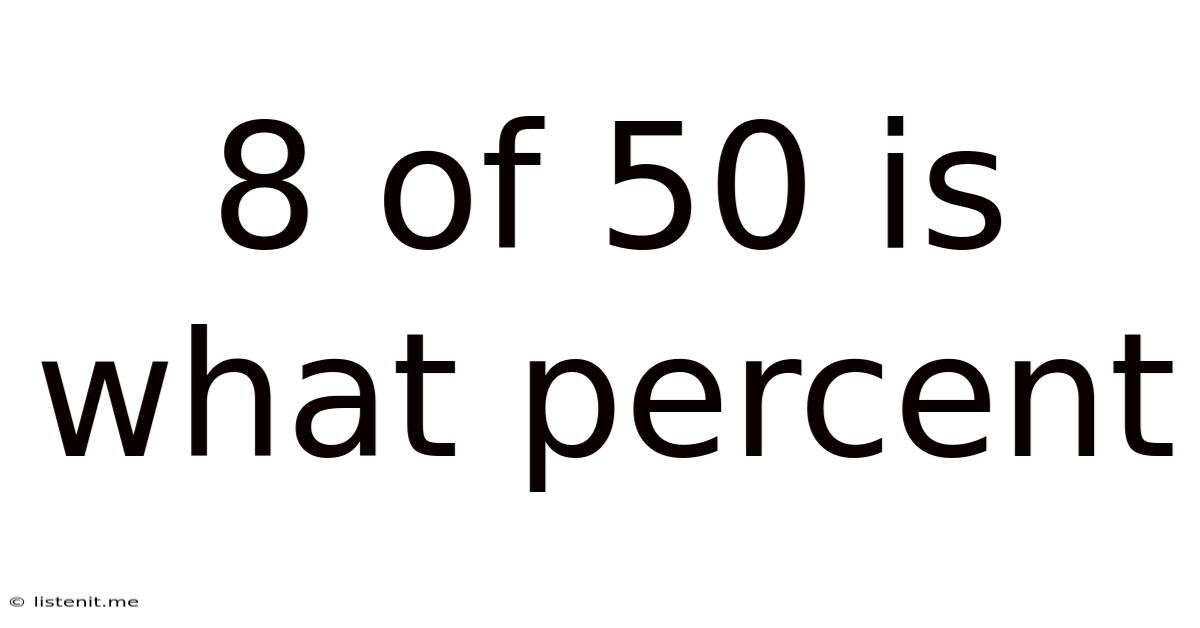
Table of Contents
8 out of 50 is what percent? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across various aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This comprehensive guide delves into the question, "8 out of 50 is what percent?" We'll explore multiple methods to solve this problem, providing a solid foundation for tackling similar percentage calculations. We'll also cover related concepts to enhance your understanding and problem-solving skills.
Understanding Percentages: The Basics
A percentage is a fraction or ratio expressed as a number out of 100. The term "percent" literally means "out of one hundred" (per centum). Therefore, finding a percentage involves determining what portion of a whole represents a specific part.
In the context of our problem, "8 out of 50," we want to determine what fraction of 100 8 represents when the total is 50.
Method 1: Using the Fraction Method
This is arguably the most intuitive method. We can express "8 out of 50" as a fraction: 8/50. To convert this fraction to a percentage, we need to find an equivalent fraction with a denominator of 100.
-
Simplify the Fraction (Optional but Recommended): We can simplify 8/50 by dividing both the numerator and the denominator by their greatest common divisor, which is 2. This simplifies the fraction to 4/25.
-
Convert to a Percentage: To get a denominator of 100, we multiply both the numerator and the denominator by 4 (since 25 x 4 = 100):
(4 x 4) / (25 x 4) = 16/100
-
Express as a Percentage: 16/100 is equivalent to 16%. Therefore, 8 out of 50 is 16%.
Method 2: Using the Decimal Method
This method involves converting the fraction to a decimal and then multiplying by 100.
-
Convert the Fraction to a Decimal: Divide the numerator (8) by the denominator (50):
8 ÷ 50 = 0.16
-
Multiply by 100 to Convert to a Percentage:
0.16 x 100 = 16%
Therefore, using the decimal method also confirms that 8 out of 50 is 16%.
Method 3: Using Proportions
This method uses the concept of proportions to solve the problem. We can set up a proportion to find the equivalent percentage:
8/50 = x/100
Where 'x' represents the percentage we want to find.
-
Cross-Multiply: To solve for 'x', we cross-multiply:
50x = 800
-
Solve for x: Divide both sides by 50:
x = 800 ÷ 50 = 16
Therefore, x = 16%, confirming that 8 out of 50 is 16%.
Practical Applications of Percentage Calculations
Understanding how to calculate percentages is crucial in numerous real-world scenarios. Here are a few examples:
-
Discounts: Calculating the price after a discount. For example, a 20% discount on a $100 item.
-
Taxes: Determining the total cost of an item including sales tax.
-
Grade Calculation: Converting scores on tests or assignments into percentages.
-
Financial Analysis: Analyzing financial statements and calculating profit margins, interest rates, and returns on investments.
-
Data Analysis: Representing data visually using percentages in charts and graphs.
Beyond the Basics: Advanced Percentage Problems
While calculating "8 out of 50 is what percent?" is relatively straightforward, more complex percentage problems often arise. Let's explore some:
-
Finding the Percentage Increase or Decrease: This involves determining the percentage change between two values. For instance, if a stock price increases from $50 to $60, the percentage increase is calculated as follows:
((60-50)/50) * 100 = 20%
-
Finding a Number Given a Percentage: This type of problem involves determining the original number when you know the percentage and the resulting value. For example, if 25% of a number is 10, the original number is 40 (10 ÷ 0.25 = 40).
-
Calculating Percentage Points: Percentage points represent the absolute difference between two percentages. For example, if interest rates rise from 2% to 5%, the increase is 3 percentage points, not 3%.
Tips for Mastering Percentage Calculations
-
Practice Regularly: Consistent practice is key to mastering any mathematical skill, including percentage calculations.
-
Use Multiple Methods: Try different methods to solve the same problem to reinforce your understanding and find the method that suits you best.
-
Understand the Underlying Concepts: Don't just memorize formulas; understand the underlying principles of fractions, decimals, and ratios.
-
Utilize Online Resources: Many online resources, such as calculators and tutorials, can help you practice and improve your skills.
Conclusion
Determining that 8 out of 50 is 16% might seem simple, but understanding the different methods of calculation is essential for tackling more complex percentage problems. By mastering these techniques and understanding the fundamental concepts, you'll be well-equipped to handle various percentage calculations in your academic, professional, and personal life. The ability to work confidently with percentages opens doors to a wider understanding of quantitative information and data analysis across numerous fields. Remember that consistent practice is the key to mastery, and don't hesitate to explore additional resources to reinforce your knowledge.
Latest Posts
Latest Posts
-
How Much Days Is 4 Months
May 24, 2025
-
What Is The Greatest Common Factor Of 48 And 12
May 24, 2025
-
35 Out Of 41 As A Percentage
May 24, 2025
-
What Is The Greatest Common Factor Of 18 And 72
May 24, 2025
-
5 X 10 To The Power Of 3
May 24, 2025
Related Post
Thank you for visiting our website which covers about 8 Of 50 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.