67 12 As A Mixed Number
listenit
May 10, 2025 · 4 min read
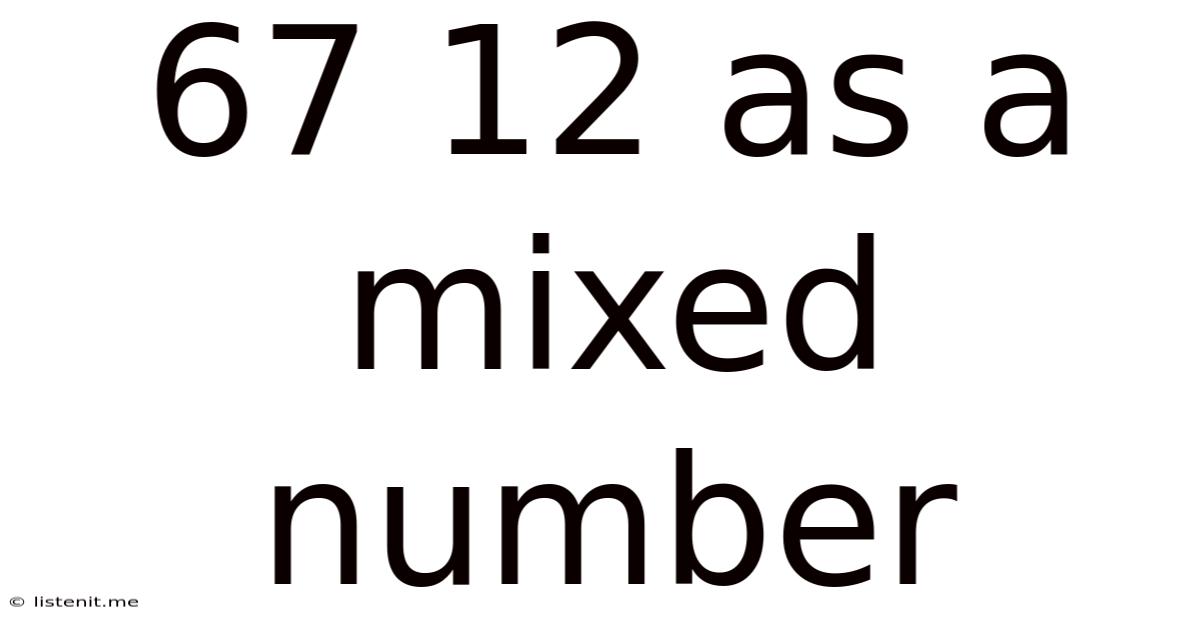
Table of Contents
67/12 as a Mixed Number: A Comprehensive Guide
Converting improper fractions to mixed numbers is a fundamental skill in arithmetic. This comprehensive guide will delve into the process of transforming the improper fraction 67/12 into a mixed number, explaining the underlying concepts and providing various methods to solve this and similar problems. We'll also explore the importance of understanding mixed numbers in real-world applications and further develop your understanding of fraction manipulation.
Understanding Improper Fractions and Mixed Numbers
Before we dive into converting 67/12, let's clarify the definitions:
-
Improper Fraction: An improper fraction is a fraction where the numerator (the top number) is greater than or equal to the denominator (the bottom number). Examples include 7/4, 11/5, and, of course, 67/12.
-
Mixed Number: A mixed number combines a whole number and a proper fraction. A proper fraction is a fraction where the numerator is less than the denominator (e.g., 1/2, 3/4, 5/8). Mixed numbers represent quantities that are greater than one.
The conversion between improper fractions and mixed numbers represents the same quantity, just expressed differently. Understanding this equivalence is crucial for various mathematical operations.
Method 1: Long Division
The most straightforward method for converting an improper fraction like 67/12 to a mixed number involves long division.
-
Divide the numerator by the denominator: Divide 67 by 12.
5 12 | 67 -60 7 -
Identify the quotient and the remainder: The quotient (the result of the division) is 5, and the remainder is 7.
-
Express the mixed number: The quotient becomes the whole number part of the mixed number, and the remainder becomes the numerator of the fractional part. The denominator remains the same as the original fraction's denominator.
Therefore, 67/12 = 5 7/12
Method 2: Repeated Subtraction
This method offers a more intuitive approach, especially for visualizing the conversion.
-
Repeatedly subtract the denominator from the numerator: Repeatedly subtract 12 from 67 until you reach a number less than 12.
67 - 12 = 55 55 - 12 = 43 43 - 12 = 31 31 - 12 = 19 19 - 12 = 7
-
Count the number of subtractions: You subtracted 12 a total of five times. This represents the whole number part of the mixed number.
-
The remaining number is the numerator: The remaining number after the subtractions (7) is the numerator of the fractional part. The denominator remains 12.
Therefore, 67/12 = 5 7/12
Method 3: Using Fraction Properties
This method utilizes the principle of equivalent fractions. We aim to express the improper fraction as a sum of a whole number and a proper fraction.
-
Find a multiple of the denominator close to the numerator: 12 x 5 = 60. This is close to 67.
-
Express the numerator as a sum: 67 = 60 + 7
-
Rewrite the fraction: 67/12 = (60 + 7)/12
-
Separate the fraction: (60 + 7)/12 = 60/12 + 7/12
-
Simplify: 60/12 simplifies to 5.
Therefore, 67/12 = 5 + 7/12 = 5 7/12
Verifying the Conversion
It's always good practice to verify your conversion to ensure accuracy. You can convert the mixed number back into an improper fraction to check:
-
Multiply the whole number by the denominator: 5 x 12 = 60
-
Add the numerator: 60 + 7 = 67
-
Place the result over the denominator: 67/12
This confirms that our conversion is correct.
Practical Applications of Mixed Numbers
Mixed numbers are frequently used in everyday life and various fields:
-
Cooking and Baking: Recipes often call for mixed numbers (e.g., 2 1/2 cups of flour).
-
Measurement: Measuring lengths, weights, and volumes frequently involves mixed numbers (e.g., 5 3/4 inches).
-
Construction and Engineering: Precise measurements and calculations in construction and engineering often utilize mixed numbers.
-
Data Analysis: Representing data or averages can involve mixed numbers to provide a more intuitive understanding.
-
Time: Expressing time frequently uses mixed numbers (e.g., 1 hour and 15 minutes can be represented as 1 1/4 hours).
Further Exploration: Working with Mixed Numbers
Once you understand how to convert improper fractions to mixed numbers, you can explore more advanced operations involving mixed numbers:
-
Addition and Subtraction of Mixed Numbers: Requires converting mixed numbers to improper fractions, performing the operation, and then converting back to a mixed number.
-
Multiplication and Division of Mixed Numbers: Similar to addition and subtraction, conversion to improper fractions simplifies these operations.
-
Comparing Mixed Numbers: Understanding the relative sizes of mixed numbers is essential for various mathematical comparisons.
Conclusion: Mastering Fraction Conversion
Converting improper fractions to mixed numbers is a fundamental skill with wide-ranging applications. By mastering the methods outlined in this guide – long division, repeated subtraction, and using fraction properties – you'll be well-equipped to handle this type of conversion effectively. Remember to always verify your results and explore the various applications of mixed numbers to solidify your understanding. The ability to confidently work with fractions is a cornerstone of mathematical proficiency, paving the way for success in more complex mathematical concepts and real-world problem-solving. Practice is key; the more you work with fractions, the more intuitive and effortless the process will become.
Latest Posts
Latest Posts
-
Write 0 2 As A Fraction In Simplest Form
May 10, 2025
-
Find The Mass Of 250 0 Ml Of Benzene
May 10, 2025
-
What Element Family Does Potassium Belong To
May 10, 2025
-
Which Plant Organelle Is Responsible For Photosynthesis
May 10, 2025
-
What Is The Largest Reservoir Of Nitrogen On Earth
May 10, 2025
Related Post
Thank you for visiting our website which covers about 67 12 As A Mixed Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.