6 Is What Percent Of 23
listenit
May 25, 2025 · 4 min read
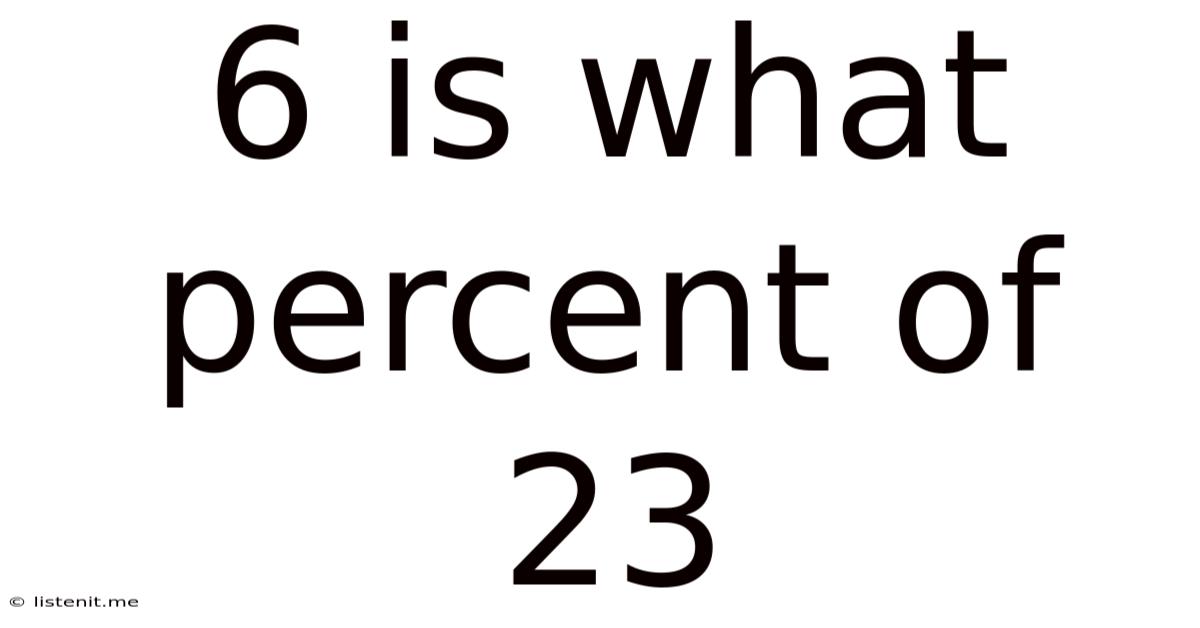
Table of Contents
6 is What Percent of 23? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from everyday finances to complex scientific calculations. This article delves deep into the question, "6 is what percent of 23?", providing not just the answer but a comprehensive understanding of the underlying principles and various methods to solve similar percentage problems. We'll explore different approaches, from basic arithmetic to utilizing online calculators and even discuss the applications of percentages in real-world scenarios.
Understanding Percentages: The Basics
Before diving into the specific problem, let's establish a firm grasp of percentages. A percentage is simply a fraction expressed as a number out of 100. The symbol "%" denotes percentage. For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
The fundamental formula for calculating percentages is:
(Part / Whole) * 100% = Percentage
In our case, "6 is what percent of 23?", 6 represents the part, and 23 represents the whole.
Method 1: Direct Calculation
Using the fundamental formula, we can directly calculate the percentage:
(6 / 23) * 100% = 26.0869565%
Rounding to two decimal places, we get 26.09%. Therefore, 6 is approximately 26.09% of 23.
Method 2: Using a Calculator
Most calculators, whether physical or digital, have a percentage function. This simplifies the process significantly. Simply input "6 ÷ 23 * 100" and press the "=" button. The result will be the same as the direct calculation: approximately 26.09%.
Method 3: Proportions
Another way to approach this problem is by setting up a proportion:
6/23 = x/100
Here, 'x' represents the percentage we are trying to find. To solve for 'x', we cross-multiply:
6 * 100 = 23 * x
600 = 23x
x = 600/23
x ≈ 26.09%
This method reinforces the concept of equivalent ratios and offers a different perspective on percentage calculations.
Method 4: Breaking Down the Percentage
While the previous methods are efficient, understanding the underlying concept can be beneficial. We can approximate the percentage by breaking down the problem. 23 is roughly 2.3 times 10 (i.e., approximately 2.3 x 10 = 23). If 6 were part of 10, it would represent 60%. Since 23 is approximately 2.3 times larger than 10, we can roughly estimate the percentage by dividing 60% by 2.3:
60% / 2.3 ≈ 26.09%
This method is less precise but provides a valuable mental approximation technique.
Real-World Applications of Percentage Calculations
Understanding percentages is crucial in various real-life situations:
1. Finance:
- Interest Rates: Calculating interest earned on savings accounts or interest paid on loans requires percentage calculations.
- Discounts: Determining the final price of an item after a discount is applied involves calculating a percentage reduction.
- Taxes: Computing sales tax or income tax relies on understanding percentages.
- Investment Returns: Tracking the growth or decline of investments necessitates using percentages to measure returns.
2. Science and Statistics:
- Data Analysis: Percentages are frequently used to represent data in graphs and charts, aiding in the interpretation of statistical information.
- Experimental Results: Scientists use percentages to express the success rate of experiments or the proportion of a specific outcome.
3. Everyday Life:
- Tip Calculations: Determining the appropriate tip in a restaurant involves calculating a percentage of the bill.
- Shopping: Comparing prices and finding the best deals often relies on understanding percentage discounts.
- Surveys and Polls: Percentages are used to summarize the results of surveys and polls, providing insights into public opinion.
Beyond the Calculation: Improving Percentage Intuition
While knowing how to calculate percentages is essential, developing an intuitive understanding is equally important. This allows for quick estimations and a deeper comprehension of numerical relationships. Here are some tips:
- Memorize common percentages: Familiarize yourself with the percentage equivalents of common fractions (e.g., 1/2 = 50%, 1/4 = 25%, 1/3 ≈ 33.33%).
- Practice regularly: The more you practice percentage calculations, the more comfortable and efficient you will become.
- Use visual aids: Diagrams and charts can help to visualize percentage relationships.
- Break down complex problems: Divide larger problems into smaller, more manageable steps.
Advanced Percentage Concepts
For those interested in delving further into the world of percentages, here are some more advanced concepts to explore:
- Percentage Change: This calculates the relative change between two values. The formula is: [(New Value - Old Value) / Old Value] * 100%.
- Percentage Point Change: This measures the absolute change between two percentages, unlike percentage change which measures the relative change. For example, a change from 10% to 15% is a 5 percentage point change.
- Compounding Percentages: This refers to applying a percentage change repeatedly over time, often used in finance to calculate compound interest.
Conclusion
Calculating "6 is what percent of 23?" is a straightforward problem, highlighting the fundamental principles of percentage calculations. We've explored several methods—direct calculation, using a calculator, proportions, and approximation—each offering a different perspective on the same problem. The ability to solve such problems extends far beyond the realm of mathematics, finding practical applications in various aspects of daily life, from financial planning to scientific analysis. By understanding the core concepts and practicing regularly, you can confidently navigate the world of percentages and harness their power in your personal and professional endeavors. Remember to utilize the method most comfortable and efficient for you, and don't hesitate to leverage technology to simplify calculations when needed.
Latest Posts
Latest Posts
-
Greatest Common Factor Of 15 And 50
May 25, 2025
-
Find The Greatest Common Factor Of 30 And 18
May 25, 2025
-
2 Hour 10 Minute Marathon Pace
May 25, 2025
-
How Much Is 300 Km In Mph
May 25, 2025
-
Least Common Multiple Of 6 And 21
May 25, 2025
Related Post
Thank you for visiting our website which covers about 6 Is What Percent Of 23 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.