5 Of What Number Is 5
listenit
May 10, 2025 · 5 min read
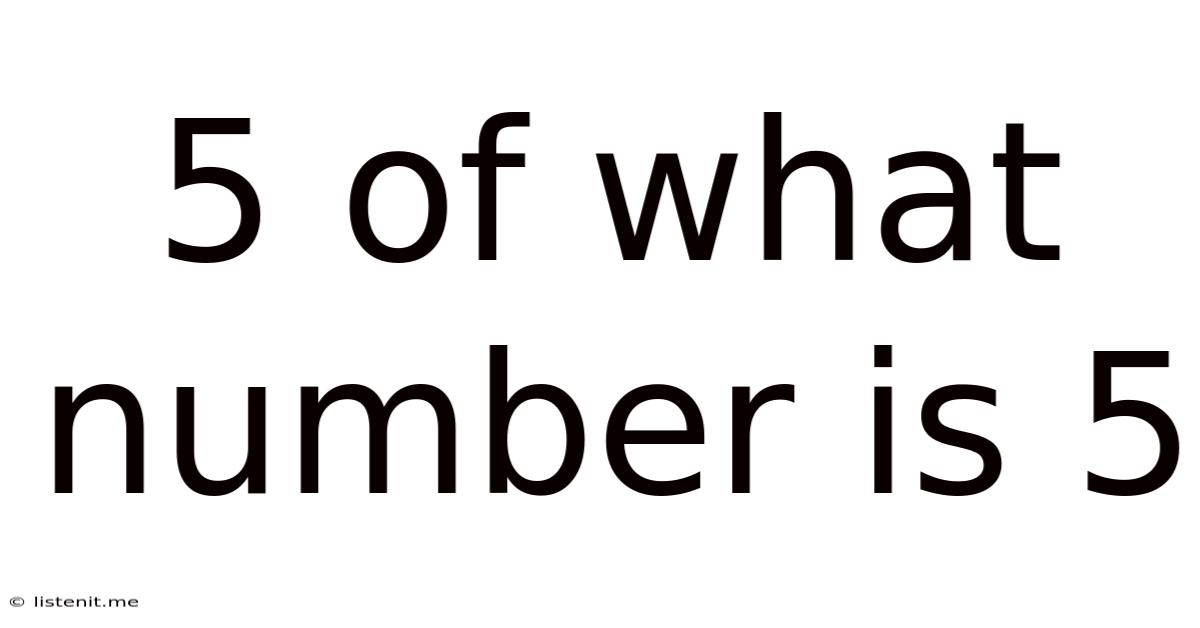
Table of Contents
5 of What Number is 5? Unpacking the Simplicity and Exploring its Mathematical Implications
The question, "5 of what number is 5?" appears deceptively simple. At first glance, the answer seems obvious: the number itself, 5. However, a deeper dive reveals a fascinating exploration of mathematical concepts, including fractions, percentages, and the fundamental nature of multiplicative relationships. This seemingly elementary question provides a springboard to understand more complex mathematical principles and their applications in real-world scenarios.
Understanding the Core Question
The phrase "5 of what number is 5" can be translated into a mathematical equation. We can represent "of" as multiplication. Let's use 'x' to represent the unknown number. Therefore, the question translates to:
5 * (x/100) = 5 (or, more simply, 5 * x = 5 when dealing with whole numbers)
This equation helps us frame the problem and understand the different approaches to finding a solution. Solving for 'x' will provide the answer to our question.
Solution Approaches: A Multifaceted Exploration
We can solve this equation using several methods, each offering a unique perspective on the problem:
Method 1: Direct Division
The simplest approach is to directly divide both sides of the equation (5 * x = 5) by 5. This isolates 'x', giving us:
x = 5 / 5
Therefore, x = 1. In this context, "5 of 1 is 5". This method highlights the fundamental relationship between multiplication and division as inverse operations.
Method 2: Fractional Representation
We can represent "5 of what number is 5" fractionally. "5 of" translates to 5/n where 'n' is the unknown number. The equation becomes:
5/n = 5/1
Cross-multiplying gives us:
5 * 1 = 5 * n
5 = 5n
Dividing both sides by 5 yields:
n = 1
This method demonstrates the power of using fractions to solve problems involving proportions and ratios. It emphasizes the equivalency between fractions and their simplified forms.
Method 3: Percentage Perspective
This problem can also be approached using percentages. If we consider "5 of what number is 5" as a percentage problem, we are asking "5 is what percentage of what number?". We can represent this as:
5 = p% * x
Where 'p' is the percentage and 'x' is the unknown number. Since 5 is 100% of itself, we can set p% = 100%, or 1. The equation then becomes:
5 = 1 * x
Therefore, x = 5. This seemingly contradicts our previous answer. However, this illustrates the importance of precisely defining the context of the problem. Here we're asking what number 5 represents 100% of. The original question, implying a fraction of a number, is different.
The Importance of Context and Precision in Mathematical Problem Solving
The different approaches and resulting answers highlight the critical role of context and precision in mathematical problem-solving. The ambiguity in phrasing the original question ("5 of what number is 5") allows for multiple valid interpretations. Each interpretation leads to a different mathematical equation and a potentially different solution.
This underscores the importance of:
- Clear and unambiguous problem statements: The language used to describe a mathematical problem must be precise to avoid misinterpretations and ensure that all parties understand the problem's requirements.
- Careful definition of variables: Clearly defining what each variable represents prevents confusion and ensures that the solution accurately addresses the problem.
- Understanding the underlying mathematical concepts: A solid understanding of fundamental concepts like fractions, percentages, and the relationships between multiplication and division is crucial for solving problems effectively.
Real-World Applications: Beyond the Classroom
The seemingly trivial nature of this question belies its relevance in real-world situations. Understanding proportional relationships, as explored through the different solution methods, is vital in various fields:
- Business and Finance: Calculating profit margins, determining discounts, and understanding interest rates all rely on the ability to work with proportions and percentages.
- Engineering and Science: Scaling models, calculating concentrations, and understanding ratios are all fundamental to many engineering and scientific applications.
- Everyday Life: Cooking (scaling recipes), shopping (calculating discounts), and even dividing resources amongst friends all involve proportional reasoning.
Extending the Concept: More Complex Variations
Let's consider more complex variations of the original problem to further illustrate the importance of precise phrasing and multiple solution approaches.
Example 1: "5 of what number is 10?"
This problem requires a different approach. The equation becomes:
5 * (x/100) = 10 (or 5x = 10 if dealing with whole numbers)
Solving for x, we get x = 2 (or x = 200%). This shows that 5 is 50% of 10. This problem highlights the flexibility in how the phrase "of what number" can be interpreted depending on the context.
Example 2: "What number multiplied by 5 equals 5?"
This is essentially the original question restated more formally. The equation is straightforward:
5x = 5
x = 1
This underscores the importance of recognizing different ways of expressing the same mathematical concept.
Conclusion: The Enduring Power of Simple Questions
The seemingly simple question, "5 of what number is 5," provides a surprisingly rich learning experience. It showcases the importance of precise language, careful variable definition, and a deep understanding of fundamental mathematical concepts. Moreover, it illustrates the wide applicability of proportional reasoning in real-world scenarios. By exploring this seemingly basic problem, we gain valuable insights into the multifaceted nature of mathematics and its power to solve problems in diverse fields. The seemingly simple can often reveal profound mathematical truths, encouraging us to always approach problems with careful consideration and a keen eye for detail. The next time you encounter a problem that seems too simple, remember the depth hidden within even the most elementary questions. The seemingly basic can often be the most insightful.
Latest Posts
Latest Posts
-
What Is The Similarity Between A Compound And A Mixture
May 10, 2025
-
Is H2s A Acid Or Base
May 10, 2025
-
Soda Fizzing Physical Or Chemical Change
May 10, 2025
-
How Many Sublevels Are In The N 3 Level
May 10, 2025
-
A Substance Made Of One Type Of Atom
May 10, 2025
Related Post
Thank you for visiting our website which covers about 5 Of What Number Is 5 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.