5 Is 5 Of What Number
listenit
May 11, 2025 · 5 min read
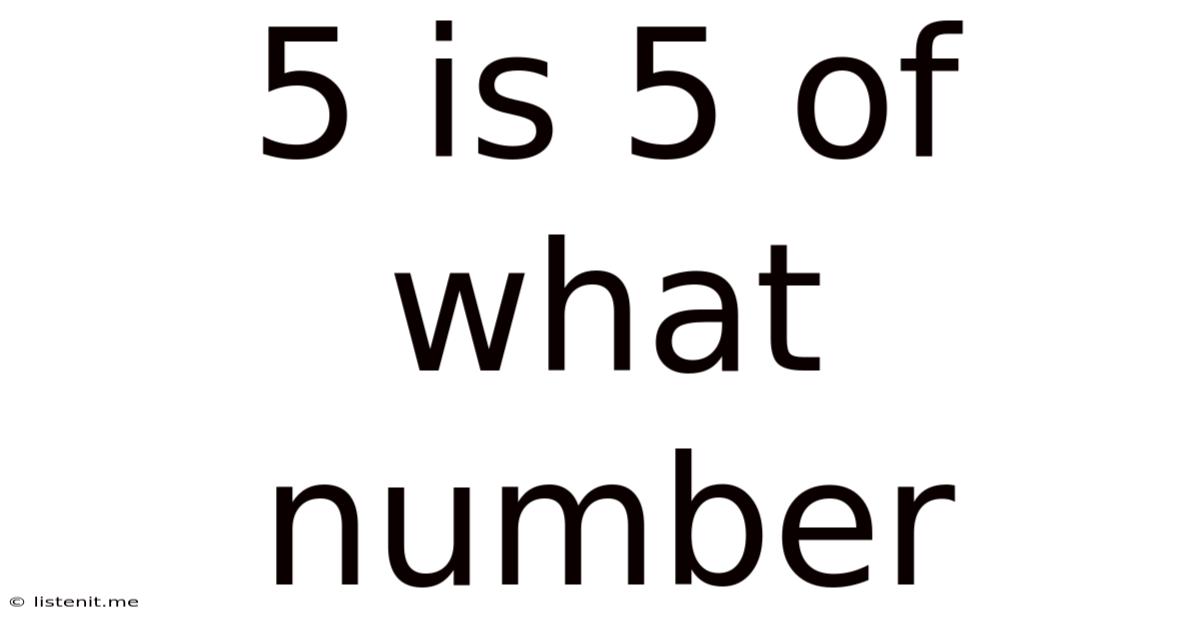
Table of Contents
5 Is 5% of What Number? Unraveling the Math Behind Percentages
Understanding percentages is a fundamental skill in mathematics with widespread applications in everyday life, from calculating discounts and taxes to analyzing financial data and understanding statistical information. This article delves into a specific percentage problem: determining the number of which 5 is 5%. We'll explore the mathematical approach, break down the steps involved, provide practical examples, and discuss the broader implications of percentage calculations.
Understanding the Problem: "5 is 5% of What Number?"
The core question, "5 is 5% of what number?", presents a simple yet crucial concept in percentage calculations. It asks us to find the base number (the whole) from which 5 represents 5%. This type of problem involves working backward from a percentage to find the original value. Many real-world scenarios require this type of calculation, making understanding the solution essential.
The Mathematical Approach: Solving for the Unknown
To solve this problem, we need to translate the word problem into a mathematical equation. The phrase "5 is 5% of what number?" can be expressed as:
5 = 0.05 * x
Where:
- 5 represents the part (the value we're given).
- 0.05 represents the percentage (5% converted to decimal form – divide the percentage by 100).
- x represents the unknown number we need to find (the whole).
To solve for 'x', we use simple algebraic manipulation:
- Divide both sides of the equation by 0.05: This isolates 'x' on one side of the equation.
- Simplify: This gives us the value of 'x'.
Let's perform the calculation:
5 / 0.05 = x
x = 100
Therefore, 5 is 5% of 100.
Step-by-Step Solution with Explanation
Let's break down the solution process into clear, easy-to-follow steps:
Step 1: Convert the Percentage to a Decimal
The first step is to convert the percentage (5%) into its decimal equivalent. To do this, divide the percentage by 100:
5% / 100 = 0.05
Step 2: Set up the Equation
Now, we set up the equation based on the problem statement:
5 = 0.05 * x
This equation represents "5 is 5% (0.05) of what number (x)?"
Step 3: Solve for the Unknown (x)
To solve for 'x', we need to isolate it. We do this by dividing both sides of the equation by 0.05:
5 / 0.05 = x
Step 4: Calculate the Result
Performing the division gives us the answer:
100 = x
Therefore, 5 is 5% of 100.
Practical Applications: Real-World Examples
Understanding percentage calculations like this has numerous practical applications. Here are a few examples:
-
Sales and Discounts: A store offers a 5% discount on an item, and you save $5. Using the method above, you can easily calculate the original price of the item ($100).
-
Taxes: If a 5% sales tax on a purchase amounts to $5, you can determine the pre-tax price of the item ($100).
-
Financial Analysis: In finance, understanding percentages is critical for analyzing investment returns, calculating interest rates, and assessing financial performance. For example, if an investment generates a 5% return and the return is $5, the initial investment was $100.
-
Statistical Analysis: Percentages are frequently used in statistics to represent proportions and probabilities. For instance, if 5% of a sample population exhibits a certain characteristic and that number is 5 individuals, the total sample size is 100.
Expanding the Concept: Solving More Complex Percentage Problems
While the example above is relatively straightforward, the same principles can be applied to more complex percentage problems. For example:
-
Finding a Percentage of a Number: If you want to find 15% of 200, you would multiply 200 by 0.15 (15/100).
-
Finding the Percentage Increase or Decrease: To calculate the percentage increase from 100 to 120, first find the difference (20), then divide by the original number (100) and multiply by 100% to get 20%.
-
Working with Multiple Percentages: Problems involving compound interest or successive discounts require understanding how to combine multiple percentages.
Importance of Percentage Calculations in Various Fields
Percentage calculations are essential in various fields, including:
-
Business and Finance: Profit margins, sales growth, investment returns, and loan interest calculations all rely on percentage calculations.
-
Science: Many scientific calculations involve percentages, such as calculating concentrations of solutions, experimental error, or statistical analysis.
-
Engineering: Engineers use percentages in designing structures, materials testing, and quality control.
-
Healthcare: Healthcare professionals use percentages in dosage calculations, analyzing health statistics, and interpreting medical test results.
Troubleshooting Common Mistakes in Percentage Calculations
Several common errors can occur when performing percentage calculations. These include:
-
Incorrect Decimal Conversion: Failing to correctly convert percentages to decimals is a frequent mistake. Always remember to divide the percentage by 100 before using it in calculations.
-
Mixing up the Parts: Make sure you correctly identify the "part," "whole," and "percentage" in the problem before setting up your equation.
-
Calculation Errors: Double-check your calculations, especially when dealing with multiple steps or complex percentages.
Advanced Techniques and Resources for Percentage Mastery
For those seeking a deeper understanding of percentages, here are some avenues to explore:
-
Online Calculators: Many free online calculators can help you check your work and solve more complex percentage problems.
-
Educational Websites and Videos: Numerous online resources offer tutorials and practice exercises on percentage calculations.
-
Textbooks and Workbooks: Mathematics textbooks and workbooks provide comprehensive coverage of percentages and related topics.
Conclusion: Mastering Percentages for Everyday Success
The seemingly simple question, "5 is 5% of what number?", highlights the fundamental importance of understanding percentage calculations. This skill is vital for navigating many aspects of life, from personal finances to professional endeavors. By mastering the techniques and avoiding common pitfalls, you can confidently tackle a wide range of percentage problems and apply this knowledge to solve real-world challenges. The ability to perform accurate percentage calculations empowers you to make informed decisions, analyze data effectively, and achieve greater success in various aspects of your life.
Latest Posts
Latest Posts
-
Oxidation Number Of Hydrogen In H2o
May 11, 2025
-
Gold Which Has A Density Of 19 32
May 11, 2025
-
Aldol Condensation Of Benzaldehyde And Acetone
May 11, 2025
-
What Was The Religion Of Colonial Virginia
May 11, 2025
-
What Is The Correct Formula For The Iron Ii Ion
May 11, 2025
Related Post
Thank you for visiting our website which covers about 5 Is 5 Of What Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.