5/7 And 2/7 Expressed As A Ratio Is
listenit
May 25, 2025 · 5 min read
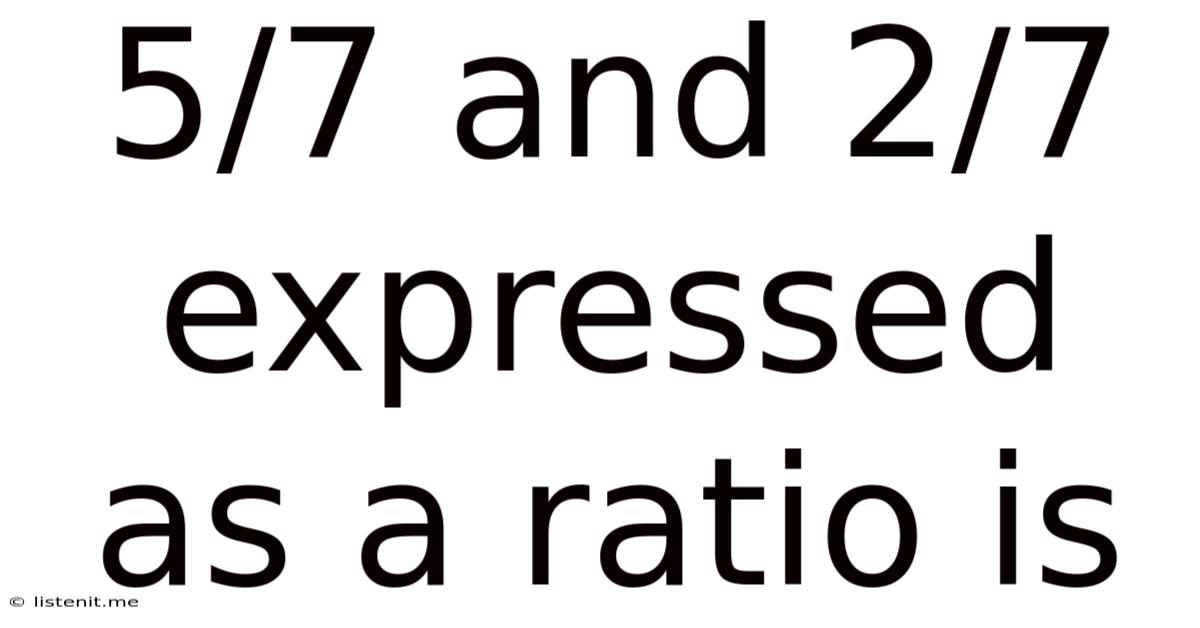
Table of Contents
5/7 and 2/7 Expressed as a Ratio: A Deep Dive into Ratios, Proportions, and Their Applications
The seemingly simple question of expressing 5/7 and 2/7 as a ratio opens a door to a fascinating world of mathematical concepts with wide-ranging applications. This article will not only answer the initial question but will also delve into the underlying principles of ratios and proportions, exploring their significance in various fields and providing practical examples to solidify your understanding.
Understanding Ratios
A ratio is a comparison of two or more quantities. It shows the relative size of one quantity compared to another. Ratios can be expressed in several ways:
- Using the colon (:): For example, the ratio of 5/7 to 2/7 can be written as 5/7 : 2/7.
- Using the word "to": The ratio can also be expressed as "5/7 to 2/7".
- As a fraction: The most common and often preferred method is to express the ratio as a fraction: (5/7) / (2/7).
Calculating the Ratio of 5/7 and 2/7
To express 5/7 and 2/7 as a ratio, we'll use the fraction method:
(5/7) / (2/7)
Remember that dividing by a fraction is the same as multiplying by its reciprocal. Therefore:
(5/7) * (7/2) = 35/14
This fraction can be simplified by dividing both the numerator and the denominator by their greatest common divisor, which is 7:
35/14 = 5/2
Therefore, the ratio of 5/7 and 2/7 is 5:2 or 5/2. This signifies that for every 5 parts of the first quantity (5/7), there are 2 parts of the second quantity (2/7).
Ratios vs. Fractions: Key Differences
While ratios and fractions might seem interchangeable, there's a subtle but important distinction:
- Fractions represent a part of a whole. For instance, 5/7 represents 5 out of 7 equal parts of a single entity.
- Ratios compare two or more quantities. They don't necessarily represent parts of a whole; they simply show the relative sizes of different quantities.
Proportions: Extending the Concept
A proportion is a statement that two ratios are equal. It's a powerful tool for solving problems involving ratios. If we have a proportion:
a/b = c/d
Then we can cross-multiply to find unknown values:
a * d = b * c
This property is extremely useful in various applications.
Real-World Applications of Ratios and Proportions
Ratios and proportions are fundamental concepts with applications across numerous fields:
1. Scaling and Modeling:
Architects and engineers use ratios and proportions to create scaled models of buildings and structures. A 1:100 scale model means that 1 unit on the model represents 100 units in the actual structure.
2. Recipe Scaling:
Cooking frequently involves scaling recipes up or down. If a recipe calls for a 2:1 ratio of flour to sugar, you can use proportions to adjust the amounts for a larger or smaller batch.
3. Mapmaking:
Maps use ratios and scales to represent distances. A scale of 1:100,000 indicates that 1 unit on the map represents 100,000 units on the ground.
4. Finance and Investing:
Ratios like the debt-to-equity ratio or the price-to-earnings ratio are crucial in financial analysis to assess the financial health and performance of companies.
5. Science and Chemistry:
In chemistry, ratios are essential for determining the proportions of reactants in chemical reactions. Stoichiometry, the study of quantitative relationships in chemical reactions, heavily relies on ratios.
6. Medicine and Pharmacology:
Dosage calculations in medicine frequently utilize ratios and proportions to determine the correct amount of medication based on a patient's weight or other factors.
7. Image Resolution and Aspect Ratio:
In digital imaging, the aspect ratio (e.g., 16:9) defines the proportional relationship between the width and height of an image.
8. Probability and Statistics:
Ratios are fundamental to probability calculations. The ratio of favorable outcomes to the total number of possible outcomes determines the probability of an event.
Advanced Concepts: Continued Proportions and Compound Ratios
Let's briefly touch upon more advanced aspects of ratios:
Continued Proportions: A continued proportion is when three or more ratios are equal. For example:
a/b = b/c = c/d
Compound Ratios: A compound ratio is the ratio of two or more ratios multiplied together. For example, if we have ratios a:b and c:d, their compound ratio is (ac) : (bd).
Solving Problems with Ratios and Proportions: Examples
Let's look at some example problems to illustrate the practical application of these concepts:
Example 1: A recipe calls for 3 cups of flour and 2 cups of sugar. If you want to make a larger batch using 6 cups of flour, how many cups of sugar will you need?
- Solution: Set up a proportion: 3/2 = 6/x. Cross-multiply to solve for x: 3x = 12, so x = 4 cups of sugar.
Example 2: A map has a scale of 1:50,000. If the distance between two points on the map is 5 cm, what is the actual distance between the points?
- Solution: Set up a proportion: 1/50000 = 5/x. Cross-multiply: x = 250,000 cm = 2.5 km
Example 3: A mixture contains 5 parts water and 2 parts concentrate. How many parts of concentrate are needed if you have 15 parts of water?
- Solution: Set up a proportion: 5/2 = 15/x. Cross-multiply: 5x = 30, so x = 6 parts of concentrate.
Conclusion: The Ubiquity of Ratios and Proportions
From the simple task of scaling a recipe to complex calculations in engineering and finance, ratios and proportions are fundamental mathematical tools with far-reaching applications. Understanding these concepts is crucial for problem-solving in a wide range of disciplines. This article has not only answered the initial question about expressing 5/7 and 2/7 as a ratio (5:2 or 5/2) but has also provided a comprehensive overview of these important mathematical concepts and their diverse applications, empowering you to tackle various ratio-related problems with confidence. Remember to practice applying these concepts through various examples to strengthen your understanding and build problem-solving skills.
Latest Posts
Latest Posts
-
Do 1 2 And 1 4 Equal 3 4
May 25, 2025
-
What Is The Gcf Of 32 And 81
May 25, 2025
-
Greatest Common Factor Of 40 And 56
May 25, 2025
-
What Day Is It 3 Weeks From Now
May 25, 2025
-
Traceloans Com Business Loans For Startups
May 25, 2025
Related Post
Thank you for visiting our website which covers about 5/7 And 2/7 Expressed As A Ratio Is . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.