4 Out Of 14 As A Percentage
listenit
May 12, 2025 · 5 min read
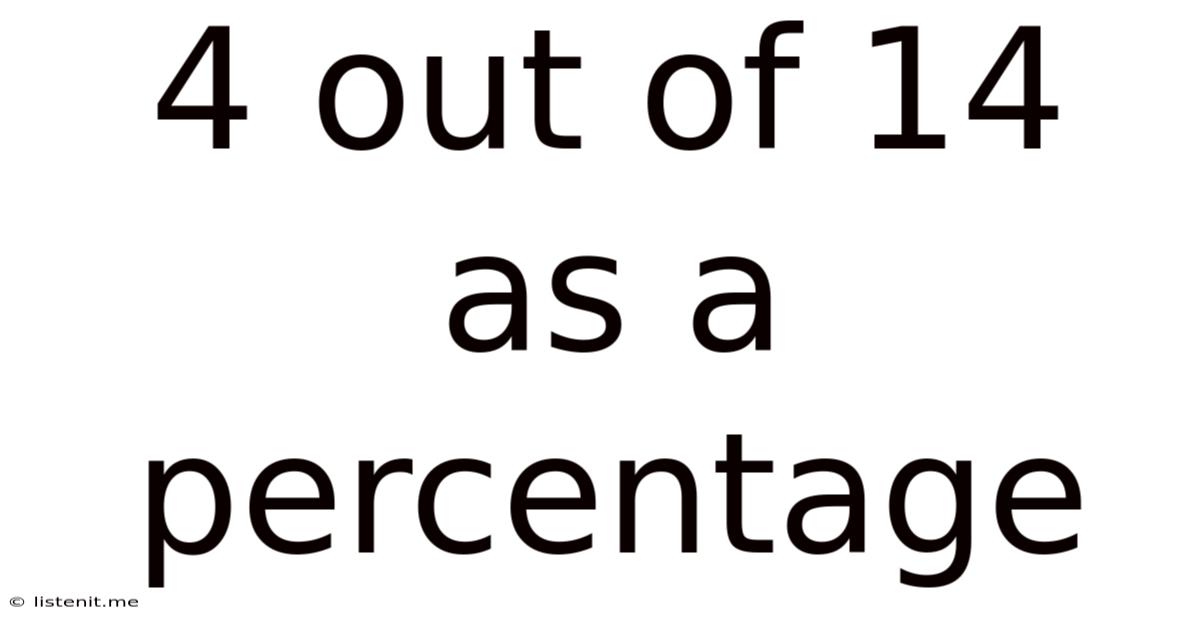
Table of Contents
4 Out of 14 as a Percentage: A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and taxes to analyzing data and understanding statistics. This article delves into the specific calculation of "4 out of 14 as a percentage," providing a step-by-step explanation, practical applications, and related concepts to solidify your understanding. We'll explore different methods, address common misconceptions, and show how to apply this knowledge in real-world scenarios.
Understanding the Fundamentals of Percentages
Before diving into the specific calculation, let's refresh our understanding of percentages. A percentage is simply a fraction expressed as a part of 100. The symbol "%" represents "per hundred" or "out of 100." For example, 50% means 50 out of 100, or 50/100, which simplifies to 1/2.
Key Terms:
- Numerator: The top number in a fraction (in our case, 4). This represents the part.
- Denominator: The bottom number in a fraction (in our case, 14). This represents the whole.
- Percentage: The fraction expressed as a number out of 100.
Calculating 4 out of 14 as a Percentage: Method 1 - Direct Calculation
The most straightforward method to calculate 4 out of 14 as a percentage involves converting the fraction to a decimal and then multiplying by 100.
Steps:
- Form the Fraction: Express the problem as a fraction: 4/14.
- Simplify the Fraction (Optional): While not strictly necessary, simplifying the fraction can make the subsequent calculation easier. In this case, both the numerator and denominator are divisible by 2, simplifying the fraction to 2/7.
- Convert to Decimal: Divide the numerator by the denominator: 2 ÷ 7 ≈ 0.2857.
- Multiply by 100: Multiply the decimal by 100 to convert it to a percentage: 0.2857 x 100 = 28.57%.
Therefore, 4 out of 14 is approximately 28.57%.
Calculating 4 out of 14 as a Percentage: Method 2 - Using Proportions
Another approach involves setting up a proportion. This method is particularly helpful for visualizing the relationship between the parts and the whole.
Steps:
- Set up a Proportion: We want to find the percentage (x) that corresponds to 4 out of 14. We can set up a proportion: 4/14 = x/100.
- Cross-Multiply: Cross-multiply the terms: 4 * 100 = 14 * x. This simplifies to 400 = 14x.
- Solve for x: Divide both sides by 14 to isolate x: x = 400/14 ≈ 28.57.
- Express as Percentage: The result (28.57) represents the percentage, so 4 out of 14 is approximately 28.57%.
This method reinforces the understanding that percentages represent a ratio out of 100.
Rounding and Precision
In the calculations above, we obtained 28.57%. The level of precision depends on the context. For many applications, rounding to the nearest whole number (29%) might suffice. However, for more precise calculations, retaining the decimal places (28.57%) is necessary. Always consider the required level of accuracy when rounding.
Real-World Applications of Percentage Calculations
Understanding percentage calculations has numerous real-world applications, including:
- Financial Calculations: Calculating discounts, interest rates, taxes, profits, and losses. For example, if a store offers a 20% discount on an item, you can easily determine the final price after the discount is applied.
- Data Analysis: Interpreting statistical data, such as survey results, test scores, and market research findings. For instance, if 4 out of 14 people surveyed prefer a particular product, you can express this preference as a percentage (28.57%).
- Academic Performance: Calculating grades, assessing progress in studies, and understanding performance relative to others. For example, if you answered 4 out of 14 questions correctly on a quiz, you can calculate your percentage score.
- Scientific Research: Expressing experimental results, analyzing data, and drawing conclusions based on percentages.
- Everyday Life: Calculating tips in restaurants, understanding sales promotions, and many more everyday situations require a basic understanding of percentages.
Common Mistakes to Avoid
While percentage calculations are relatively straightforward, several common mistakes can lead to inaccurate results:
- Incorrect Fraction Formation: Ensure you correctly identify the numerator (the part) and the denominator (the whole) before forming the fraction.
- Division Errors: Double-check your division when converting the fraction to a decimal. Using a calculator can help minimize errors.
- Rounding Errors: Be mindful of the required level of precision and round appropriately. Avoid premature rounding during the calculation process.
- Misunderstanding the Context: Always carefully read the problem statement to ensure you're correctly identifying the relevant parts and the whole.
Expanding on Percentage Concepts: Ratios and Proportions
Understanding percentages is intrinsically linked to ratios and proportions. A ratio is a comparison of two quantities, often expressed as a fraction. A proportion is a statement that two ratios are equal. In our example, the ratio of 4 to 14 can be expressed as 4:14 or 4/14. We used a proportion to solve the percentage calculation using Method 2.
Percentage Increase and Decrease
Percentage calculations often involve determining percentage increases or decreases. This is commonly seen in situations involving price changes, population growth, or economic indicators. Understanding how to calculate these changes is crucial for accurate analysis and prediction.
Example of Percentage Increase: If the price of an item increased from $10 to $14, the percentage increase can be calculated as follows:
- Find the difference: $14 - $10 = $4
- Divide the difference by the original amount: $4 / $10 = 0.4
- Multiply by 100: 0.4 * 100 = 40%
The price increased by 40%.
Example of Percentage Decrease: If the price of an item decreased from $14 to $10, the percentage decrease can be calculated similarly:
- Find the difference: $14 - $10 = $4
- Divide the difference by the original amount: $4 / $14 ≈ 0.2857
- Multiply by 100: 0.2857 * 100 ≈ 28.57%
The price decreased by approximately 28.57%.
Conclusion: Mastering Percentage Calculations
Understanding how to calculate percentages, especially simple problems like "4 out of 14 as a percentage," is a valuable skill with wide-ranging applications. By mastering the methods outlined in this article and understanding the underlying concepts of fractions, ratios, and proportions, you'll be well-equipped to tackle various percentage-related calculations confidently and accurately in your personal and professional life. Remember to pay attention to detail, avoid common mistakes, and choose the method that best suits your needs and understanding. Practice makes perfect, so work through several examples to solidify your grasp of this fundamental mathematical concept.
Latest Posts
Latest Posts
-
How To Solve For 3 Variables With 3 Equations
May 13, 2025
-
Is Silver A Solid Liquid Or Gas
May 13, 2025
-
What Is 1 3 8 In Fraction
May 13, 2025
-
Composed Of Two Or More Elements
May 13, 2025
-
Visible Light Comes From Which One Of The Suns Layers
May 13, 2025
Related Post
Thank you for visiting our website which covers about 4 Out Of 14 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.