3x 2y 8 In Slope Intercept Form
listenit
May 10, 2025 · 5 min read
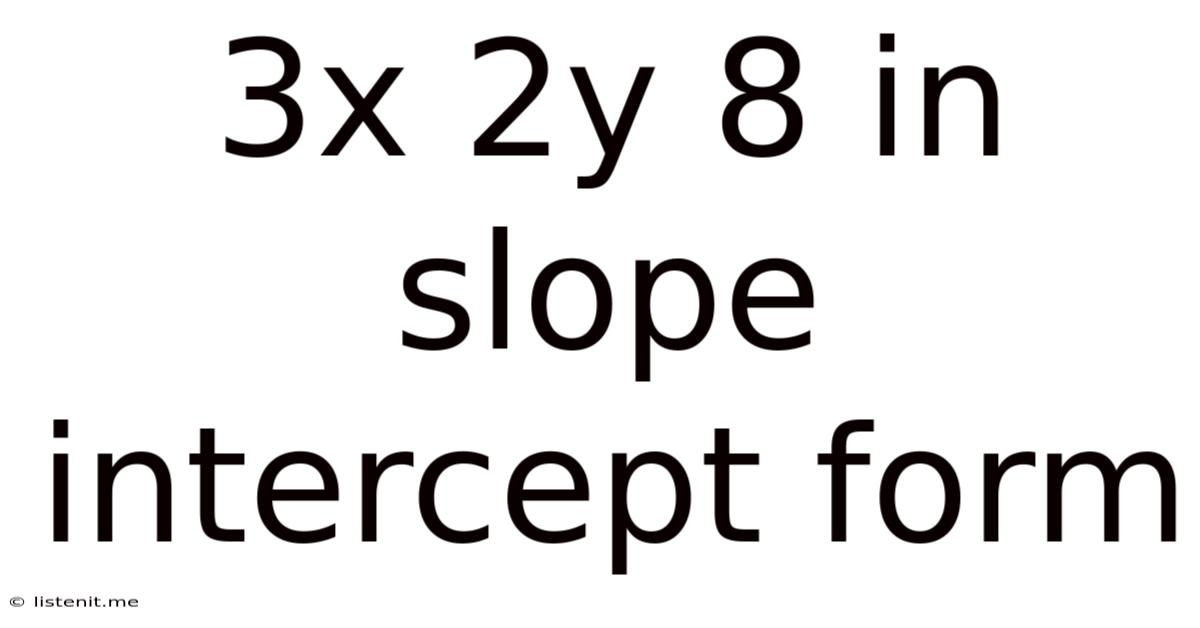
Table of Contents
Converting 3x + 2y = 8 to Slope-Intercept Form: A Comprehensive Guide
The equation 3x + 2y = 8 represents a straight line. While useful in its current form, converting it to slope-intercept form (y = mx + b) offers significant advantages for understanding and visualizing the line's properties. This form readily reveals the line's slope (m) and y-intercept (b), crucial pieces of information for various mathematical applications and real-world problems. This comprehensive guide will walk you through the process step-by-step, exploring the underlying concepts, and providing practical examples.
Understanding Slope-Intercept Form (y = mx + b)
Before diving into the conversion, let's refresh our understanding of the slope-intercept form: y = mx + b.
- y: Represents the dependent variable, typically plotted on the vertical axis.
- x: Represents the independent variable, typically plotted on the horizontal axis.
- m: Represents the slope of the line. The slope indicates the steepness and direction of the line. A positive slope means the line rises from left to right, while a negative slope means the line falls from left to right. A slope of zero indicates a horizontal line, and an undefined slope indicates a vertical line. The slope is calculated as the change in y divided by the change in x (rise over run).
- b: Represents the y-intercept, which is the point where the line intersects the y-axis (where x = 0).
Converting 3x + 2y = 8 to Slope-Intercept Form
Our goal is to manipulate the equation 3x + 2y = 8 to isolate 'y' on one side of the equation, resulting in the y = mx + b form. Here's how we do it:
-
Subtract 3x from both sides:
This step removes the '3x' term from the left side, leaving only the '2y' term.
3x + 2y - 3x = 8 - 3x
This simplifies to:
2y = -3x + 8
-
Divide both sides by 2:
This step isolates 'y', giving us the desired slope-intercept form.
2y / 2 = (-3x + 8) / 2
This simplifies to:
y = (-3/2)x + 4
Therefore, the equation 3x + 2y = 8 in slope-intercept form is y = (-3/2)x + 4.
Interpreting the Slope and Y-Intercept
Now that we have the equation in slope-intercept form, we can easily identify the slope and y-intercept:
-
Slope (m) = -3/2: This indicates a negative slope. The line slopes downwards from left to right. The slope's numerical value (-3/2 or -1.5) represents the rate of change of y with respect to x. For every 2 units increase in x, y decreases by 3 units.
-
Y-intercept (b) = 4: This means the line intersects the y-axis at the point (0, 4).
Graphing the Line
With the slope and y-intercept, graphing the line is straightforward:
-
Plot the y-intercept: Start by plotting the point (0, 4) on the y-axis.
-
Use the slope to find another point: The slope is -3/2. This means from the y-intercept (0, 4), move 2 units to the right (positive x-direction) and 3 units down (negative y-direction). This gives us the point (2, 1).
-
Draw the line: Draw a straight line passing through the points (0, 4) and (2, 1). This line represents the equation 3x + 2y = 8.
Applications and Real-World Examples
The slope-intercept form has numerous applications in various fields:
-
Physics: Describing the motion of objects with constant velocity (where slope represents velocity and y-intercept represents initial position).
-
Economics: Modeling supply and demand curves (slope represents the change in quantity demanded or supplied with respect to price).
-
Engineering: Designing ramps and inclines (slope represents the angle of inclination).
-
Finance: Analyzing investment growth or decline (slope represents the rate of return).
-
Computer graphics: Representing lines and other geometric shapes.
Advanced Concepts and Related Topics
While the conversion process is relatively straightforward, understanding related concepts provides a deeper appreciation for the significance of slope-intercept form:
-
Standard Form: The equation 3x + 2y = 8 is in standard form (Ax + By = C). Converting between standard and slope-intercept forms is a fundamental skill in algebra.
-
Point-Slope Form: This form (y - y1 = m(x - x1)) is useful when you know the slope and a point on the line.
-
Parallel and Perpendicular Lines: The slope plays a crucial role in determining whether two lines are parallel (same slope) or perpendicular (slopes are negative reciprocals).
-
Linear Inequalities: The slope-intercept form can be extended to represent linear inequalities (e.g., y > (-3/2)x + 4), which are useful for modeling constraints or regions in a plane.
-
Systems of Linear Equations: Solving systems of linear equations often involves graphing lines in slope-intercept form to find points of intersection.
Solving Problems Involving the Equation
Let's consider some example problems involving the equation y = (-3/2)x + 4:
Problem 1: Find the value of y when x = 2.
Substitute x = 2 into the equation:
y = (-3/2)(2) + 4 = -3 + 4 = 1
Therefore, when x = 2, y = 1.
Problem 2: Find the value of x when y = 7.
Substitute y = 7 into the equation and solve for x:
7 = (-3/2)x + 4
3 = (-3/2)x
x = 3 * (-2/3) = -2
Therefore, when y = 7, x = -2.
Problem 3: Determine if the point (4, -2) lies on the line.
Substitute x = 4 and y = -2 into the equation:
-2 = (-3/2)(4) + 4
-2 = -6 + 4
-2 = -2
Since the equation holds true, the point (4, -2) lies on the line.
Conclusion
Converting the equation 3x + 2y = 8 to slope-intercept form (y = (-3/2)x + 4) provides valuable insights into the line's properties, including its slope and y-intercept. This form simplifies graphing, problem-solving, and understanding the line's behavior within various mathematical and real-world contexts. By mastering this conversion and grasping the related concepts, you'll significantly enhance your algebraic skills and ability to analyze linear relationships. This comprehensive guide has equipped you with the knowledge to confidently tackle problems involving this fundamental equation and its various applications. Remember to practice regularly to solidify your understanding and build proficiency.
Latest Posts
Latest Posts
-
How Do You Write 7 9 As A Decimal
May 10, 2025
-
What Is The Period Of Tan X
May 10, 2025
-
Is Souring Of Milk A Chemical Change
May 10, 2025
-
Is 2 Pi Rational Or Irrational
May 10, 2025
-
A Container Of N2o3 Has A Pressure Of
May 10, 2025
Related Post
Thank you for visiting our website which covers about 3x 2y 8 In Slope Intercept Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.