30 Is 12 Percent Of What Number
listenit
May 25, 2025 · 4 min read
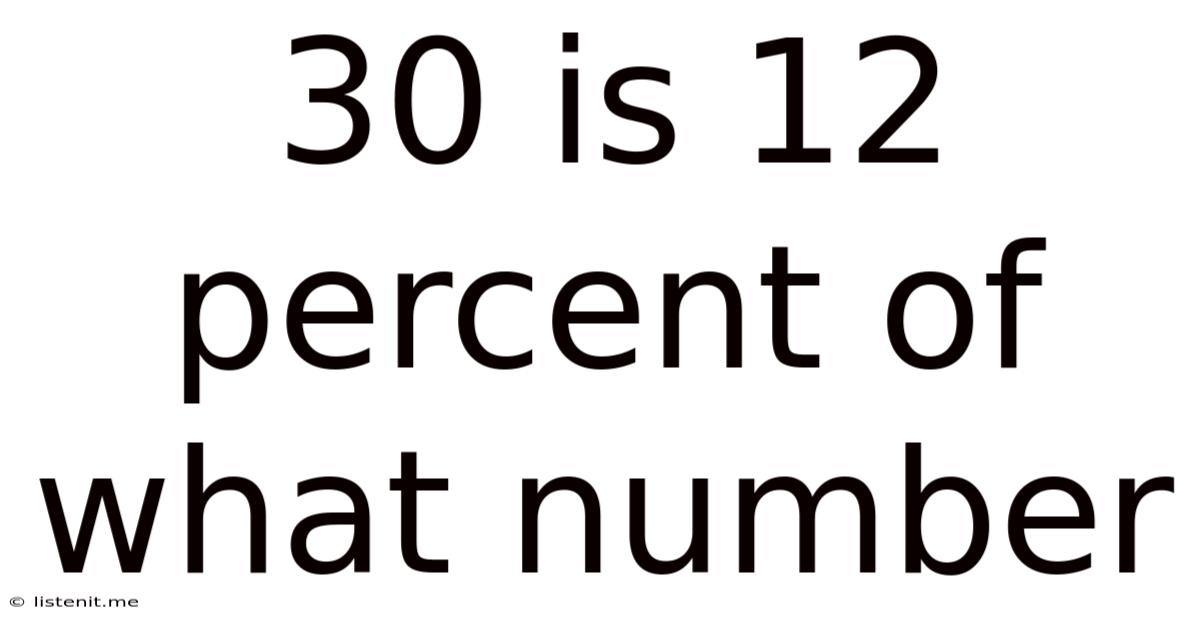
Table of Contents
30 is 12 Percent of What Number: A Comprehensive Guide to Percentage Calculations
Solving percentage problems is a fundamental skill applicable across various fields, from everyday budgeting to complex financial analysis. Understanding how to calculate percentages is crucial for making informed decisions and interpreting data effectively. This article delves into the question, "30 is 12 percent of what number?", providing a detailed explanation of the solution, exploring different approaches, and offering practical applications to enhance your understanding of percentage calculations.
Understanding the Problem: Deconstructing the Percentage Equation
The problem "30 is 12 percent of what number?" essentially presents a percentage equation where we need to find the unknown base value. Let's break down the elements:
- 30: This is the resulting value (or part) of the whole.
- 12%: This represents the percentage or the fraction of the whole.
- What number?: This is the unknown value we need to determine – the whole or the base number.
To solve this, we can translate the problem into a mathematical equation:
30 = 0.12 * x
Where 'x' represents the unknown number we're trying to find.
Method 1: Solving the Equation Algebraically
This is the most straightforward approach. We need to isolate 'x' to find its value. Here's how:
-
Convert the percentage to a decimal: 12% is equivalent to 0.12 (divide 12 by 100).
-
Substitute into the equation: Our equation now becomes: 30 = 0.12 * x
-
Isolate x: To solve for 'x', divide both sides of the equation by 0.12:
x = 30 / 0.12
-
Calculate the result: Performing the division, we find that:
x = 250
Therefore, 30 is 12 percent of 250.
Method 2: Using Proportions
Proportions offer another effective way to solve percentage problems. We can set up a proportion based on the given information:
30 / x = 12 / 100
This proportion states that the ratio of the part (30) to the whole (x) is equal to the ratio of the percentage (12) to 100 (the whole percentage).
To solve this proportion:
-
Cross-multiply: Multiply 30 by 100 and 12 by x:
30 * 100 = 12 * x
-
Simplify: This gives us:
3000 = 12x
-
Isolate x: Divide both sides by 12:
x = 3000 / 12
-
Calculate the result:
x = 250
Again, we arrive at the same solution: 30 is 12 percent of 250.
Method 3: Using the Percentage Formula
The basic percentage formula is:
(Part / Whole) * 100 = Percentage
In our case, we know the part (30) and the percentage (12%). We need to find the whole. Let's rearrange the formula:
Whole = (Part / Percentage) * 100
Substituting the known values:
Whole = (30 / 12) * 100
Whole = 2.5 * 100
Whole = 250
This method provides the same answer, confirming that 30 is 12 percent of 250.
Practical Applications of Percentage Calculations
Understanding percentage calculations is essential in various real-world situations:
1. Finance and Budgeting:
- Calculating interest: Determining interest earned on savings accounts or interest paid on loans relies heavily on percentage calculations.
- Analyzing financial statements: Understanding profit margins, revenue growth, and expense ratios all involve percentage analysis.
- Budgeting: Allocating funds based on percentages of income helps manage personal or business finances effectively. For example, determining how much to allocate towards savings (e.g., 10% of your income) requires percentage calculations.
2. Sales and Marketing:
- Calculating discounts: Determining the final price after a percentage discount is a common application.
- Analyzing sales performance: Tracking sales growth, conversion rates, and customer acquisition costs often uses percentages.
- Setting marketing budgets: Allocating resources to different marketing channels based on their projected return on investment involves percentage calculations.
3. Science and Research:
- Analyzing data: Many scientific experiments involve collecting and analyzing data expressed as percentages. For example, calculating the percentage of successful trials or the percentage change in a measured variable.
- Interpreting statistical results: Statistical analysis often involves calculating percentages to represent the significance of results.
4. Everyday Life:
- Calculating tips: Determining the appropriate tip amount in a restaurant or for services rendered.
- Understanding sales tax: Calculating the final price including sales tax.
- Comparing prices: Determining which product offers the best value by comparing prices and discounts.
Tips and Tricks for Mastering Percentage Calculations
- Practice Regularly: The more you practice, the more confident and efficient you'll become.
- Understand the Fundamentals: A solid grasp of basic arithmetic is crucial for mastering percentage calculations.
- Use Multiple Methods: Trying different approaches helps you develop a deeper understanding and verify your answers.
- Utilize Calculators and Software: Tools like calculators and spreadsheet software can simplify complex calculations.
- Check Your Work: Always double-check your calculations to ensure accuracy.
Conclusion: The Power of Percentage Understanding
The ability to solve percentage problems, as demonstrated by the example "30 is 12 percent of what number?", is a powerful skill that extends far beyond simple mathematical exercises. It's a crucial tool for navigating financial decisions, analyzing data, understanding sales and marketing metrics, and many other aspects of daily life and professional pursuits. Mastering percentage calculations empowers you to make informed decisions, interpret information critically, and ultimately, succeed in various endeavors. By understanding the different methods and applying the tips provided, you can confidently tackle percentage problems and unlock their practical value.
Latest Posts
Latest Posts
-
30 Days From April 27 2024
May 25, 2025
-
Between 3 4 And 7 8
May 25, 2025
-
The Sum Of 7 2 And A Number Is 10
May 25, 2025
-
11 Out Of 28 As A Percentage
May 25, 2025
-
How Do You Find The Volume Of A Room
May 25, 2025
Related Post
Thank you for visiting our website which covers about 30 Is 12 Percent Of What Number . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.