3 Gallon And 5 Gallon Bucket Riddle
listenit
May 10, 2025 · 6 min read
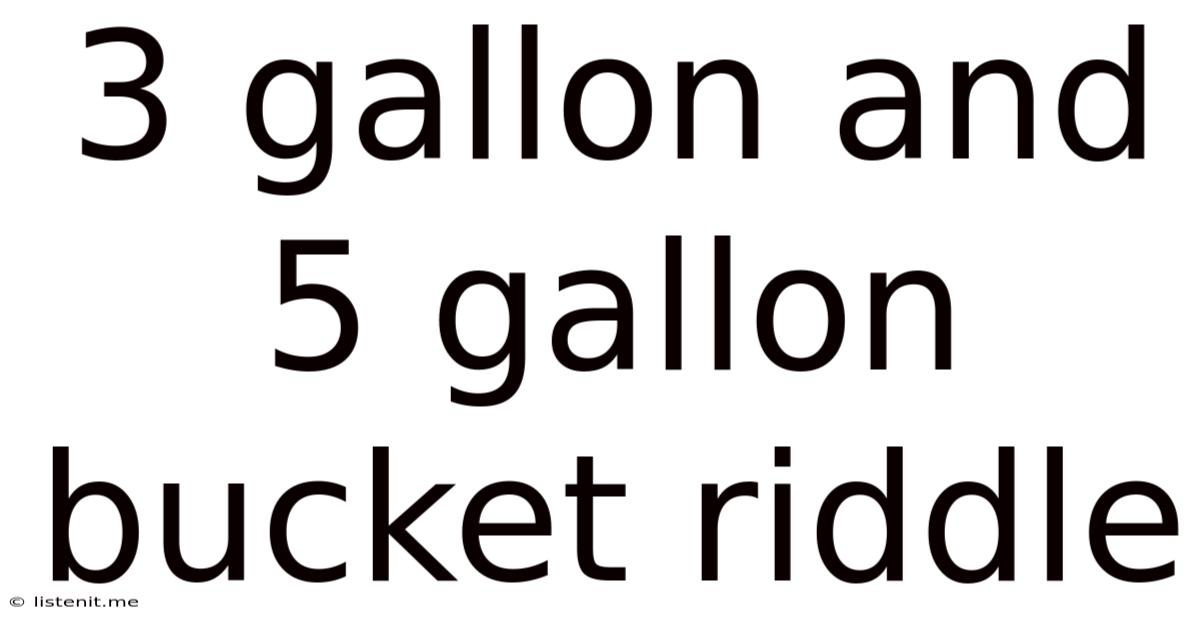
Table of Contents
3 Gallon and 5 Gallon Bucket Riddle: A Deep Dive into Problem-Solving
The classic "3 gallon and 5 gallon bucket riddle" is more than just a brain teaser; it's a microcosm of problem-solving, critical thinking, and the power of methodical approaches. This seemingly simple puzzle, often posed as a water-measuring challenge, has captivated minds for generations, revealing valuable insights into logical reasoning and strategic planning. This comprehensive guide delves into the riddle itself, explores various solutions, analyzes the underlying mathematical principles, and examines its applications in broader contexts.
Understanding the Riddle
The core of the riddle presents a straightforward scenario: you have two buckets, one with a 3-gallon capacity and the other with a 5-gallon capacity. There's an unlimited supply of water. The challenge is to precisely measure out exactly 4 gallons of water using only these two buckets. You can fill a bucket completely, empty a bucket completely, or pour water from one bucket into another until either the source bucket is empty or the receiving bucket is full. No other measuring tools are allowed.
This seemingly simple setup often leads to initial frustration, as intuitive approaches may fail to yield the desired 4 gallons. The key to success lies in a systematic and methodical approach, embracing trial and error while learning from each attempt.
Solving the Riddle: A Step-by-Step Approach
Several solutions exist, showcasing the riddle's flexibility and the multiple pathways to a correct answer. Here's one of the most common and efficient solutions:
Step 1: Fill the 5-gallon bucket completely.
Step 2: Pour water from the 5-gallon bucket into the 3-gallon bucket until it's full. This leaves 2 gallons in the 5-gallon bucket.
Step 3: Empty the 3-gallon bucket.
Step 4: Pour the 2 gallons from the 5-gallon bucket into the empty 3-gallon bucket.
Step 5: Fill the 5-gallon bucket completely.
Step 6: Carefully pour water from the 5-gallon bucket into the 3-gallon bucket (which already contains 2 gallons) until the 3-gallon bucket is full. This will require 1 gallon from the 5-gallon bucket, leaving exactly 4 gallons in the 5-gallon bucket.
Success! You have precisely 4 gallons of water.
Alternative Solutions and Variations
The beauty of this riddle lies in its multiple solutions. While the above method is efficient, alternative approaches can also achieve the same result. Experimentation is key to discovering these variations. For example, you could start by filling the 3-gallon bucket, then transferring its contents to the 5-gallon bucket, and repeating the process. Through careful manipulations of filling, emptying, and transferring, you can arrive at the 4-gallon target.
The riddle can also be modified:
-
Different Bucket Sizes: The challenge can be adapted using different bucket capacities, increasing the difficulty and requiring a more complex strategic approach. For example, using a 7-gallon and a 11-gallon bucket requires a more sophisticated solution.
-
Different Target Amounts: Instead of 4 gallons, the riddle can be adjusted to target other specific amounts within the combined capacity of the buckets (8 gallons in this case). This expands the problem-solving aspect, requiring a flexible approach adaptable to varying targets.
-
Multiple Constraints: Adding further constraints, such as limiting the number of pours or using only specific pouring methods, dramatically enhances the challenge and encourages creative problem-solving.
The Mathematical Underpinnings
At its core, the 3-gallon and 5-gallon bucket riddle involves basic arithmetic and algebraic principles. The solution relies on finding a combination of adding and subtracting quantities to reach the target value of 4 gallons. This involves understanding the relationships between the bucket capacities and the target amount, employing a systematic trial-and-error method to discover a successful combination of operations.
The riddle implicitly introduces the concept of linear Diophantine equations, a branch of number theory dealing with linear equations where solutions must be integers. While not explicitly required to solve the riddle at this level, understanding this mathematical background provides a deeper appreciation of its underlying complexity.
Beyond the Bucket: Applications in Real-World Problem Solving
The skills honed by tackling the 3-gallon and 5-gallon bucket riddle extend far beyond the realm of simple puzzles. The systematic approach, attention to detail, and methodical trial-and-error process are transferable to numerous real-world scenarios:
-
Software Development: Debugging complex code often requires a similar methodical approach, tracing execution flow, testing different paths, and isolating the source of errors.
-
Project Management: Allocating resources efficiently and managing project timelines require careful planning and strategic resource allocation, mirroring the precise water-pouring steps in the riddle.
-
Scientific Research: Experimental design and data analysis demand a methodical approach to data collection, analysis, and interpretation, often requiring iterative refinement and adjustment.
-
Financial Planning: Balancing budgets and managing investments necessitate a systematic and organized approach to ensure efficient resource utilization and goal achievement.
Developing Problem-Solving Skills: Tips and Strategies
Mastering the 3-gallon and 5-gallon bucket riddle, and puzzles like it, isn't about finding the answer immediately; it's about cultivating effective problem-solving skills. Here are some crucial strategies:
-
Visualize the Problem: Creating a mental picture or a physical representation (using actual containers and water, for instance) can greatly enhance understanding.
-
Break Down the Problem: Divide the overall goal into smaller, more manageable steps. This makes the process less daunting and allows for a more focused approach.
-
Embrace Trial and Error: Don't be discouraged by failed attempts. Each attempt provides valuable insights and eliminates potential dead ends. Learn from your mistakes and adjust your approach accordingly.
-
Document Your Progress: Keep track of your steps, noting both successful and unsuccessful attempts. This aids in identifying patterns and refining your strategy.
-
Seek Different Perspectives: Discussing the problem with others can provide fresh insights and alternative approaches that you might not have considered independently.
Conclusion: The Enduring Appeal of a Simple Puzzle
The seemingly simple 3-gallon and 5-gallon bucket riddle offers a powerful tool for honing critical thinking, problem-solving, and strategic planning skills. Its enduring appeal lies in its ability to challenge and engage individuals of all ages and backgrounds. By mastering this puzzle, you not only unlock the solution but also cultivate a more structured and effective approach to tackling complex challenges across various aspects of life. The riddle serves as a reminder that the most effective solutions often involve a methodical approach, a willingness to learn from mistakes, and a persistent pursuit of the optimal outcome. Its simplicity belies its depth, offering a valuable lesson in the power of logical reasoning and the importance of a systematic approach to problem-solving.
Latest Posts
Latest Posts
-
What Is The Electron Configuration For He
May 10, 2025
-
The Difference Between Static And Current Electricity
May 10, 2025
-
A Force Of 350 N Causes A Body To Move
May 10, 2025
-
What Is The Relationship Between Energy Use And Motion
May 10, 2025
-
Any Place Where Two Bones Meet
May 10, 2025
Related Post
Thank you for visiting our website which covers about 3 Gallon And 5 Gallon Bucket Riddle . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.