2y 18x 26 Solve For Y
listenit
May 11, 2025 · 4 min read
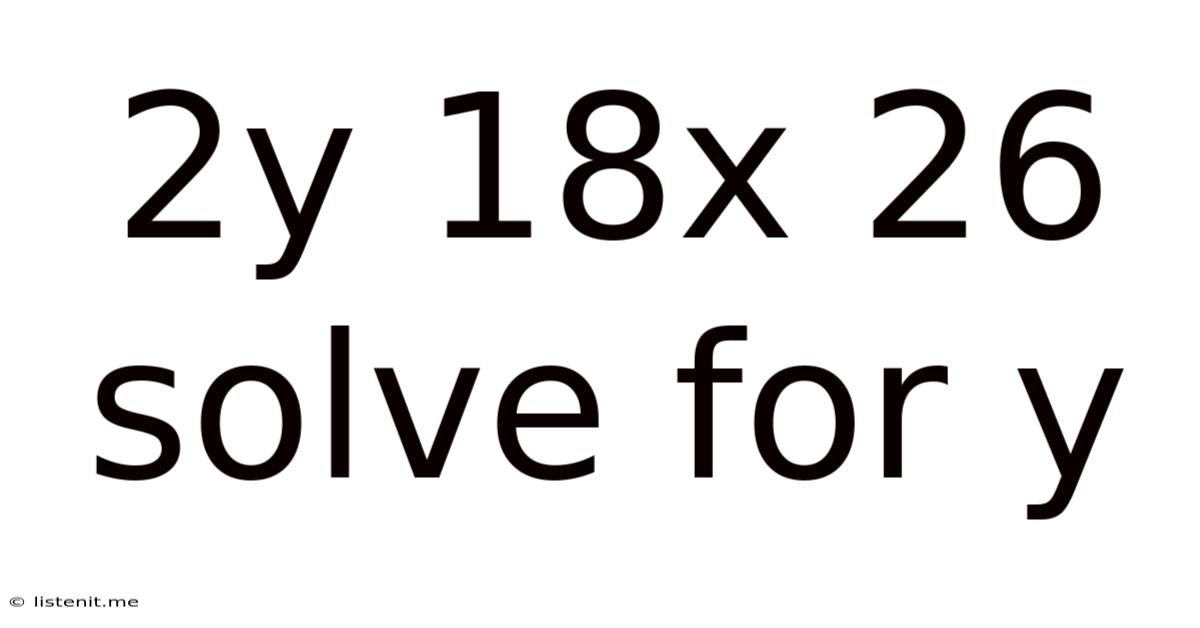
Table of Contents
Decoding the Mathematical Enigma: Solving for 'y' in 2y + 18x = 26
The equation 2y + 18x = 26 presents a seemingly simple algebraic challenge: solve for 'y'. While straightforward in its presentation, this equation offers a valuable opportunity to explore fundamental algebraic principles and their applications. This comprehensive guide will not only provide the solution but also delve into the underlying concepts, offering a deeper understanding of algebraic manipulation and its relevance in various fields.
Understanding the Equation: Variables and Constants
Before we embark on solving for 'y', let's dissect the equation's components. We have:
- 2y: This represents two times the value of 'y'. 'y' is a variable, meaning its value is unknown and can change.
- 18x: This represents eighteen times the value of 'x'. Similarly, 'x' is also a variable.
- 26: This is a constant, a fixed numerical value that doesn't change.
- += This represents the equality; the expression on the left side of the equation is equal to the expression on the right.
The equation 2y + 18x = 26 is a linear equation in two variables. This means that if we were to graph it, it would represent a straight line. The solution to this equation isn't a single numerical value for 'y', but rather an expression that defines 'y' in terms of 'x'.
Solving for 'y': A Step-by-Step Guide
Our goal is to isolate 'y' on one side of the equation. We achieve this by performing a series of algebraic manipulations, ensuring we maintain the equality of both sides. Here's the breakdown:
-
Subtract 18x from both sides: This removes the '18x' term from the left side, leaving only terms involving 'y'.
2y + 18x - 18x = 26 - 18x
This simplifies to:
2y = 26 - 18x
-
Divide both sides by 2: This isolates 'y' by eliminating the coefficient '2'.
(2y) / 2 = (26 - 18x) / 2
This simplifies to:
y = 13 - 9x
Therefore, the solution for 'y' is y = 13 - 9x. This expression shows that the value of 'y' depends on the value of 'x'. For every value of 'x' you choose, you'll get a corresponding value of 'y'.
Exploring Different Values of x and y
Let's examine how different values of 'x' influence the value of 'y':
- If x = 0: y = 13 - 9(0) = 13
- If x = 1: y = 13 - 9(1) = 4
- If x = 2: y = 13 - 9(2) = -5
- If x = -1: y = 13 - 9(-1) = 22
This demonstrates the relationship between 'x' and 'y'. Plotting these points on a graph would reveal the straight line representing the equation 2y + 18x = 26.
The Significance of Linear Equations
Linear equations, like the one we solved, are fundamental in mathematics and have numerous applications across various disciplines:
- Physics: Describing motion, calculating forces, and analyzing relationships between physical quantities.
- Engineering: Modeling systems, designing structures, and optimizing processes.
- Economics: Analyzing supply and demand, forecasting economic trends, and modeling market behavior.
- Computer Science: Developing algorithms, creating simulations, and designing computer graphics.
- Statistics: Analyzing data, creating regression models, and making predictions.
Advanced Concepts and Extensions
While we've solved the equation for 'y', let's explore some related mathematical concepts:
- Simultaneous Equations: If we had another equation involving 'x' and 'y', we could solve for both variables simultaneously. This often involves methods like substitution or elimination.
- Linear Inequalities: Instead of an equals sign, we could have an inequality (>, <, ≥, ≤). This would change the solution from a line to a region on a graph.
- Systems of Linear Equations: Dealing with multiple linear equations with multiple variables, often solved using matrices and linear algebra techniques.
- Non-linear Equations: Equations where the variables are raised to powers other than 1 (e.g., quadratic equations, cubic equations). Solving these often requires different techniques.
Practical Applications and Real-World Examples
The ability to solve linear equations like 2y + 18x = 26 has numerous practical applications:
- Calculating Costs: Imagine a scenario where 'x' represents the number of hours worked and 'y' represents the total earnings. The equation could model the relationship between hours worked and total pay, considering a base pay and an hourly rate.
- Mixing Solutions: In chemistry, linear equations can be used to calculate the amounts of different solutions needed to achieve a desired concentration.
- Distance-Rate-Time Problems: Linear equations are frequently used in solving problems involving distance, rate, and time.
Conclusion: Mastering Algebraic Manipulation
Solving the equation 2y + 18x = 26 for 'y' isn't just about finding a numerical answer; it's about understanding the fundamental principles of algebra. The process of manipulating the equation to isolate 'y' showcases core algebraic techniques applicable to a wide range of problems. Mastering these techniques provides a solid foundation for tackling more complex mathematical challenges and applying algebraic concepts to real-world scenarios. The solution, y = 13 - 9x, is not just an answer; it's a representation of a relationship between two variables, a relationship that can be visualized, analyzed, and applied across diverse fields. By understanding this seemingly simple equation, we unlock a gateway to a deeper understanding of the power and versatility of algebra.
Latest Posts
Latest Posts
-
Chloroplast And Mitochondria Are Similar In That They Both
May 11, 2025
-
What Is The Name Of The Compound Co
May 11, 2025
-
What Is The Domain Of Y Cos X
May 11, 2025
-
Find All Zeros Of The Function
May 11, 2025
-
Write Sin In Terms Of Cos
May 11, 2025
Related Post
Thank you for visiting our website which covers about 2y 18x 26 Solve For Y . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.