25 Percent As A Fraction In Simplest Form
listenit
May 10, 2025 · 5 min read
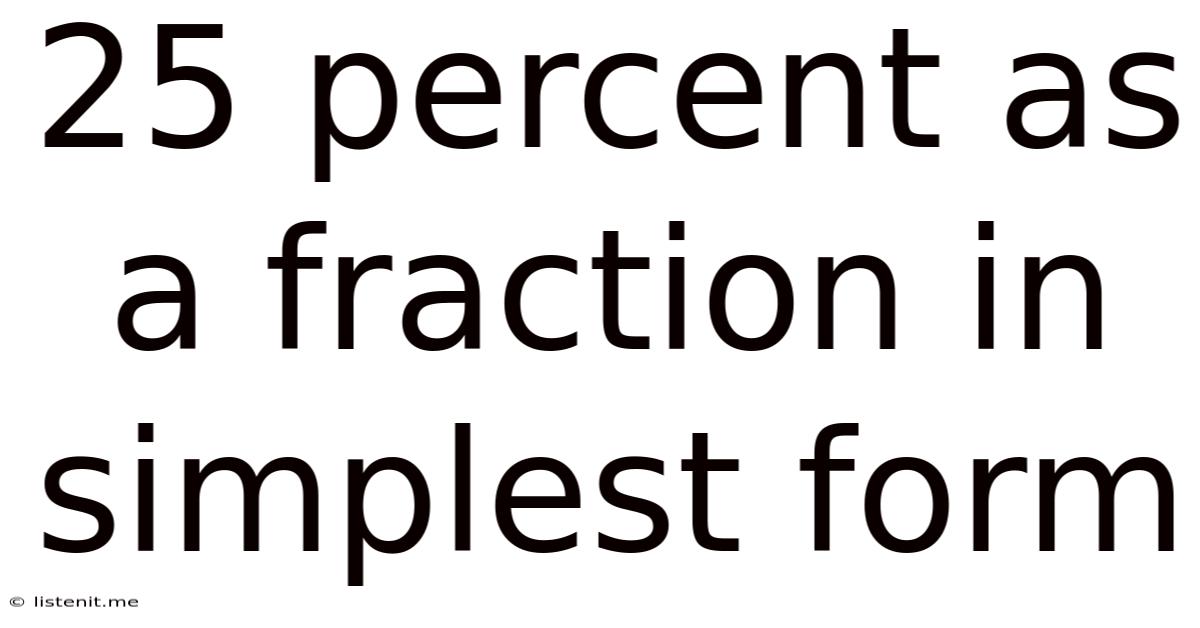
Table of Contents
25 Percent as a Fraction in Simplest Form: A Comprehensive Guide
Understanding percentages, fractions, and decimals is fundamental to various aspects of life, from everyday calculations to complex financial analyses. This comprehensive guide dives deep into expressing 25 percent as a fraction in its simplest form, exploring the underlying concepts and providing practical examples. We'll also delve into related percentage conversions and demonstrate how to simplify fractions effectively.
Understanding Percentages and Fractions
Before we tackle the conversion of 25 percent to a fraction, let's solidify our understanding of these two core mathematical concepts.
What is a Percentage?
A percentage is a way of expressing a number as a fraction of 100. The word "percent" literally means "out of one hundred" ("per cent" from Latin). Therefore, 25 percent means 25 out of 100. This representation is incredibly useful for comparing proportions and making relative comparisons between different quantities.
What is a Fraction?
A fraction represents a part of a whole. It's expressed as a ratio of two numbers, the numerator (top number) and the denominator (bottom number). The denominator indicates the total number of equal parts, while the numerator indicates how many of those parts are being considered. For example, ½ represents one out of two equal parts.
Converting 25 Percent to a Fraction
The conversion of 25 percent to a fraction is a straightforward process. Since percentage means "out of 100", 25 percent can be written directly as a fraction:
25/100
This fraction represents 25 parts out of a total of 100 parts. However, this fraction isn't in its simplest form. To simplify a fraction, we need to find the greatest common divisor (GCD) of both the numerator and the denominator and divide both by it.
Finding the Greatest Common Divisor (GCD)
The greatest common divisor (GCD) of two numbers is the largest number that divides both numbers without leaving a remainder. For 25 and 100, the GCD is 25. This means that both 25 and 100 are divisible by 25.
Simplifying the Fraction
Now that we've identified the GCD as 25, we can simplify the fraction:
25 ÷ 25 / 100 ÷ 25 = 1/4
Therefore, 25 percent expressed as a fraction in its simplest form is 1/4.
Practical Applications and Real-World Examples
Understanding the equivalence of 25% and 1/4 has numerous practical applications in everyday life:
-
Sales and Discounts: If a store offers a 25% discount, it's equivalent to a ¼ reduction in price. If an item costs $100, the discount would be $25 ($100 x ¼ = $25).
-
Recipe Adjustments: When halving or quartering a recipe, understanding fractions is crucial. If a recipe calls for 1 cup of flour and you want to make ¼ of the recipe, you would use ¼ cup of flour. This is directly related to 25%.
-
Data Analysis: In data analysis, percentages are often converted to fractions for easier calculations and comparisons. For instance, if 25% of survey respondents answered "yes," this represents ¼ of the total respondents.
-
Financial Calculations: Interest rates, loan repayments, and investment returns are often expressed as percentages. Understanding the fractional equivalent facilitates calculations and comparisons.
Expanding on Fraction Simplification Techniques
While the simplification of 25/100 to 1/4 was relatively straightforward, let's explore more general techniques for simplifying fractions:
Method 1: Finding the Greatest Common Divisor (GCD)
This method, as demonstrated earlier, involves finding the largest number that divides both the numerator and the denominator evenly. There are several ways to find the GCD:
-
Listing Factors: List all the factors of both the numerator and the denominator. The largest factor common to both is the GCD.
-
Prime Factorization: Break down both the numerator and the denominator into their prime factors. The GCD is the product of the common prime factors raised to the lowest power.
-
Euclidean Algorithm: This algorithm efficiently finds the GCD of two numbers through a series of divisions.
Method 2: Dividing by Common Factors
This involves repeatedly dividing the numerator and the denominator by common factors until no common factors remain. This method is often simpler for smaller numbers. For example, if we had the fraction 12/18, we could divide both by 2 to get 6/9, and then divide both by 3 to get 2/3.
Method 3: Using a Fraction Calculator
Many online calculators and software programs can simplify fractions automatically. Simply input the numerator and denominator, and the calculator will provide the simplified fraction. This is particularly useful for larger and more complex fractions.
Converting Other Percentages to Fractions
The process of converting percentages to fractions follows the same principle:
-
Write the percentage as a fraction with a denominator of 100. For example, 75% becomes 75/100.
-
Simplify the fraction by finding the GCD of the numerator and denominator and dividing both by it. 75/100 simplifies to ¾ (GCD = 25).
-
Express the fraction in its simplest form.
Here are a few more examples:
- 50% = 50/100 = 1/2
- 75% = 75/100 = 3/4
- 10% = 10/100 = 1/10
- 15% = 15/100 = 3/20
Common Percentage to Fraction Conversions
Remembering common percentage-to-fraction conversions can be very helpful:
- 25% = 1/4
- 50% = 1/2
- 75% = 3/4
- 10% = 1/10
- 20% = 1/5
- 33.33% ≈ 1/3 (approximately, as 1/3 is a repeating decimal)
- 66.66% ≈ 2/3 (approximately, as 2/3 is a repeating decimal)
Knowing these conversions can speed up calculations and improve understanding in various contexts.
Conclusion
Expressing 25 percent as a fraction in its simplest form (1/4) is a fundamental skill with numerous practical applications. Understanding the underlying principles of percentages and fractions, along with mastering fraction simplification techniques, empowers individuals to handle a wide array of mathematical problems efficiently and confidently. Whether you're dealing with discounts, recipes, data analysis, or financial calculations, a solid grasp of these concepts is invaluable.
Latest Posts
Latest Posts
-
Mitosis Occurs In Which Layer Of The Skin
May 10, 2025
-
Which Of The Following Problems Would Not Have A Solution
May 10, 2025
-
How Many Valence Electrons Does Group 13 Have
May 10, 2025
-
How Many Oxygen Atoms Are In Water
May 10, 2025
-
4 Is Subtracted From The Cube Of A Number
May 10, 2025
Related Post
Thank you for visiting our website which covers about 25 Percent As A Fraction In Simplest Form . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.