25.7 Is What Percent Of 141
listenit
May 24, 2025 · 4 min read
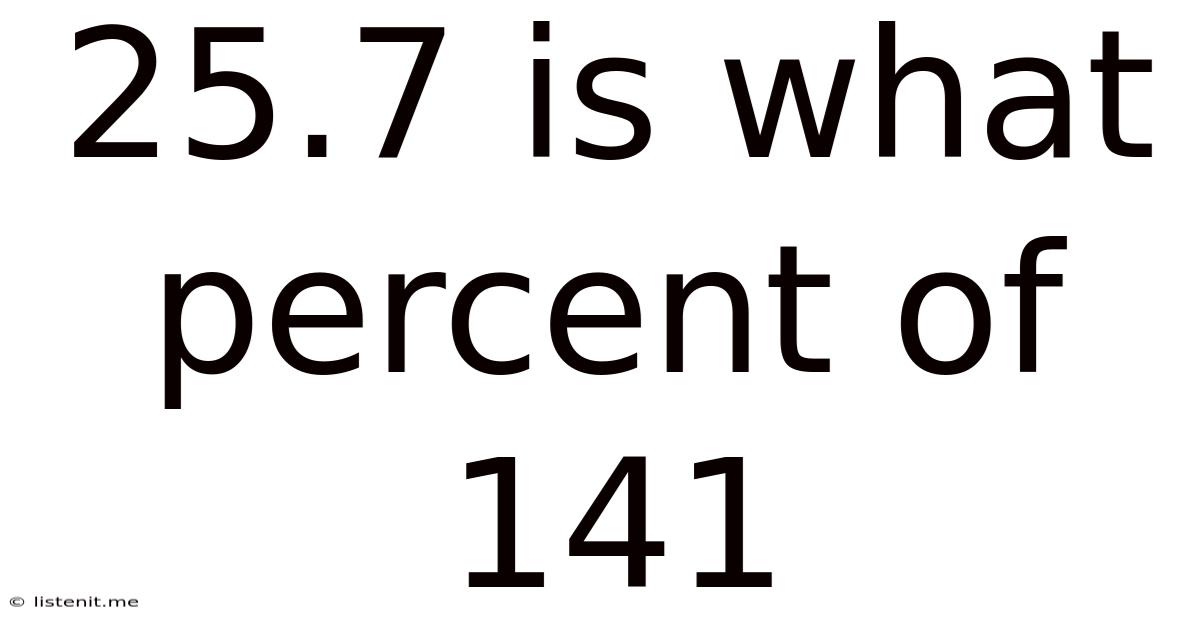
Table of Contents
25.7 is What Percent of 141? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across various fields, from everyday budgeting to complex financial analysis. This article delves deep into calculating percentages, using the example "25.7 is what percent of 141?" as a springboard to explore different methods, applications, and related concepts. We'll cover the basic formula, alternative approaches, practical examples, and troubleshooting common mistakes. By the end, you'll not only know the answer to our initial question but also possess a solid understanding of percentage calculations.
Understanding the Fundamentals: The Percentage Formula
The core of any percentage problem lies in understanding the basic formula:
Part / Whole = Percentage / 100
Where:
- Part: Represents the portion you're interested in (in our case, 25.7).
- Whole: Represents the total amount (in our case, 141).
- Percentage: The unknown value we need to find.
This formula can be rearranged to solve for any of the three variables. To find the percentage, we rearrange it as follows:
Percentage = (Part / Whole) * 100
Calculating "25.7 is What Percent of 141?"
Let's apply the formula to our specific problem:
Percentage = (25.7 / 141) * 100
Using a calculator:
Percentage ≈ 18.23%
Therefore, 25.7 is approximately 18.23% of 141.
Alternative Methods and Approaches
While the above method is straightforward, let's explore alternative approaches to demonstrate the versatility of percentage calculations:
Method 2: Using Proportions
We can also solve this using proportions. We set up a proportion where x represents the percentage:
25.7 / 141 = x / 100
To solve for x, we cross-multiply:
141x = 2570
x = 2570 / 141
x ≈ 18.23%
This method confirms our previous result.
Method 3: Using Decimal Conversion
Another approach involves converting the fraction (Part/Whole) into a decimal and then multiplying by 100:
25.7 / 141 ≈ 0.1823
0.1823 * 100 ≈ 18.23%
This highlights the relationship between decimals and percentages; simply multiplying a decimal by 100 converts it to a percentage.
Practical Applications of Percentage Calculations
Percentage calculations are incredibly useful in various real-world scenarios:
-
Finance: Calculating interest rates, discounts, profit margins, tax rates, and investment returns all rely on percentage calculations. For instance, understanding the percentage change in your investment portfolio is crucial for financial planning.
-
Retail: Determining discounts, sales tax, and markups are fundamental aspects of retail operations. Calculating the percentage discount on a sale item helps customers understand the savings they are getting.
-
Science and Statistics: Percentages are essential in data analysis, representing proportions, probabilities, and error rates. Scientific studies often report results as percentages to make findings easier to interpret.
-
Education: Calculating grades, test scores, and performance metrics often involve percentages. A student's overall grade is typically represented as a percentage of the total possible points.
-
Everyday Life: Understanding tips, tax increases, and price changes often requires calculating percentages. Knowing the percentage of ingredients in a recipe ensures accurate measurements.
Understanding Percentage Change
Beyond calculating a percentage of a whole, we often need to determine the percentage change between two values. The formula for percentage change is:
Percentage Change = [(New Value - Old Value) / Old Value] * 100
A positive percentage change indicates an increase, while a negative percentage change indicates a decrease.
Addressing Common Mistakes in Percentage Calculations
Several common mistakes can lead to inaccurate results:
-
Incorrect Order of Operations: Always remember the order of operations (PEMDAS/BODMAS): Parentheses/Brackets, Exponents/Orders, Multiplication and Division (from left to right), Addition and Subtraction (from left to right).
-
Misinterpreting the "Whole": Carefully identify the correct "whole" or total value in the problem. This is crucial for accurate calculations.
-
Rounding Errors: Rounding intermediate results can lead to significant errors in the final answer. It’s best to carry out calculations to several decimal places before rounding the final answer.
-
Confusing Percentage Increase/Decrease with the Final Value: Don't confuse the percentage change with the final value after the percentage change has been applied.
Advanced Percentage Problems and Concepts
While our initial problem was relatively simple, percentage calculations can become more complex. Here are some advanced concepts:
-
Compound Interest: This involves calculating interest not only on the principal amount but also on accumulated interest.
-
Percentage Points vs. Percentage Change: A difference of "percentage points" is different from a percentage change. For example, a change from 10% to 15% is a 5 percentage point increase but a 50% percentage increase.
-
Percentage of a Percentage: This requires calculating a percentage of a percentage, often involving nested calculations.
Conclusion: Mastering Percentage Calculations
Understanding percentages is a valuable skill with wide-ranging applications. This article provides a comprehensive guide to calculating percentages, including various methods, practical examples, and common pitfalls. By mastering these techniques, you'll be better equipped to handle percentage calculations confidently in your academic, professional, and personal life. Remember to practice regularly and refer back to these methods as needed. With consistent practice, you'll find percentage calculations become second nature. The initial problem, "25.7 is what percent of 141?", serves as a simple yet effective starting point for this valuable journey into the world of percentages. Now you not only know the answer, but you also possess the tools to tackle a wide range of percentage-related problems with confidence.
Latest Posts
Latest Posts
-
How Old Am I If I M Born In 1967
May 24, 2025
-
8 5 X 11 Divided By 2
May 24, 2025
-
If Someone Was Born In 1989 How Old Are They
May 24, 2025
-
What Is 6 5 As A Fraction
May 24, 2025
-
Greatest Common Factor Of 10 And 35
May 24, 2025
Related Post
Thank you for visiting our website which covers about 25.7 Is What Percent Of 141 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.