2 Out Of 30 As A Percentage
listenit
May 24, 2025 · 5 min read
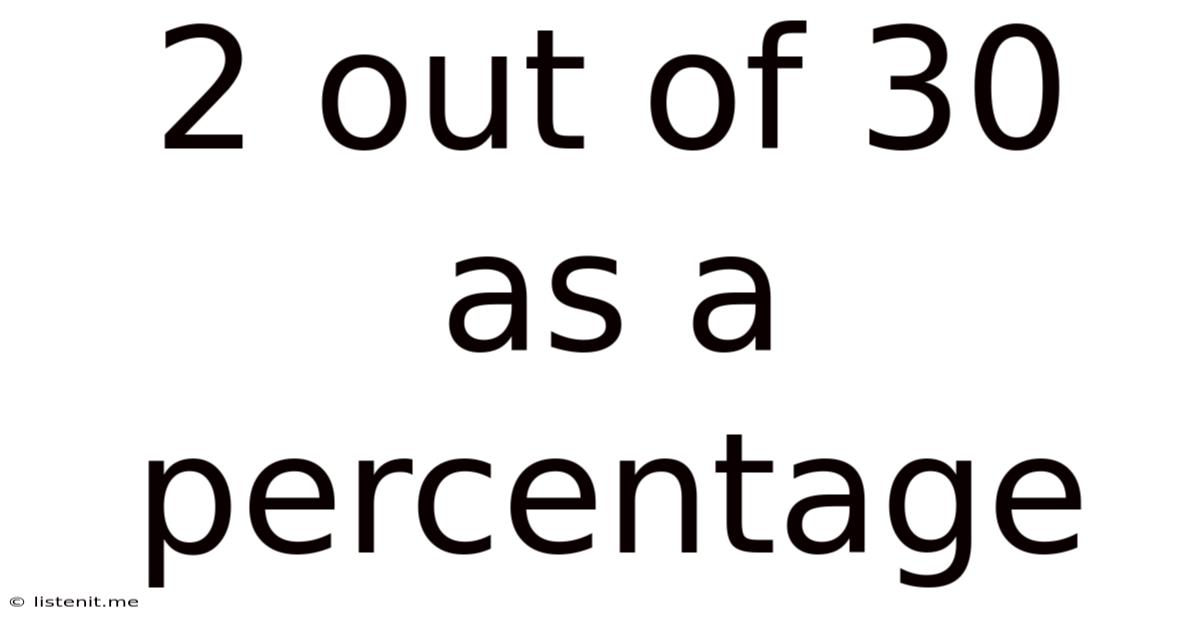
Table of Contents
Decoding the Fraction: 2 out of 30 as a Percentage and its Practical Applications
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts in a store to comprehending complex financial data. This article delves deep into the seemingly simple question: What is 2 out of 30 as a percentage? We'll not only provide the answer but also explore the underlying mathematical concepts, practical applications, and different methods for solving similar percentage problems. We'll also touch upon the importance of percentage calculations in various fields and offer tips for improving your understanding and skills.
Understanding the Basics: Fractions, Decimals, and Percentages
Before we tackle the specific problem of converting "2 out of 30" into a percentage, let's solidify our understanding of the fundamental relationships between fractions, decimals, and percentages.
-
Fractions: A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). In our case, "2 out of 30" is represented as the fraction 2/30.
-
Decimals: Decimals are another way to represent parts of a whole. They use a base-ten system, with the digits to the right of the decimal point representing tenths, hundredths, thousandths, and so on.
-
Percentages: A percentage represents a fraction or decimal as a proportion of 100. The symbol "%" signifies "per hundred." For example, 50% means 50 out of 100, which is equivalent to the fraction 50/100 or the decimal 0.5.
Calculating 2 out of 30 as a Percentage: Step-by-Step Guide
There are several ways to calculate 2 out of 30 as a percentage. Here are two common methods:
Method 1: Using the Fraction-to-Percentage Conversion
-
Express as a fraction: Write "2 out of 30" as the fraction 2/30.
-
Simplify the fraction: Reduce the fraction to its simplest form by dividing both the numerator and the denominator by their greatest common divisor (GCD), which is 2 in this case. This simplifies to 1/15.
-
Convert to a decimal: Divide the numerator (1) by the denominator (15). This gives us 0.066666... (a repeating decimal).
-
Convert to a percentage: Multiply the decimal by 100. 0.066666... x 100 ≈ 6.67%.
Therefore, 2 out of 30 is approximately 6.67%.
Method 2: Using the Direct Percentage Calculation
-
Set up the proportion: We can set up a proportion: (2/30) = (x/100), where 'x' represents the percentage we want to find.
-
Cross-multiply: Multiply 2 by 100 and 30 by x: 200 = 30x
-
Solve for x: Divide both sides by 30: x = 200/30 = 6.6666...
-
Convert to a percentage: This gives us approximately 6.67%.
Again, we find that 2 out of 30 is approximately 6.67%. The slight difference in the last decimal place is due to rounding.
Practical Applications of Percentage Calculations
Understanding percentage calculations is crucial in numerous real-world scenarios. Here are a few examples:
-
Finance: Calculating interest rates, returns on investments, discounts, taxes, and loan repayments all involve percentages. Understanding these calculations helps in making informed financial decisions.
-
Statistics: Percentages are frequently used to represent data and trends in statistical analysis. For instance, survey results are often expressed as percentages to show the proportion of respondents who chose a particular answer.
-
Retail: Businesses use percentages to calculate discounts, markups, and sales tax. Consumers need to understand percentages to compare prices and find the best deals.
-
Science: Percentages are used extensively in scientific studies to express proportions, concentrations, and changes in various quantities.
-
Education: Grade calculations, test scores, and performance evaluations often rely on percentage calculations.
Beyond the Basics: Handling More Complex Percentage Problems
While the example of "2 out of 30" is relatively straightforward, more complex percentage problems may arise. Let's explore some scenarios and techniques to tackle them:
1. Finding the Percentage Increase or Decrease: This involves calculating the percentage change between two values. For example, if a price increases from $10 to $12, the percentage increase is calculated as follows:
- Change in value: $12 - $10 = $2
- Percentage increase: ($2/$10) x 100 = 20%
2. Finding the Original Value: Sometimes, you know the percentage and the resulting value, and you need to find the original value. For example, if a discounted item costs $80 after a 20% discount, the original price can be calculated using the following:
- Let 'x' be the original price.
- 80 = x - 0.20x
- 80 = 0.80x
- x = 80/0.80 = $100
3. Dealing with Multiple Percentages: When dealing with multiple percentage changes (e.g., successive discounts), it's crucial to apply each percentage sequentially. Simply adding the percentages together will lead to an incorrect result.
Improving Your Percentage Calculation Skills
Mastering percentage calculations requires consistent practice and a solid understanding of the underlying concepts. Here are some tips to improve your skills:
-
Practice regularly: Solve a variety of percentage problems to build your confidence and fluency.
-
Use different methods: Try both the fraction-to-percentage and direct calculation methods to understand the different approaches.
-
Visual aids: Diagrams and charts can help visualize percentage relationships and make the calculations more intuitive.
-
Online resources: Numerous online calculators, tutorials, and practice exercises are available to help you learn and practice.
-
Real-world applications: Apply your knowledge to real-life situations to reinforce your understanding and make the learning more engaging.
Conclusion: The Power of Percentages
The seemingly simple problem of converting "2 out of 30" into a percentage (approximately 6.67%) highlights the importance of understanding percentage calculations. This fundamental mathematical concept has widespread applications in various fields, empowering us to analyze data, make informed decisions, and solve practical problems in our daily lives. By mastering the techniques discussed in this article and practicing regularly, you can build a strong foundation in percentage calculations and confidently tackle more complex problems. Remember, the key is consistent practice and applying your knowledge to real-world situations to enhance your understanding and build your skills.
Latest Posts
Latest Posts
-
2895 Divided By 229 With Remainder
May 24, 2025
-
How Many Square Feet Does 24000 Btu Cool
May 24, 2025
-
How To Calculate Concrete In A Cylinder
May 24, 2025
-
What Is The Greatest Common Factor Of 26 And 34
May 24, 2025
-
Army Height And Weight Tape Standards 2023
May 24, 2025
Related Post
Thank you for visiting our website which covers about 2 Out Of 30 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.