19 Out Of 50 As A Percentage
listenit
May 24, 2025 · 4 min read
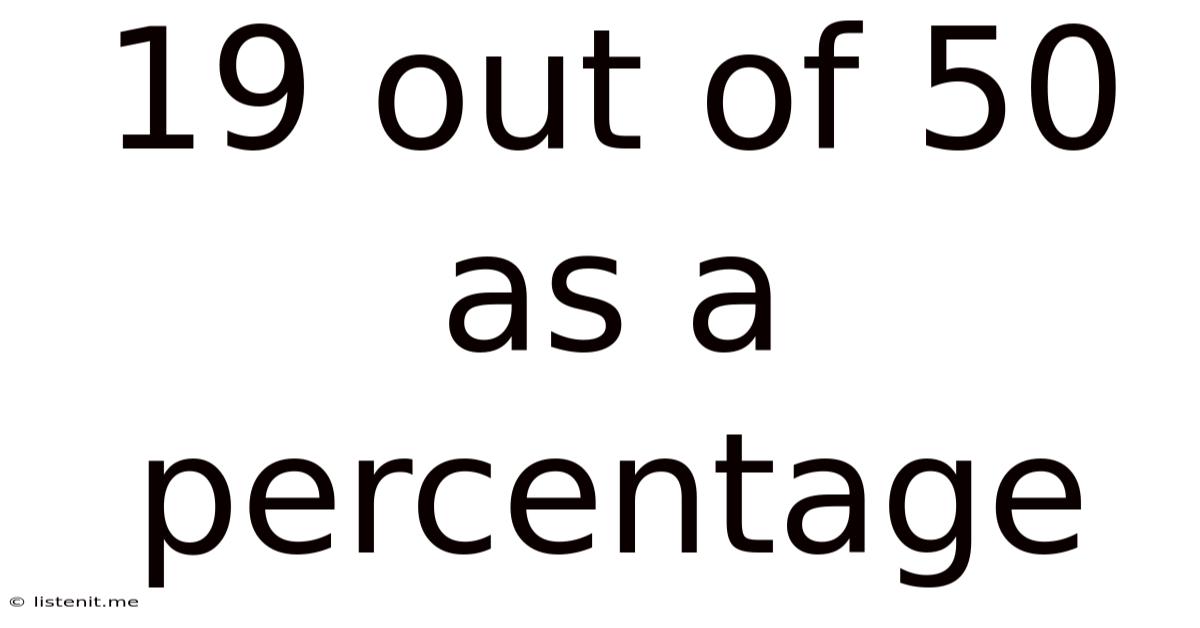
Table of Contents
19 out of 50 as a Percentage: A Comprehensive Guide
Converting fractions to percentages is a fundamental skill in mathematics with widespread applications in everyday life, from calculating discounts in shops to understanding statistics in news reports. This comprehensive guide delves into the calculation of "19 out of 50 as a percentage," exploring various methods and offering practical examples to solidify your understanding. We'll also touch upon the broader implications of percentage calculations and how they relate to other mathematical concepts.
Understanding Fractions and Percentages
Before we dive into the calculation, let's refresh our understanding of fractions and percentages.
Fractions: A fraction represents a part of a whole. It's expressed as a ratio of two numbers: the numerator (top number) and the denominator (bottom number). In our case, "19 out of 50" is represented as the fraction 19/50. The numerator (19) indicates the part we're interested in, while the denominator (50) represents the whole.
Percentages: A percentage is a way of expressing a fraction as a portion of 100. The symbol "%" represents "per cent," meaning "out of 100." Therefore, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Calculating 19 out of 50 as a Percentage: Method 1 - Using Proportions
This method uses the concept of proportions to find the equivalent percentage. We set up a proportion where x represents the percentage we want to find:
19/50 = x/100
To solve for x, we cross-multiply:
50x = 19 * 100
50x = 1900
x = 1900 / 50
x = 38
Therefore, 19 out of 50 is 38%.
Calculating 19 out of 50 as a Percentage: Method 2 - Decimal Conversion
This approach involves first converting the fraction to a decimal and then multiplying by 100 to obtain the percentage.
-
Convert the fraction to a decimal: Divide the numerator (19) by the denominator (50):
19 ÷ 50 = 0.38
-
Multiply the decimal by 100: This converts the decimal to a percentage:
0.38 * 100 = 38
Therefore, 19 out of 50 is 38%.
Calculating 19 out of 50 as a Percentage: Method 3 - Using a Calculator
Most calculators have a percentage function that simplifies the process significantly. Simply enter 19 ÷ 50 and then multiply the result by 100. The calculator will automatically display the answer as 38%. This is the most straightforward method for quick calculations.
Practical Applications of Percentage Calculations
Understanding how to calculate percentages is crucial in numerous real-world scenarios:
-
Sales and Discounts: Calculating discounts offered in stores. For example, a 38% discount on a $100 item would mean a saving of $38.
-
Grade Calculation: Determining your final grade in a course based on individual assignment scores.
-
Financial Analysis: Analyzing financial statements, calculating interest rates, understanding investment returns, and assessing profit margins.
-
Data Analysis and Statistics: Interpreting statistical data presented as percentages, such as survey results, election polls, or economic indicators.
-
Scientific Research: Representing data and results in scientific experiments and studies.
-
Everyday Life: Determining tips in restaurants, calculating sales tax, and understanding various proportions in daily life.
Beyond the Basics: Understanding Percentage Increase and Decrease
While we've focused on calculating a percentage from a fraction, it's also important to understand percentage increases and decreases.
Percentage Increase: This calculates the percentage change when a value increases. The formula is:
[(New Value - Original Value) / Original Value] * 100
Percentage Decrease: This calculates the percentage change when a value decreases. The formula is:
[(Original Value - New Value) / Original Value] * 100
For example, if a stock price increases from $50 to $69, the percentage increase is:
[(69 - 50) / 50] * 100 = 38%
Conversely, if the price decreases from $69 to $50, the percentage decrease is:
[(69 - 50) / 69] * 100 ≈ 27.54%
Common Mistakes to Avoid When Calculating Percentages
-
Incorrect Order of Operations: Always follow the order of operations (PEMDAS/BODMAS) to ensure accurate calculations.
-
Incorrect Decimal Placement: Be mindful of decimal points when converting fractions to decimals and decimals to percentages.
-
Confusing Percentage Increase and Decrease: Understand the difference between the two and use the correct formula.
-
Rounding Errors: Be aware of potential rounding errors, especially when dealing with multiple calculations.
Advanced Applications and Related Concepts
The ability to calculate percentages is foundational to understanding more advanced mathematical concepts such as:
-
Ratios and Proportions: Percentages are directly related to ratios and proportions.
-
Probability and Statistics: Percentages are extensively used in probability calculations and statistical analysis.
-
Algebra: Percentage problems can often be solved using algebraic equations.
-
Finance and Economics: Percentages are essential tools in various financial and economic models.
Conclusion
Converting 19 out of 50 to a percentage, resulting in 38%, is a straightforward process achievable through multiple methods. This seemingly simple calculation has broad implications across numerous disciplines and everyday scenarios. Mastering this skill provides a strong foundation for understanding more complex mathematical concepts and effectively navigating the numerical world around us. By avoiding common mistakes and understanding the underlying principles, you can confidently apply percentage calculations to various situations, enhancing your analytical skills and decision-making abilities. Remember to practice regularly to solidify your understanding and improve your speed and accuracy.
Latest Posts
Latest Posts
-
How Many Days Has It Been Since March 1st
May 24, 2025
-
What Is 10 Percent Of 10 Thousand Dollars
May 24, 2025
-
What Fraction Of 1 2 Is 1 3
May 24, 2025
-
What Percentage Is 4 Out Of 24
May 24, 2025
-
Fit By Katy Macro Calculator Free
May 24, 2025
Related Post
Thank you for visiting our website which covers about 19 Out Of 50 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.