19 Of 30 Is What Percent
listenit
May 24, 2025 · 4 min read
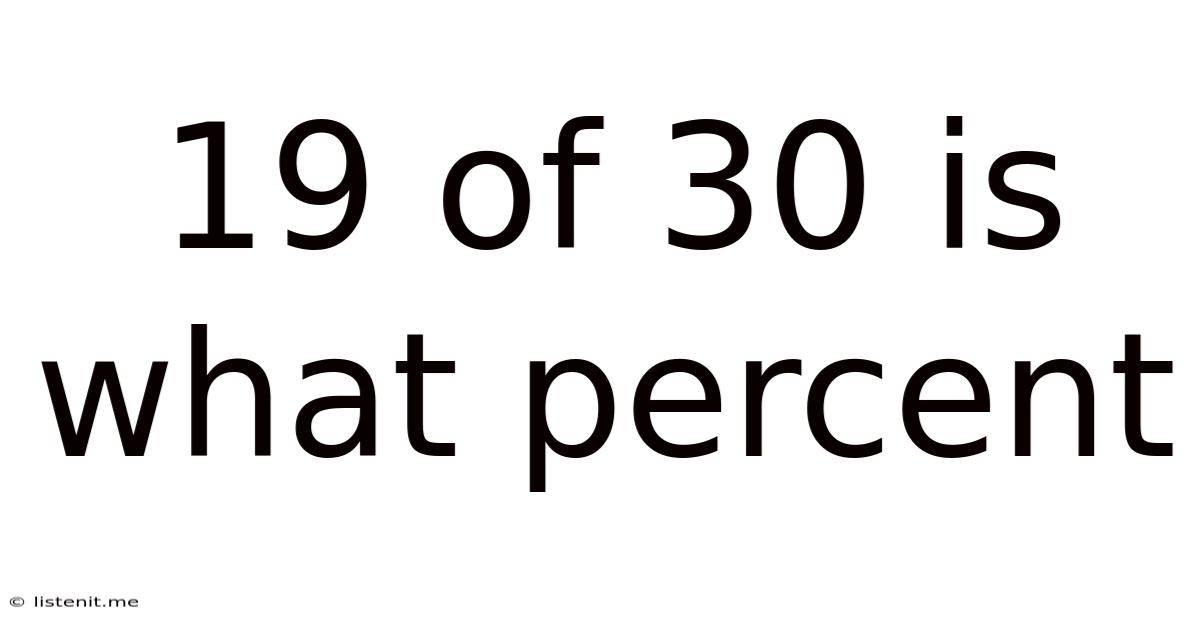
Table of Contents
19 out of 30 is What Percent? A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill applicable across various aspects of life, from understanding sales discounts to analyzing statistical data. This comprehensive guide delves into the calculation of "19 out of 30 is what percent?", exploring the methods, applications, and broader implications of percentage calculations. We'll cover the basic formula, different calculation methods, real-world examples, and even touch on advanced concepts related to percentages.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of one hundred." Therefore, 19 out of 30 represents a portion of the whole (30), and we want to express that portion as a percentage.
Key Terms:
- Numerator: The top number in a fraction (in this case, 19). This represents the part.
- Denominator: The bottom number in a fraction (in this case, 30). This represents the whole.
- Percentage: The number expressed as a fraction of 100.
Method 1: The Classic Formula
The most straightforward way to calculate the percentage is using the basic formula:
(Part / Whole) * 100 = Percentage
Substituting our values:
(19 / 30) * 100 = Percentage
- Divide the part by the whole: 19 divided by 30 equals approximately 0.6333.
- Multiply by 100: 0.6333 multiplied by 100 equals 63.33.
Therefore, 19 out of 30 is approximately 63.33%.
Method 2: Using Proportions
This method involves setting up a proportion:
19/30 = x/100
To solve for 'x' (the percentage):
Cross-multiply: 19 * 100 = 30 * x
1900 = 30x
Divide both sides by 30:
x = 1900 / 30 = 63.33
Again, we arrive at the answer: 63.33%.
Method 3: Utilizing a Calculator
Modern calculators simplify percentage calculations significantly. Simply divide 19 by 30, and then multiply the result by 100. Most calculators have a percentage function (%) that will automate this process.
Real-World Applications: Where Percentages Matter
Understanding percentage calculations is crucial in many everyday situations:
1. Finance and Budgeting:
- Interest Rates: Banks and financial institutions use percentages to calculate interest on loans and savings accounts.
- Sales Tax: Governments levy sales tax as a percentage of the purchase price.
- Discounts and Sales: Retailers advertise discounts (e.g., 20% off) using percentages. Calculating the actual savings requires understanding percentage calculations.
- Investment Returns: Investors track their portfolio performance using percentage changes in asset values.
2. Academic Performance:
- Grades and Scores: Grades are often expressed as percentages, reflecting the proportion of correctly answered questions or the overall performance in a course.
- Test Scores: Standardized tests frequently report scores as percentages.
3. Data Analysis and Statistics:
- Market Share: Businesses analyze market share using percentages to determine their position relative to competitors.
- Survey Results: Survey results are often expressed as percentages to represent the proportion of respondents who chose a particular option.
- Statistical Significance: In statistical analysis, percentages play a crucial role in determining the significance of findings.
4. Everyday Life:
- Tip Calculations: Calculating tips in restaurants usually involves calculating a percentage of the total bill.
- Recipe Scaling: Adjusting recipe quantities involves understanding proportions and percentages.
Beyond the Basics: Working with Percentages
While calculating "19 out of 30" is a straightforward example, many percentage problems require more complex calculations:
1. Finding the Percentage Increase or Decrease:
Suppose a product's price increased from $20 to $25. To calculate the percentage increase:
- Find the difference: $25 - $20 = $5
- Divide the difference by the original value: $5 / $20 = 0.25
- Multiply by 100: 0.25 * 100 = 25%
The price increased by 25%.
Conversely, if the price decreased from $25 to $20, the percentage decrease would also be 25%.
2. Finding the Original Value:
If a product is discounted by 20% and now costs $80, we can find the original price:
- Represent the discount as a decimal: 20% = 0.20
- Subtract the discount from 1: 1 - 0.20 = 0.80 (This represents 80% of the original price)
- Divide the current price by the remaining percentage: $80 / 0.80 = $100
The original price was $100.
3. Compounding Percentages:
Compounding percentages involves calculating percentage changes on a value that has already undergone a percentage change. This is common in finance (compound interest) and population growth. These calculations require careful attention to the order of operations.
Practical Tips for Accurate Percentage Calculations:
- Double-check your work: Errors in simple arithmetic can significantly impact the results.
- Use a calculator: Calculators provide speed and accuracy, especially for more complex calculations.
- Understand the context: Always consider the context of the problem. What does the percentage represent in the real world?
- Round appropriately: Round your final answer to a reasonable number of decimal places, based on the context of the problem.
Conclusion: Mastering Percentages for Success
Understanding percentages is a vital skill with far-reaching applications. Whether you're managing personal finances, analyzing data, or navigating everyday situations, mastering percentage calculations empowers you to make informed decisions and interpret information effectively. From the simple calculation of "19 out of 30 is what percent?" to more advanced applications, a strong grasp of percentage concepts is an invaluable asset in both personal and professional life. Remember to practice regularly and apply these methods to various real-world scenarios to solidify your understanding.
Latest Posts
Latest Posts
-
What Is The Greatest Common Factor Of 85 And 51
May 25, 2025
-
What Is 8 Weeks Ago From Today
May 25, 2025
-
How To Do The Love Calculator On Paper
May 25, 2025
-
What Is The Greatest Common Factor Of 4 And 16
May 25, 2025
-
Write The Prime Factorization Of 6
May 25, 2025
Related Post
Thank you for visiting our website which covers about 19 Of 30 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.