18 Out Of 35 As A Percentage
listenit
May 25, 2025 · 5 min read
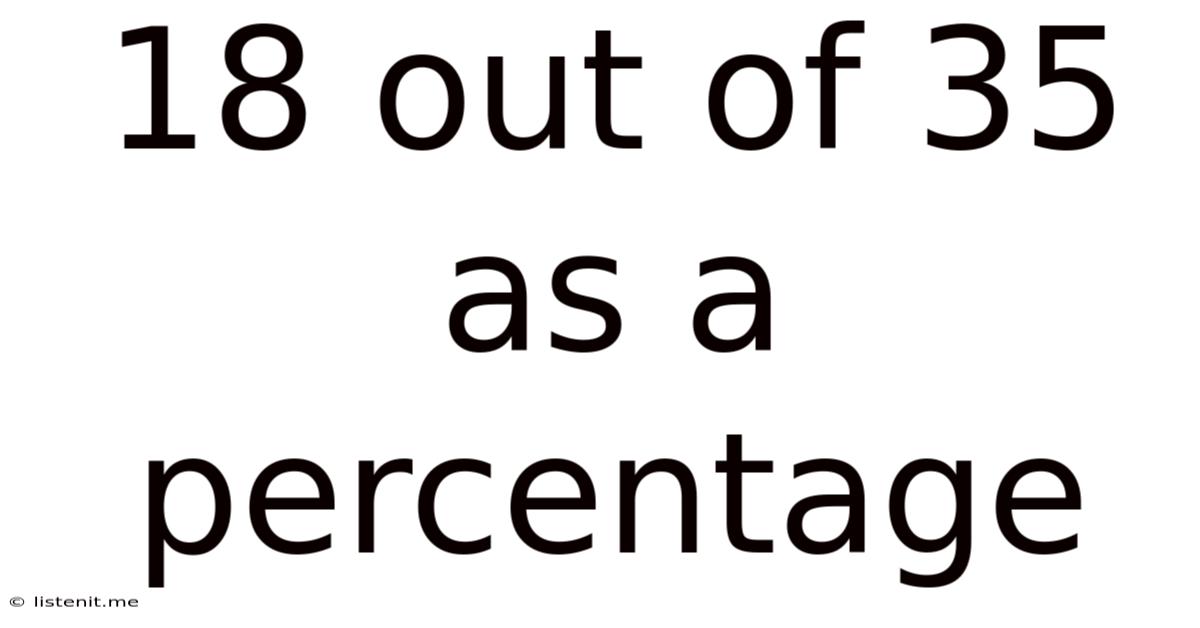
Table of Contents
18 out of 35 as a Percentage: A Comprehensive Guide
Calculating percentages is a fundamental skill with widespread applications in various aspects of life, from academic assessments and financial transactions to everyday decision-making. Understanding how to convert fractions into percentages is crucial for accurate interpretation of data and effective communication of numerical information. This comprehensive guide will delve into the calculation of "18 out of 35 as a percentage," providing not only the answer but also a detailed explanation of the process, variations, and practical applications.
Understanding Percentages
Before we jump into the specific calculation, let's establish a solid understanding of percentages. A percentage is a way of expressing a number as a fraction of 100. The term "percent" literally means "out of 100" (per centum). Therefore, 50% represents 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5 as a decimal. This fundamental concept forms the basis of all percentage calculations.
Calculating 18 out of 35 as a Percentage: The Step-by-Step Method
To calculate 18 out of 35 as a percentage, we follow a straightforward three-step process:
Step 1: Express the fraction:
The phrase "18 out of 35" can be directly expressed as a fraction: 18/35. This represents the ratio of 18 to 35.
Step 2: Convert the fraction to a decimal:
To convert the fraction 18/35 to a decimal, we divide the numerator (18) by the denominator (35):
18 ÷ 35 ≈ 0.5143
Step 3: Convert the decimal to a percentage:
To convert the decimal to a percentage, we multiply the decimal by 100 and add the "%" symbol:
0.5143 × 100 ≈ 51.43%
Therefore, 18 out of 35 is approximately 51.43%.
Alternative Methods and Rounding
While the above method is the most straightforward, there are alternative approaches, particularly useful when dealing with more complex fractions or when using a calculator.
Method 2: Using a Calculator:
Most calculators have a percentage function. You can directly input 18/35 and then press the percentage button (%) to obtain the result of 51.43%.
Method 3: Proportion Method:
This method involves setting up a proportion:
18/35 = x/100
To solve for x (the percentage), cross-multiply:
35x = 1800
x = 1800/35 ≈ 51.43
Rounding:
In the calculations above, we've rounded the percentage to two decimal places (51.43%). The level of rounding depends on the context. For instance, in a scientific context, more decimal places might be necessary, while in everyday situations, rounding to the nearest whole number (51%) might suffice.
Practical Applications of Percentage Calculations
Understanding percentage calculations has far-reaching implications across diverse fields:
1. Academic Performance:
- Grade Calculation: Many grading systems utilize percentages. If a student scores 18 out of 35 on a test, their score is 51.43%. This allows for easy comparison of performance across different tests with varying total marks.
- Exam Results: Understanding your percentage score in exams provides a clear indication of your overall performance and areas for improvement.
2. Financial Matters:
- Interest Rates: Interest rates are frequently expressed as percentages. For example, a 5% interest rate on a loan means that you pay 5% of the principal amount as interest.
- Discounts and Sales: Retailers often advertise discounts as percentages (e.g., 20% off). Calculating the actual discount requires percentage calculations.
- Tax Calculations: Taxes are often levied as a percentage of income or the value of goods and services.
3. Data Analysis and Statistics:
- Representing Proportions: Percentages are widely used to represent proportions within data sets, enabling clearer visual representation in charts and graphs.
- Surveys and Polls: Survey results are often expressed as percentages to summarize the opinions of a larger population.
4. Everyday Life:
- Tip Calculation: Calculating tips in restaurants frequently involves estimating a percentage (e.g., 15% or 20%) of the bill.
- Recipe Adjustments: Scaling recipes up or down requires adjusting ingredient quantities by a certain percentage.
Beyond the Basics: Advanced Percentage Calculations
While calculating "18 out of 35 as a percentage" is a relatively simple operation, mastering percentages also entails understanding more complex scenarios:
1. Percentage Increase and Decrease:
These calculations involve finding the percentage change between two values. For example, if a price increases from $100 to $120, the percentage increase is 20%. Conversely, a decrease from $120 to $100 is a 16.67% decrease.
2. Percentage of a Percentage:
This involves finding a percentage of a value that is already a percentage. For instance, finding 10% of 25% of 1000. This often occurs in situations involving compound interest or multi-stage discounts.
3. Working Backwards from Percentages:
This involves finding the original value when only a percentage and a resulting value are known. For example, if a product is on sale at 20% off and the sale price is $80, the original price can be calculated using percentage calculations.
Conclusion
Calculating "18 out of 35 as a percentage" (approximately 51.43%) is a foundational skill with widespread practical applications. This guide has provided a detailed explanation of the calculation process, along with alternative methods and a discussion of more advanced percentage calculations. By mastering these concepts, you'll enhance your ability to interpret data, make informed decisions, and confidently tackle various real-world problems that involve numerical representations. Remember to practice regularly to solidify your understanding and build proficiency in calculating percentages accurately and efficiently. Understanding percentages is not just about numbers; it's about understanding proportions and interpreting the world around us more effectively.
Latest Posts
Latest Posts
-
Find The Quotient Of A Fraction
May 26, 2025
-
What Is 20 Percent Of 50 Dollars
May 26, 2025
-
11 Out Of 40 As A Percentage
May 26, 2025
-
How Many Btus To Heat 600 Square Feet
May 26, 2025
-
12 4 5 X 15 4
May 26, 2025
Related Post
Thank you for visiting our website which covers about 18 Out Of 35 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.