17 As A Percentage Of 30
listenit
May 24, 2025 · 5 min read
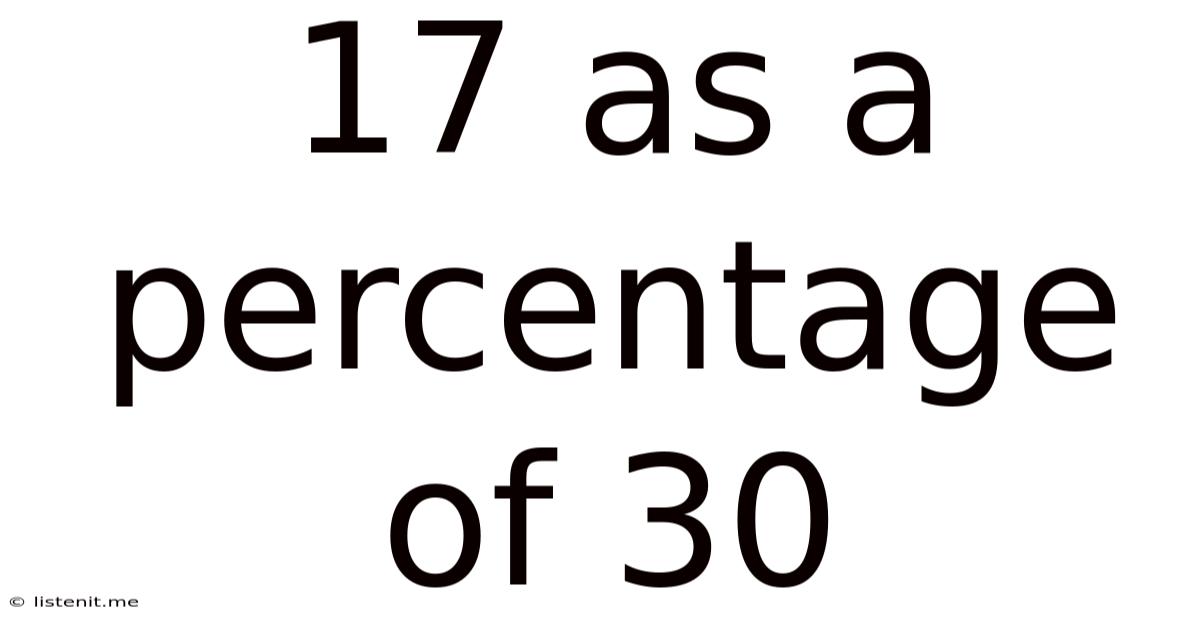
Table of Contents
17 as a Percentage of 30: A Comprehensive Guide to Percentage Calculations
Calculating percentages is a fundamental skill with applications spanning various fields, from finance and statistics to everyday life. Understanding how to determine one number as a percentage of another is crucial for making informed decisions and interpreting data effectively. This article delves into the calculation of 17 as a percentage of 30, providing a step-by-step guide, exploring different calculation methods, and offering practical applications and examples.
Understanding Percentages
A percentage is a fraction or ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." Percentages are a convenient way to express proportions and comparisons, making them easier to understand and interpret than fractions or decimals.
Calculating 17 as a Percentage of 30: The Basic Method
The most straightforward method to calculate 17 as a percentage of 30 involves three simple steps:
-
Divide the part by the whole: Divide the number you want to express as a percentage (17) by the total number (30). This gives you a decimal:
17 ÷ 30 = 0.5667 (approximately)
-
Multiply by 100: Multiply the decimal obtained in step 1 by 100 to convert it into a percentage.
0.5667 x 100 = 56.67%
-
Round off (if necessary): Round the percentage to the desired number of decimal places. In this case, rounding to two decimal places gives us 56.67%.
Therefore, 17 is 56.67% of 30.
Alternative Calculation Methods
While the basic method is efficient, several alternative methods can be used depending on your preference and the context of the problem.
Method 2: Using Proportions
This method leverages the concept of proportions to solve for the percentage. We can set up a proportion:
Let x be the percentage we want to find.
17/30 = x/100
To solve for x, we cross-multiply:
30x = 1700
x = 1700/30
x = 56.67%
This method demonstrates the underlying relationship between fractions and percentages.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the process. Simply enter 17 ÷ 30 and then multiply the result by 100. The calculator will automatically display the result as a percentage. This is particularly helpful for more complex calculations or when dealing with larger numbers.
Practical Applications and Examples
The ability to calculate percentages has wide-ranging applications across numerous fields. Here are some examples showcasing the practical use of this calculation:
1. Business and Finance
- Profit margins: Calculating the profit margin involves determining the percentage of profit relative to revenue. If a company made a profit of $17 million on a revenue of $30 million, their profit margin would be 56.67%.
- Sales growth: Tracking sales growth over time often involves calculating percentage change. If sales increased from $30 million to $47 million, the growth would be calculated as (47-30)/30 * 100 = 56.67%.
- Interest rates: Understanding interest rates requires calculating percentages. If you borrow $30,000 and pay $17,000 in interest, the interest rate would be calculated as a percentage of the principal amount.
- Discounts and Sales Tax: Calculating discounts and sales tax involves determining a percentage of the original price. For example, a 56.67% discount on a $30 item means the discount is $17.
2. Statistics and Data Analysis
- Data representation: Percentages are used extensively in data representation to illustrate proportions within a dataset. For instance, if 17 out of 30 survey respondents answered "yes" to a particular question, the percentage of "yes" responses would be 56.67%.
- Statistical significance: Percentages are used in hypothesis testing to determine the statistical significance of findings.
- Probability: Calculating probabilities often involves determining percentages.
3. Everyday Life
- Tip calculation: Calculating a tip in a restaurant is a common application of percentages. A 56.67% tip on a $30 bill would be $17.
- Grade calculation: In many grading systems, a student's final grade is expressed as a percentage of the total possible points.
- Recipe scaling: Adjusting recipes to serve more or fewer people requires calculating percentages of the original ingredient quantities.
Understanding the Significance of the Result (56.67%)
The result of 56.67% indicates that 17 represents a significant portion of 30. It's more than half, signifying a substantial proportion. Understanding this magnitude is crucial in interpreting the data and making informed decisions based on the information. In many contexts, a percentage above 50% represents a majority or a significant proportion.
Beyond the Calculation: Improving Your Percentage Skills
Mastering percentage calculations extends beyond simply performing the calculations. It also involves developing a strong understanding of the underlying concepts and developing problem-solving skills. Here are some tips to improve your skills:
- Practice Regularly: The more you practice, the more comfortable you'll become with these calculations. Work through various examples and problems to build your proficiency.
- Understand the Concepts: Don't just memorize the steps; understand the reasoning behind each step. This will help you approach different percentage problems more effectively.
- Use Different Methods: Try solving the same problem using different methods. This will reinforce your understanding and help you identify the most efficient method for different situations.
- Utilize Online Resources: Numerous online resources, including calculators and tutorials, can provide additional support and practice opportunities.
- Apply to Real-World Scenarios: Practice applying percentage calculations to real-world scenarios to make the concepts more relevant and engaging.
Conclusion
Calculating 17 as a percentage of 30, resulting in 56.67%, is a straightforward yet crucial skill. Understanding the various methods of calculation, their applications, and the significance of the result will equip you with a powerful tool for making informed decisions across various aspects of life and professional endeavors. By mastering percentage calculations and practicing regularly, you’ll enhance your ability to interpret data, make better judgments, and navigate a world increasingly reliant on numerical information. Remember to always practice and apply your knowledge in diverse contexts for a comprehensive understanding.
Latest Posts
Latest Posts
-
25 As A Power With Base 5
May 24, 2025
-
What Is 15 Rounded To The Nearest 10
May 24, 2025
-
How Long Will 1 5 Million Last In Retirement Calculator
May 24, 2025
-
6 4 5 As An Improper Fraction
May 24, 2025
-
18 Months In Years And Days
May 24, 2025
Related Post
Thank you for visiting our website which covers about 17 As A Percentage Of 30 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.