16.2 Is What Percent Of 45
listenit
May 24, 2025 · 4 min read
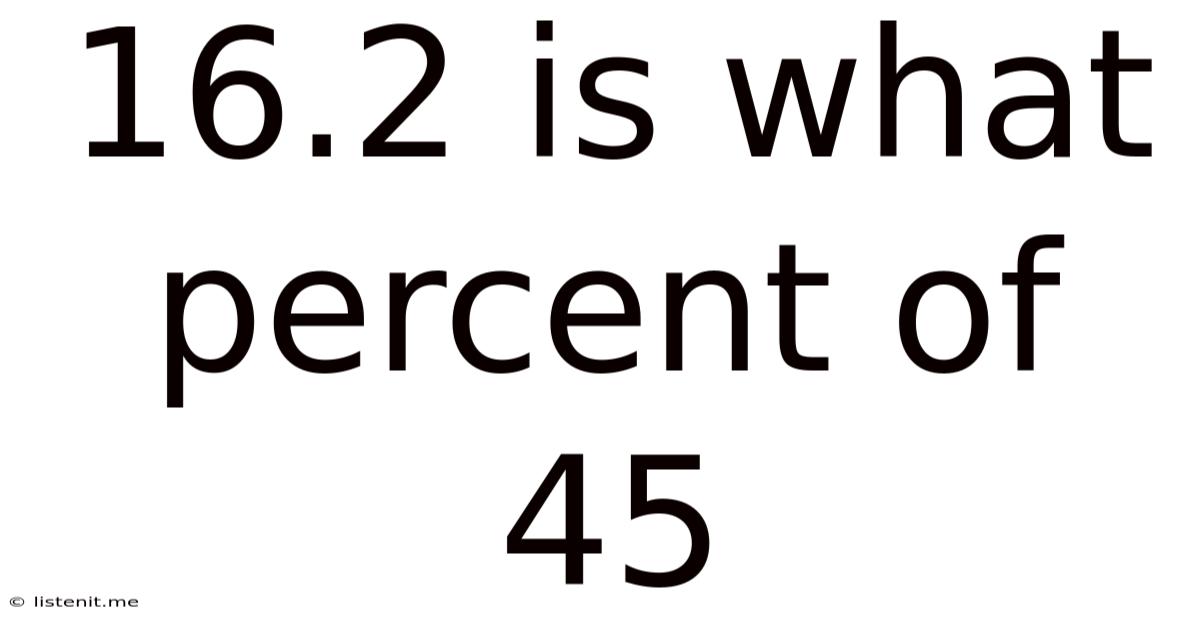
Table of Contents
16.2 is What Percent of 45? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous fields, from finance and statistics to everyday life. This comprehensive guide will not only answer the question, "16.2 is what percent of 45?" but also equip you with the knowledge and techniques to tackle similar percentage problems with confidence. We'll explore different methods, delve into the underlying concepts, and provide practical examples to solidify your understanding.
Understanding Percentages
Before we dive into the calculation, let's establish a solid foundation. A percentage is a way of expressing a number as a fraction of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For instance, 50% means 50 out of 100, or 50/100, which simplifies to 1/2 or 0.5.
Method 1: Using the Percentage Formula
The most direct way to determine what percentage 16.2 represents of 45 is to use the fundamental percentage formula:
(Part / Whole) x 100 = Percentage
In this case:
- Part: 16.2
- Whole: 45
Therefore, the calculation is:
(16.2 / 45) x 100 = ?
Let's break it down:
-
Divide the part by the whole: 16.2 / 45 = 0.36
-
Multiply the result by 100: 0.36 x 100 = 36
Therefore, 16.2 is 36% of 45.
Method 2: Using Proportions
Another effective approach involves setting up a proportion. A proportion is an equation stating that two ratios are equal. We can represent the problem as:
16.2 / 45 = x / 100
Where 'x' represents the unknown percentage. To solve for 'x', we cross-multiply:
16.2 * 100 = 45 * x
1620 = 45x
Now, divide both sides by 45:
x = 1620 / 45 = 36
Again, we find that 16.2 is 36% of 45.
Method 3: Working with Decimals
This method emphasizes the direct relationship between decimals and percentages. We can first express the part as a decimal fraction of the whole:
16.2 / 45 = 0.36
To convert this decimal to a percentage, simply multiply by 100:
0.36 x 100 = 36%
This reinforces the result: 16.2 is 36% of 45.
Practical Applications and Real-World Examples
Understanding percentage calculations is crucial in various real-world scenarios. Here are a few examples:
1. Sales and Discounts:
Imagine a store offering a discount on an item originally priced at $45. If the discount amounts to $16.20, you can use the percentage formula to determine the discount percentage: ($16.20 / $45) x 100 = 36%. The store is offering a 36% discount.
2. Grade Calculation:
If a student scores 16.2 out of 45 points on a quiz, their percentage score would be (16.2 / 45) x 100 = 36%.
3. Financial Analysis:
In finance, percentage calculations are essential for analyzing profit margins, returns on investment (ROI), and interest rates. For example, if an investment of $45 yields a profit of $16.20, the return on investment is 36%.
4. Survey Results:
Analyzing survey results often involves calculating percentages. If 16.2 out of 45 respondents answered "yes" to a particular question, the percentage of "yes" responses is 36%.
5. Data Analysis:
Percentage calculations are frequently used in various forms of data analysis to understand proportions and trends. They're integral to presenting data in a clear and easily understandable manner.
Advanced Percentage Problems and Techniques
While the problem "16.2 is what percent of 45?" is relatively straightforward, more complex percentage problems may arise. Here are some advanced techniques:
1. Finding the Whole When the Percentage and Part are Known:
Suppose you know that 36% of a number is 16.2. To find the whole number, you can use the formula:
Whole = (Part / Percentage) x 100
Whole = (16.2 / 36) x 100 = 45
2. Finding the Part When the Percentage and Whole are Known:
If you know that 36% of 45 is a certain number, you can find the part using:
Part = (Percentage / 100) x Whole
Part = (36 / 100) x 45 = 16.2
3. Percentage Increase or Decrease:
Calculating percentage change involves determining the increase or decrease in a value relative to the original value. The formula is:
Percentage Change = [(New Value - Old Value) / Old Value] x 100
For example, if a value increases from 45 to 60.8, the percentage increase is [(60.8 - 45) / 45] x 100 = 35.11%.
Troubleshooting Common Percentage Calculation Errors
Many errors in percentage calculations stem from misinterpreting the problem or incorrectly applying the formula. Here are some common mistakes to avoid:
- Incorrect identification of the part and the whole: Always carefully determine which value represents the part and which represents the whole.
- Improper order of operations: Ensure you follow the order of operations (PEMDAS/BODMAS) correctly – parentheses, exponents, multiplication and division (from left to right), addition and subtraction (from left to right).
- Decimal errors: Be mindful of decimal points when performing calculations.
- Misunderstanding percentage increase/decrease: Clearly understand whether you are calculating an increase or decrease and apply the correct formula accordingly.
Conclusion: Mastering Percentage Calculations
This in-depth exploration of percentage calculations has not only provided a definitive answer to "16.2 is what percent of 45?" (36%) but also equipped you with the tools and understanding to tackle a wide range of percentage problems. By mastering these techniques and avoiding common pitfalls, you'll be well-prepared to confidently handle percentage calculations in various academic, professional, and personal contexts. Remember to practice regularly to reinforce your understanding and build your proficiency. The more you practice, the more intuitive percentage calculations will become.
Latest Posts
Latest Posts
-
1989 How Old Would I Be
May 24, 2025
-
30 Days After May 10 2024
May 24, 2025
-
What Is 1 Divided By 7
May 24, 2025
-
What Is The Prime Factorization Of 86
May 24, 2025
-
1 Day 13 Hours From Now
May 24, 2025
Related Post
Thank you for visiting our website which covers about 16.2 Is What Percent Of 45 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.