120 Is What Percent Of 240
listenit
May 12, 2025 · 4 min read
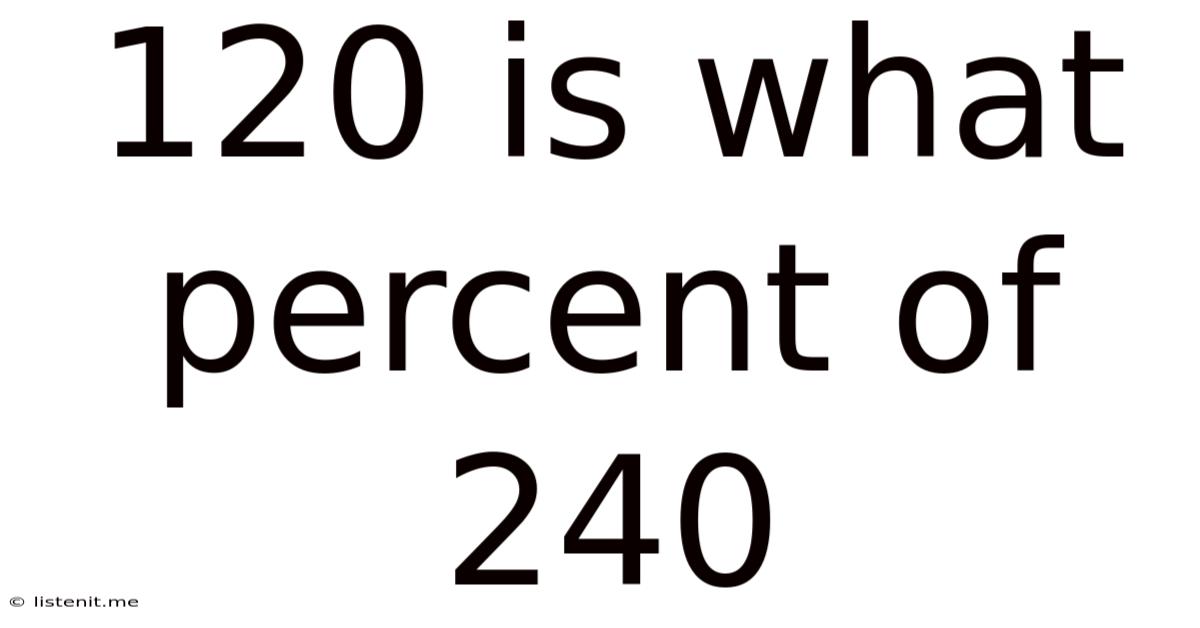
Table of Contents
120 is What Percent of 240? A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts and tax rates to analyzing data and making informed decisions. This comprehensive guide will not only answer the question "120 is what percent of 240?" but will also equip you with the knowledge and techniques to tackle similar percentage problems confidently and efficiently.
Understanding Percentages: The Basics
A percentage is a fraction or a ratio expressed as a number out of 100. The symbol "%" represents "per cent," meaning "out of one hundred." For example, 50% means 50 out of 100, which is equivalent to 1/2 or 0.5.
Percentages are used extensively because they provide a standardized way to compare and understand proportions. Whether you're dealing with financial matters, statistical analysis, or everyday situations, mastering percentage calculations is crucial.
Calculating Percentages: The Formula
The fundamental formula for calculating percentages is:
(Part / Whole) * 100 = Percentage
Where:
- Part: Represents the value you want to express as a percentage of the whole.
- Whole: Represents the total value or the base amount.
- Percentage: The result expressed as a percentage.
Let's break down this formula with an example: If you scored 80 out of 100 on a test, what is your percentage score?
In this case:
- Part = 80
- Whole = 100
Applying the formula:
(80 / 100) * 100 = 80%
Therefore, your test score is 80%.
Solving the Problem: 120 is What Percent of 240?
Now, let's apply this formula to answer the question posed in the title: "120 is what percent of 240?"
Here:
- Part = 120
- Whole = 240
Applying the formula:
(120 / 240) * 100 = 50%
Therefore, 120 is 50% of 240.
Different Approaches to Solving Percentage Problems
While the fundamental formula is always applicable, understanding alternative approaches can enhance your problem-solving skills and make calculations more efficient. Here are some common methods:
1. Using Proportions:
Setting up a proportion is a valuable technique, especially when dealing with more complex percentage problems. A proportion is a statement that two ratios are equal. We can represent the problem as:
120/240 = x/100
Solving for x:
x = (120 * 100) / 240 = 50
Therefore, x = 50%, confirming our previous result.
2. Using Decimal Conversion:
Converting the fraction to a decimal before multiplying by 100 can simplify the calculation:
120/240 = 0.5
0.5 * 100 = 50%
3. Mental Math Techniques:
For simpler problems, mental math can be a quick and efficient approach. Recognizing that 120 is half of 240 directly leads to the answer of 50%.
Real-World Applications of Percentage Calculations
Understanding percentages is crucial in numerous real-world scenarios:
1. Financial Matters:
- Calculating discounts: A 20% discount on a $100 item means a saving of $20 ($100 * 0.20).
- Determining interest rates: Understanding interest rates on loans, savings accounts, and investments is crucial for financial planning.
- Analyzing financial statements: Percentages are used extensively in analyzing financial reports and assessing profitability.
- Tax calculations: Sales tax, income tax, and other taxes are often expressed as percentages.
2. Data Analysis and Statistics:
- Representing proportions: Percentages help visualize and compare proportions within datasets.
- Calculating growth rates: Percentage change is frequently used to measure growth or decline in various metrics.
- Interpreting survey results: Survey results are often represented as percentages to show the distribution of responses.
3. Everyday Life:
- Calculating tips: Calculating tips in restaurants involves understanding percentages.
- Comparing prices: Determining which product offers better value often involves comparing prices and discounts expressed as percentages.
- Understanding nutritional information: Nutritional labels on food products often list percentages of daily recommended values for various nutrients.
Beyond the Basics: More Complex Percentage Problems
The techniques discussed above provide a solid foundation for solving a wide range of percentage problems. However, some problems might involve more complex calculations, such as:
- Finding the original amount: If 25% of a number is 50, what is the original number? (This involves working backward from the percentage.)
- Calculating percentage increase or decrease: This requires understanding the difference between the original and final values and expressing that difference as a percentage of the original value.
- Compound percentages: These involve applying percentages repeatedly, such as compound interest calculations.
Mastering these more advanced techniques requires practice and a deeper understanding of the underlying principles of percentage calculations.
Practice Problems: Test Your Understanding
To solidify your understanding, let's try some practice problems:
- What is 30% of 150?
- 60 is what percent of 120?
- If you receive a 15% discount on an item priced at $80, what is the final price?
- A store increases its prices by 10%. If the original price of an item was $50, what is the new price?
- If a company's profits increased from $100,000 to $125,000, what is the percentage increase in profit?
Solutions:
- 45
- 50%
- $68
- $55
- 25%
Conclusion: Mastering Percentages for Success
Percentages are an integral part of numerous aspects of life. By understanding the fundamental formula, exploring different calculation methods, and practicing regularly, you can confidently tackle various percentage problems. This comprehensive guide provides a solid foundation for mastering percentages, empowering you to make informed decisions in your personal and professional life. Remember to practice consistently to improve your speed and accuracy in solving percentage-related problems, ensuring you are well-equipped to handle the mathematical challenges that arise in everyday situations.
Latest Posts
Latest Posts
-
How Many Bones Does A Monkey Have
May 12, 2025
-
What Is The Electronic Configuration Of Gold
May 12, 2025
-
4 9 As A Decimal And Percent
May 12, 2025
-
A Mixture That Can Be Separated By Filtration
May 12, 2025
-
When The Frequency Increases The Waves Become
May 12, 2025
Related Post
Thank you for visiting our website which covers about 120 Is What Percent Of 240 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.