12 Times Square Root Of 2
listenit
May 11, 2025 · 5 min read
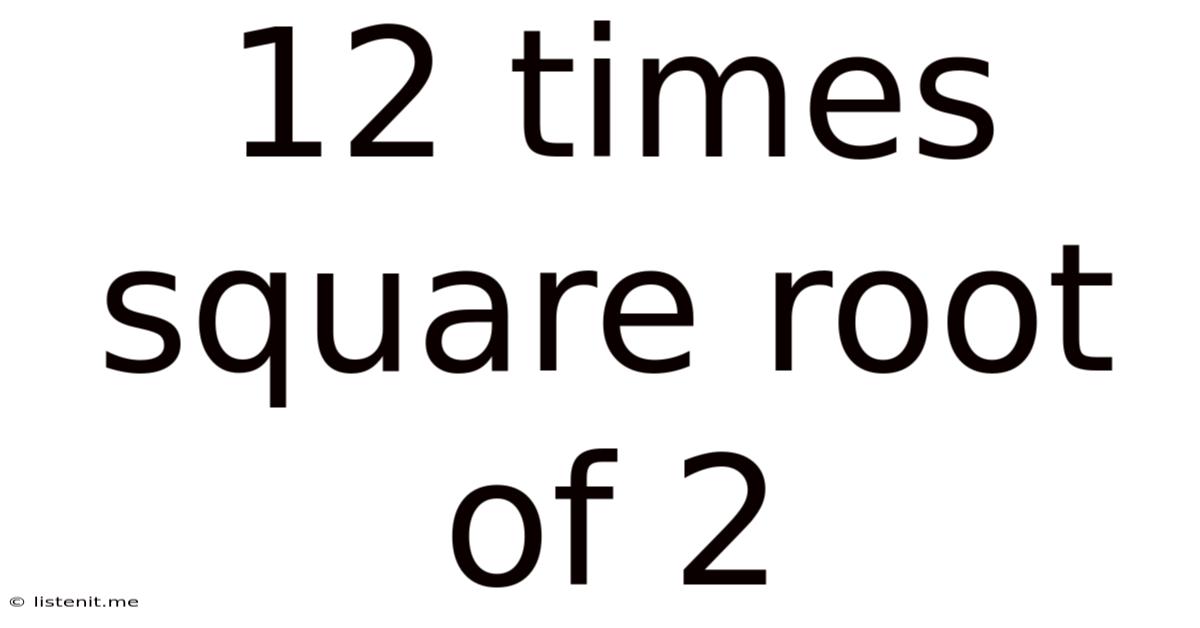
Table of Contents
12 Times the Square Root of 2: Unveiling the Mathematical Mystery
The seemingly simple expression "12√2" hides a surprising depth of mathematical significance and practical applications. While at first glance it might appear to be just a numerical value, a closer examination reveals its connections to geometry, trigonometry, and even the real world. This article will delve into the various aspects of 12√2, exploring its properties, calculations, and its role in diverse fields.
Understanding the Basics: What is 12√2?
Before we delve into the intricacies of 12√2, let's establish a firm understanding of its components. The number 12 is a familiar integer, easily grasped and understood. The square root of 2 (√2), however, represents a unique type of number: an irrational number. This means it cannot be expressed as a simple fraction (a ratio of two integers) and its decimal representation continues infinitely without repeating. Its approximate value is 1.41421356...
Therefore, 12√2 represents the product of 12 and the square root of 2. This results in an irrational number approximately equal to 16.97056. While we can use this approximation for practical calculations, it's crucial to remember that the true value is an infinitely long decimal.
Calculating 12√2: Methods and Approaches
Calculating the precise value of 12√2 is impossible due to the irrational nature of √2. However, we can obtain highly accurate approximations using several methods:
1. Using a Calculator:
The simplest method is to use a calculator with a square root function. Simply input "12 * √2" and the calculator will provide a decimal approximation. The accuracy depends on the calculator's capabilities.
2. Utilizing Long Multiplication and Approximation:
For a more hands-on approach, we can use a pre-calculated approximation of √2 (e.g., 1.4142) and perform long multiplication with 12. This method provides a good approximation, especially if using a sufficient number of decimal places for √2.
3. Employing Numerical Methods:
For even greater accuracy, numerical methods like the Babylonian method (or Heron's method) can be used to approximate √2 to a high degree of precision. These iterative methods refine the approximation with each step, converging towards the true value of √2. Once a highly precise value for √2 is obtained, it can be multiplied by 12.
Geometrical Significance: The 45-45-90 Triangle
The number 12√2 holds significant geometrical importance, particularly in relation to the 45-45-90 triangle, also known as an isosceles right-angled triangle.
In a 45-45-90 triangle, the two legs (the sides forming the right angle) are equal in length. If we let the length of each leg be 'x', then by the Pythagorean theorem (a² + b² = c²), the hypotenuse (the side opposite the right angle) can be calculated as:
x² + x² = c² 2x² = c² c = x√2
This demonstrates that the hypotenuse of a 45-45-90 triangle is always √2 times the length of one of its legs. Therefore, if the length of one leg is 12 units, the hypotenuse will be 12√2 units long. This relationship is widely used in various geometrical problems and constructions.
Applications in Real-World Scenarios
The value 12√2, and more generally the concept of √2, surfaces in a surprising number of real-world situations:
-
Construction and Engineering: Calculations involving diagonal measurements frequently involve √2. For instance, determining the length of a diagonal brace in a square frame or the distance across a square field. If a square room has sides of 12 feet, its diagonal would measure 12√2 feet.
-
Computer Graphics and Game Development: √2 plays a crucial role in calculating distances and rotations in two-dimensional space. This is fundamental in programming video games, creating 3D models, and rendering images.
-
Physics and Engineering: Many physical phenomena involve diagonal movements or forces. The calculation of resultant vectors, for example, might involve √2 in determining the magnitude of the combined force.
-
Navigation and Surveying: Determining distances and directions often necessitates using trigonometry, and √2 frequently arises in calculations involving right-angled triangles.
The Irrationality of 12√2: A Deeper Dive
The irrationality of √2 has been proven mathematically, and because 12 is a rational number (an integer), the product 12√2 remains irrational. This means its decimal representation is non-terminating and non-repeating. This property has implications for computational processes:
-
Precision Limitations: Computers can only store approximations of irrational numbers. This can introduce small errors in calculations that accumulate over time in complex computations.
-
Algorithmic Challenges: Working with irrational numbers requires careful consideration of precision and error handling in algorithms.
Extending the Concept: Beyond 12√2
The principles explored concerning 12√2 extend to other multiples of √2. The concept remains the same: a rational number multiplied by an irrational number always results in an irrational number. Understanding the behavior and properties of irrational numbers like √2 is essential in various mathematical and scientific fields.
Conclusion: The Enduring Relevance of 12√2
While seemingly a simple mathematical expression, 12√2 encapsulates a wealth of mathematical concepts and practical applications. Its connections to the 45-45-90 triangle, its role in geometric calculations, and its frequent appearance in real-world problems highlight its importance. The underlying irrationality of the number adds another layer of complexity and challenges to its precise computation. However, understanding this irrationality and employing suitable approximation methods are vital for navigating its various applications accurately and efficiently. 12√2, therefore, stands not merely as a numerical value but as a gateway to a deeper understanding of mathematics and its relevance to the world around us.
Latest Posts
Latest Posts
-
Why Do Electric Field Lines Never Cross
May 13, 2025
-
1 10 As A Percent And Decimal
May 13, 2025
-
Can All Minerals Be A Gemstone
May 13, 2025
-
Multicellular Heterotrophs Without A Cell Wall
May 13, 2025
-
What Are The Gcf Of 48
May 13, 2025
Related Post
Thank you for visiting our website which covers about 12 Times Square Root Of 2 . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.