10 Out Of 50 As A Percentage
listenit
May 25, 2025 · 5 min read
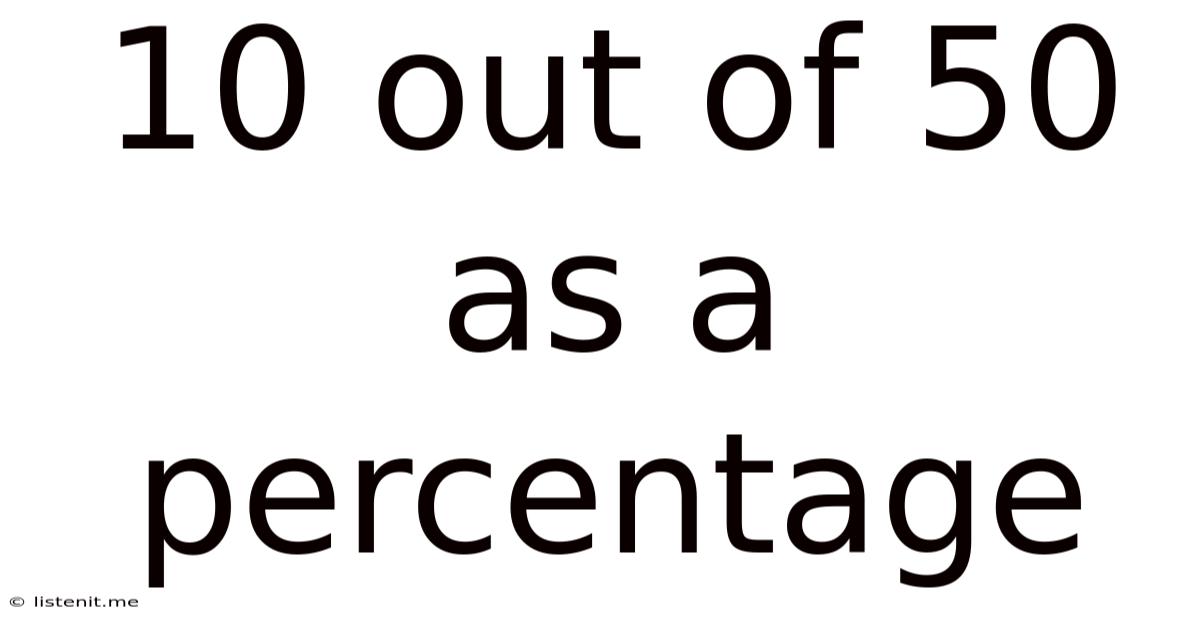
Table of Contents
10 Out of 50 as a Percentage: A Comprehensive Guide to Percentage Calculations
Understanding percentages is a fundamental skill in various aspects of life, from calculating discounts in a store to analyzing financial reports. This comprehensive guide will delve into the calculation of "10 out of 50 as a percentage," explaining the process step-by-step and expanding on related percentage concepts. We'll explore different methods, provide practical examples, and address common misconceptions to solidify your understanding.
Understanding the Basics: What is a Percentage?
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Percentages are denoted by the symbol "%". Essentially, a percentage represents a proportion or ratio out of a whole, where the whole is always considered to be 100.
Calculating 10 out of 50 as a Percentage: The Simple Method
The most straightforward way to calculate 10 out of 50 as a percentage is using a simple formula:
(Part / Whole) * 100%
In this case:
- Part: 10
- Whole: 50
Therefore, the calculation is:
(10 / 50) * 100% = 20%
Therefore, 10 out of 50 is equal to 20%.
Alternative Methods: Simplifying the Fraction
Before applying the formula, you can simplify the fraction 10/50. Both the numerator (10) and the denominator (50) are divisible by 10. Simplifying the fraction gives us 1/5. This simplification makes the calculation easier:
(1 / 5) * 100% = 20%
This method highlights the flexibility in percentage calculations. Simplifying the fraction first can often lead to simpler arithmetic.
Visual Representation: Understanding Proportions
Imagine you have a pie chart representing 50 slices. 10 of these slices are shaded. The shaded portion represents the proportion we want to express as a percentage. Clearly, 10 out of 50 is one-fifth (1/5) of the whole pie. This visual representation reinforces the concept that 10 out of 50 equates to 20%.
Practical Applications: Real-World Examples
Percentage calculations like this are used extensively in daily life and various professional fields. Here are a few examples:
- Test Scores: If you answered 10 questions correctly out of a total of 50 questions on a test, your score would be 20%.
- Sales and Discounts: A store offering a 20% discount on an item priced at $50 would reduce the price by $10 (20% of $50).
- Financial Analysis: In financial statements, percentages are used to represent ratios, proportions, and changes in various financial metrics. For example, a company's profit margin might be expressed as a percentage of revenue.
- Surveys and Polls: Survey results often present data as percentages. If 10 out of 50 respondents chose a particular option, that option received a 20% preference.
Beyond the Basics: Working with Different Fractions
Let's expand our understanding by looking at how to calculate percentages from other fractions. This will reinforce your understanding of the fundamental concepts and equip you to handle various percentage problems.
Example 1: 15 out of 50
Using the formula: (15 / 50) * 100% = 30%
Example 2: 25 out of 50
Using the formula: (25 / 50) * 100% = 50% (Note: 25/50 simplifies to 1/2, or 50%)
Example 3: 7 out of 50
Using the formula: (7 / 50) * 100% = 14%
Dealing with Decimals: Percentage Calculations with Non-Whole Numbers
Percentages are not limited to whole numbers. Let's consider scenarios involving decimal numbers:
Example 1: 12.5 out of 50
Using the formula: (12.5 / 50) * 100% = 25%
Example 2: 37.5 out of 50
Using the formula: (37.5 / 50) * 100% = 75%
These examples demonstrate the versatility of the percentage calculation formula. It accurately handles both whole numbers and decimal numbers, providing a consistent method for calculating percentages.
Common Mistakes to Avoid
While percentage calculations are relatively straightforward, some common mistakes can lead to inaccurate results. Let's address these to prevent errors:
- Incorrect Order of Operations: Always remember the order of operations (PEMDAS/BODMAS). In percentage calculations, division should be performed before multiplication.
- Misinterpreting the "Part" and "Whole": Carefully identify the "part" and the "whole" in the problem. The "part" is the portion you're expressing as a percentage of the "whole".
- Forgetting the "100%": Don't forget to multiply the fraction by 100% to express the result as a percentage.
Using Calculators and Spreadsheets: Efficient Calculation Tools
Calculators and spreadsheet software like Microsoft Excel or Google Sheets offer efficient ways to perform percentage calculations. These tools minimize manual calculation errors and save time, especially when dealing with numerous calculations. Most calculators have a percentage function (%) that simplifies the process significantly.
Advanced Percentage Applications: Percentage Increase and Decrease
Understanding percentages extends beyond basic calculations. Let's explore the concepts of percentage increase and decrease:
Percentage Increase
This represents the increase in a value expressed as a percentage of the original value.
Formula: [(New Value - Original Value) / Original Value] * 100%
Percentage Decrease
This represents the decrease in a value expressed as a percentage of the original value.
Formula: [(Original Value - New Value) / Original Value] * 100%
Conclusion: Mastering Percentage Calculations
Mastering percentage calculations is essential for navigating numerous real-world situations. Understanding the basic formula, different calculation methods, and common pitfalls will empower you to confidently tackle percentage problems across various contexts. Remember that consistent practice and a clear understanding of the fundamental principles are key to mastering this important mathematical skill. By applying these techniques and avoiding common errors, you'll build a strong foundation in percentage calculations, enabling you to accurately interpret and utilize percentage data in various applications.
Latest Posts
Latest Posts
-
What Is 40 Off Of 80 Dollars
May 25, 2025
-
Calculating Cap Rate For Rental Property
May 25, 2025
-
9 As A Fraction In Simplest Form
May 25, 2025
-
What Is The Greatest Common Factor Of 17 And 51
May 25, 2025
-
40 Mph To Meters Per Second
May 25, 2025
Related Post
Thank you for visiting our website which covers about 10 Out Of 50 As A Percentage . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.