1 Of 15 Is What Percent
listenit
May 24, 2025 · 5 min read
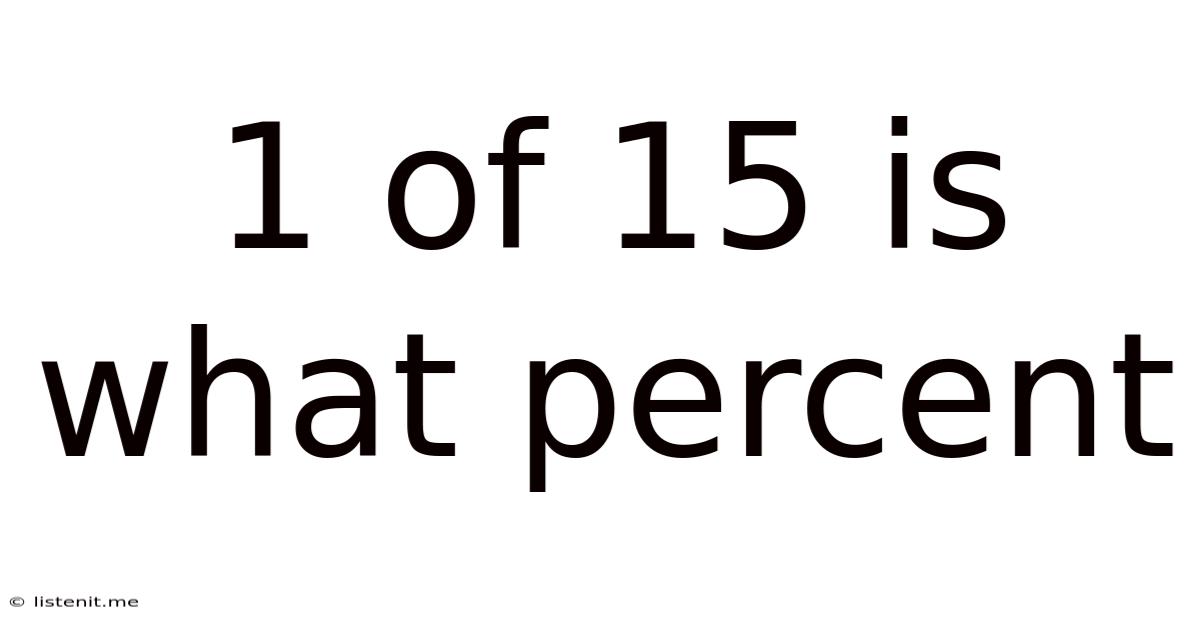
Table of Contents
1 out of 15: What Percent is That? A Deep Dive into Percentage Calculations
Understanding percentages is a fundamental skill applicable across numerous aspects of life, from calculating discounts and taxes to comprehending statistics and data analysis. This comprehensive guide will not only answer the question, "1 out of 15 is what percent?", but also equip you with the knowledge and methods to solve similar percentage problems with confidence. We'll explore various calculation methods, delve into the practical applications of percentage calculations, and offer tips and tricks for mastering this essential mathematical concept.
Understanding Percentages: The Basics
A percentage is a way of expressing a number as a fraction of 100. The word "percent" itself comes from the Latin "per centum," meaning "out of a hundred." Therefore, 10% means 10 out of 100, or 10/100, which simplifies to 1/10.
Understanding this fundamental principle is crucial for solving percentage problems. To calculate a percentage, we determine the ratio of the part to the whole, then multiply that ratio by 100.
Calculating "1 out of 15" as a Percentage: Three Methods
There are several ways to calculate what percentage 1 out of 15 represents. Let's explore three common methods:
Method 1: The Fraction Method
This method is straightforward and relies on converting the given ratio into a fraction and then into a percentage.
-
Express as a Fraction: "1 out of 15" can be written as the fraction 1/15.
-
Convert to Decimal: Divide the numerator (1) by the denominator (15): 1 ÷ 15 = 0.066666... (This is a recurring decimal).
-
Convert to Percentage: Multiply the decimal by 100: 0.066666... × 100 ≈ 6.67%.
Therefore, 1 out of 15 is approximately 6.67%. The recurring decimal necessitates rounding, typically to two decimal places for practical purposes.
Method 2: The Proportion Method
This method utilizes the concept of proportions to solve for the unknown percentage.
-
Set up a Proportion: We can set up a proportion as follows:
1/15 = x/100
Where 'x' represents the percentage we want to find.
-
Cross-Multiply: Cross-multiply the proportion: 15x = 100
-
Solve for x: Divide both sides by 15: x = 100/15 ≈ 6.67%
Again, we arrive at the same approximate answer: 6.67%.
Method 3: Using a Calculator
Most calculators have a percentage function that simplifies the calculation. Simply divide 1 by 15 and then multiply by 100. This method provides the same result, offering speed and convenience.
Practical Applications: Where Percentage Calculations Matter
Understanding percentage calculations is vital in numerous real-world scenarios. Here are some examples:
1. Business and Finance:
- Profit Margins: Calculating profit margins involves determining the percentage of revenue that represents profit.
- Discounts and Sales: Understanding discounts helps consumers determine the actual price after a percentage reduction.
- Interest Rates: Interest rates are expressed as percentages, crucial for understanding loan repayments and investment returns.
- Tax Calculations: Sales tax, income tax, and other taxes are often calculated as percentages of the total amount.
- Financial Statements: Analyzing financial statements requires interpreting data presented as percentages (e.g., debt-to-equity ratio).
2. Education and Statistics:
- Grade Calculation: Grades are often expressed as percentages, reflecting the proportion of correctly answered questions or assignments completed.
- Data Analysis: Statistical analysis often involves interpreting data presented as percentages, such as the percentage of respondents choosing a specific option in a survey.
- Test Scores: Standardized test scores are frequently converted into percentiles, indicating the student's ranking relative to others.
3. Everyday Life:
- Tipping: Calculating a tip in a restaurant usually involves determining a percentage of the total bill.
- Recipe Scaling: Adjusting ingredient quantities in recipes often involves scaling them up or down by a certain percentage.
- Sales Tax: Calculating the total price of an item including sales tax requires understanding percentage increases.
- Savings and Investments: Tracking savings and investment growth involves calculating percentage increases or decreases over time.
Beyond the Basics: More Complex Percentage Problems
While "1 out of 15 is what percent?" is a relatively simple problem, the principles extend to more complex scenarios. Consider these variations:
Finding the Whole when given a Percentage and Part
If you know that 10% of a quantity is 3, you can find the total quantity using a proportion:
10/100 = 3/x
Solving for x gives you the total quantity (30).
Finding the Part when given a Percentage and the Whole
If you know that a product costs $100 and is discounted by 20%, you can find the discount amount:
20/100 = x/100
Solving for x gives you the discount amount ($20).
Percentage Increase and Decrease
Calculating percentage increases and decreases involves comparing two values and expressing the difference as a percentage of the original value. For example, if a quantity increases from 50 to 60, the percentage increase is [(60-50)/50] × 100 = 20%.
Tips and Tricks for Mastering Percentage Calculations
- Practice Regularly: The key to mastering percentage calculations is consistent practice.
- Use Visual Aids: Diagrams and charts can help visualize percentages and make calculations easier to understand.
- Break Down Complex Problems: Decompose complex problems into smaller, more manageable steps.
- Utilize Online Calculators: Online calculators can verify your calculations and assist with complex problems.
- Understand the Context: Always consider the context of the problem to ensure you're interpreting the results correctly.
Conclusion: Mastering the Power of Percentages
The seemingly simple question, "1 out of 15 is what percent?", opens the door to a vast world of percentage calculations that are essential for navigating various aspects of daily life and professional endeavors. By understanding the different methods, applications, and variations discussed in this guide, you’ll equip yourself with the skills to confidently tackle percentage problems of all levels of complexity. Remember that consistent practice is key to mastering this essential mathematical concept. With dedication and practice, you’ll become proficient in calculating percentages and harness their power for success in all your pursuits.
Latest Posts
Latest Posts
-
5 10 At 15 How Tall Will I Be
May 25, 2025
-
When Will The Dew Dry Today
May 25, 2025
-
How Many Permutations Of 3 Numbers
May 25, 2025
-
What Is 3 Divided By 21
May 25, 2025
-
Highest Common Factor Of 21 And 28
May 25, 2025
Related Post
Thank you for visiting our website which covers about 1 Of 15 Is What Percent . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.